Two for one in a colloidal glass
It is often said that glass is a frozen liquid. In a glass, the molecules are disordered like a liquid, but macroscopically, the sample no longer flows, behaving like a solid. While we can describe what a glass is, we still don’t fully understand the transition to the glassy state [1]. One way to learn about the glass transition is to study model systems. Perhaps the simplest experimental model system is a colloidal glass. Colloidal particles are small solid particles, suspended in a liquid and capable of rearranging via Brownian motion. The key control parameter is the volume fraction ϕ, the fraction of space occupied by the particles. When the volume fraction is sufficiently high, particles collide with their neighbors frequently and find it impossible to rearrange, a state called a colloidal glass. One advantage of using colloidal particles as a model system for all glasses is that they are large enough to be viewed directly with microscopy, so we can develop a microscopic picture of how particles rearrange at the glass transition [2,3].
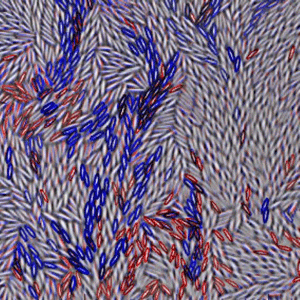
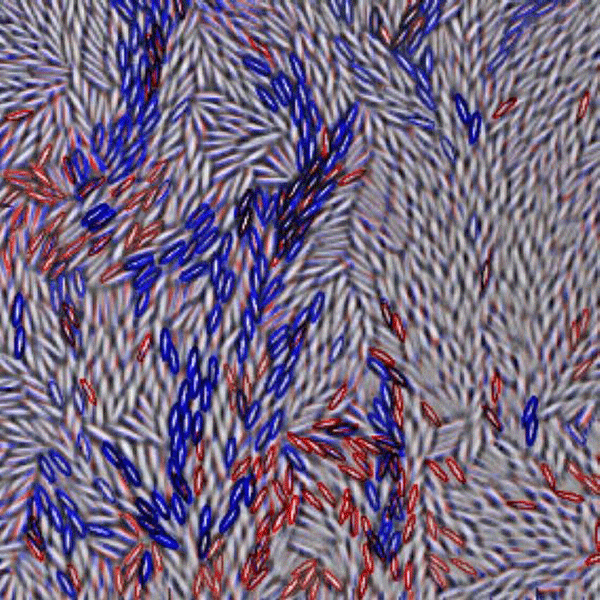
A drawback of using colloidal particles is that they are almost always spherical, whereas molecules usually have nontrivial shapes and directional interactions. It could be argued that for a model system, colloidal spheres miss some important physics. An experiment presented in Physical Review Letters from Zhongyu Zheng and his colleagues at the Hong Kong University of Science and Technology dramatically improves upon previous colloidal experiments by using colloidal ellipsoids with an aspect ratio of six [4]. To keep their experiment simple, they confine their samples between parallel glass plates so that the particle motions are limited to two dimensions. They then use video microscopy to follow the motion of several thousand ellipsoids, a small portion of which is shown in Fig. 1. By increasing the area fraction ϕ (the two dimensional counterpart to the volume fraction) occupied by the particles, they can induce glassy behavior. Strikingly, the sample undergoes not one, but two distinct glass transitions: one where the rotational motion drastically slows down, and a second corresponding to a slow down in translational motion.
The two glass transitions that Zheng et al. observe occur at different area fractions. Samples with ϕ<0.72 are liquids, such as the sample shown in Fig. 1 ( ϕ=0.70). In these samples, particles can rotate and diffuse throughout the sample, although this motion is quite slow in samples with densities approaching ϕ=0.72. Rotational motion becomes nearly impossible for 0.72<ϕ<0.79, while particles are still able to diffuse translationally. This signals a rotational glass transition, but translationally, the colloid is still a liquid. For ϕ>0.79, both translational and rotational degrees of freedom are glassy. These two distinct glass transitions were predicted by theory in 1997 [5], but Zheng et al.’s work is the first experimental confirmation.
As Fig. 1 shows, even at moderate area fractions the ellipsoids have small domains where the particles are aligned, similar to a liquid crystal in the nematic phase. Between these domains, the ellipsoids are disordered. Brownian motion enables the ellipsoids to either rotate or translate (most easily along their long axis). At higher area fractions, diffusion becomes difficult because particles become more and more constrained by their neighbors. In order for particles to rearrange, they must move in groups. Figure 1 shows a snapshot of this behavior. At this instant, the particles outlined in blue are the ones undergoing a large translational displacement, the red ones are rotating the most, and the few black particles are both translating and rotating by a significant amount. Similar behavior, where particles rearrange in groups, has been seen near the glass transition before in both simulations [6] and experiments on colloids [2,3], but these previous observations focused on spherical particles. In both the cases with spheres and Zheng et al.’s experiment with ellipsoids, the size of such regions grows sharply as the glass transition is approached. However, the frequency with which these rearrangements occur decreases just as sharply. Given that such microscopic rearrangements occur less often, this suggests a possible explanation for the macroscopic viscosity increase, which occurs in a glass, although the link between a microscopic picture and macroscopic measurements is not yet completely clear [1].
There are a number of obvious questions to address next. First, simulations and theory predict that the volume fraction where the glass transition occurs has a nonmonotonic dependence on aspect ratio [7–9]. The distinction between the two glass transitions should disappear for aspect ratios closer to one [5]. Such more spherical colloidal particles may be more relevant for understanding glass transitions of molecular glasses, which do not have two distinct glass transitions. Second, recent studies of how particles pack together show that even subtle shape differences matter. Convex shapes such as ellipsoids pack slightly better than concave shapes such as dumbbells, even when they have the same aspect ratio [10]. It is certainly possible that these details would influence the glass transition. Third, none of the shapes studied so far are as complex as molecules; there’s plenty of room to explore more molecularly realistic colloidal particles [11].
A reasonable question one might ask about the experimental work is how the colloidal glass differs from molecular glasses. Molecules do not undergo Brownian motion but, rather, move ballistically. Colloidal particles have hydrodynamic interactions whereby the motion of one particle influences the motion of neighboring particles; such interactions are absent in a molecular glass. Zheng et al.’s experiment is quasi-two-dimensional, while actual molecular glasses are three dimensional. If one wishes to use colloidal glasses as models for understanding molecular glasses, then hopefully these differences are unimportant. Prior studies of spherical particles show strong similarities between simulation and experiment [2,3,6]. Many simulations have studied both two-dimensional and three-dimensional glass transitions, and the dimensionality does not seem to be a significant distinction. Zheng et al.’s experiment is yet another piece of evidence that, indeed, the differences between colloidal samples and other glasses are not crucial. In this case, the simple colloidal experiment agrees well with simulations [7,9] and theory [5,8]. In fact, one of the most striking things about the experiment is that such a simple system—ellipsoids confined to two dimensions—is able to exhibit such interesting behavior. Given recent advances in making colloidal particles with various shapes [11], more interesting colloidal glass experiments from many groups are likely in the near future.
References
- L. Berthier and G. Biroli, Rev. Mod. Phys. 83, 587 (2011)
- W. K. Kegel and A. van Blaaderen, Science 287, 290 (2000)
- E. R. Weeks, J. C. Crocker, A. C. Levitt, A. Schofield, and D. A. Weitz, Science 287, 627 (2000)
- Z. Zheng, F. Wang, and Y. Han, Phys. Rev. Lett. 107, 065702 (2011)
- R. Schilling and T. Scheidsteger, Phys. Rev. E 56, 2932 (1997)
- C. Donati, J. F. Douglas, W. Kob, S. J. Plimpton, P. H. Poole, and S. C. Glotzer, Phys. Rev. Lett. 80, 2338 (1998)
- M. Letz, R. Schilling, and A. Latz, Phys. Rev. E 62, 5173 (2000)
- G. Yatsenko and K. S. Schweizer, Phys. Rev. E 76, 041506 (2007)
- C. De Michele, R. Schilling, and F. Sciortino, Phys. Rev. Lett. 98, 265702 (2007)
- C. F. Schreck, N. Xu, and C. S. O’Hern, Soft Matter 6, 2960 (2010)
- S. C. Glotzer and M. J. Solomon, Nature Mater. 6, 557 (2007)