Nuclear Spins Tango to the Tune of an Electron

Xuedong Hu

Viewpoint

Nuclear Spins Tango to the Tune of an Electron

Xuedong Hu

Department of Physics, University at Buffalo, State University of New York, Buffalo, NY 14260-1500, USA

October 17, 2011• Physics 4, 82

The anisotropic hyperfine interaction allows entanglement of two nuclear spins using microwave pulses.

Figure caption — **Figure 1:** (a) The magnetic dipole field produced by an electron spin. Note that the magnetic field experienced by the nuclear spin has a transverse component along the $x$ -axis, even though the electron spin is aligned with the external field along the $z$ -axis. Therefore, flipping the electron spin would lead to changes in both the magnitude and the direction of the magnetic field experienced by the nuclear spin. This is the essence of the anisotropic hyperfine interaction (AHF). (b) A malonic acid molecule. The red spheres are oxygen nuclei, the larger dark gray spheres are carbon nuclei, and the smaller light gray spheres are protons. One proton has been knocked off by x-ray irradiation, to leave a radical electron localized at the central carbon-13 nucleus. AHF is used in Ref. [7] to couple the nuclear spins of the carbon-13 and the proton via the electron spin.

Many nuclei have a magnetic moment that arises from their intrinsic spin. In an external magnetic field, these nuclei tend to align their moment with the field, just as a compass points to the North Pole in the Earth’s magnetic field. In many material systems, the nuclear spins are extremely well isolated from their environments, so that the dynamics of an individual nuclear spin is completely quantum coherent and governed by the Schrödinger equation of nonrelativistic quantum mechanics [1]. This high degree of quantum coherence makes some of the nuclear spins enticing candidates for bits for a quantum-mechanical computer [2]. However, the fact that these nuclear spins enjoy a quantum coherent “quiet life” also means that they are hard to access with external means, making them difficult to manipulate rapidly.

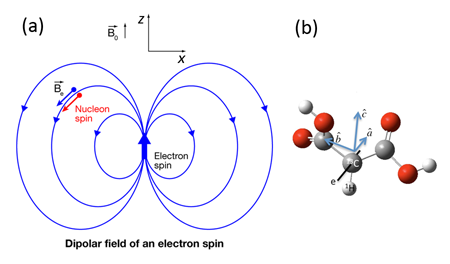
One of the established means to initialize, manipulate, and readout individual nuclear spins in a nanostructure is via an electron spin [2]. If an electron has a finite probability to be right on top of a nuclear spin, they interact through the so-called contact hyperfine interaction [1]. Otherwise they would still interact with each other through magnetic dipolar interaction, which decays as an inverse cubic function of the electron-nucleus distance $\sim 1 / r^{3}$ , and is, in general, anisotropic (thus the name anisotropic hyperfine interaction), as shown in Fig.1(a). Electron spins are much easier and faster to control than nuclear spins because they interact strongly with both electric and magnetic fields, and their energies are in the microwave frequency regime. Indeed, in recent years there has been tremendous experimental progress in controlling and reading out single electron-spin states in a variety of systems, such as $GaAs$ double dots [3,4], nitrogen-vacancy (NV) centers in diamond [5], and phosphorus ( $P$ ) donors [6]. Therefore, controlling nuclear spins via their hyperfine interaction with electron spins is becoming experimentally feasible.

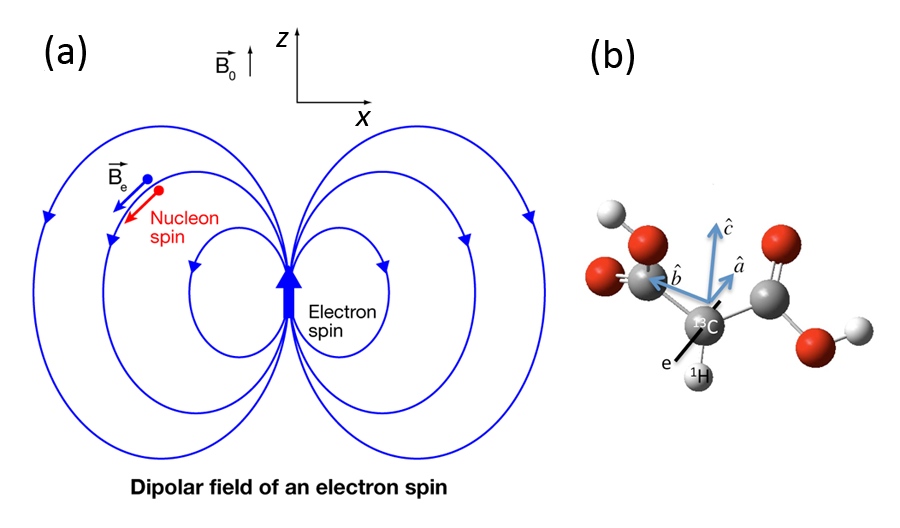
In a paper appearing in Physical Review Letters, Yingjie Zhang and colleagues from the University of Waterloo, Canada, have demonstrated how two nuclear spins can be coupled by an electron via anisotropic hyperfine interaction in a solid-state environment [7]. The material system studied is an x-ray-irradiated crystal of malonic acid molecules, in which a radical electron is hyperfine coupled to a $^{13} C$ nucleus and a proton, as shown in Fig.1(b). By applying various optimized electron-spin resonance (ESR) pulses in the microwave frequency range, Zhang et al. were able to create quantum coherence between the two nuclear spins, starting from an initial nuclear-spin state that is completely thermal and uncorrelated. The key for this experiment is the existence of the anisotropic component of the hyperfine interaction (AHF), which drives a nuclear spin to precess differently, depending on the electron-spin orientation, therefore allowing conditional operations on the nuclear spin. When such operations are applied to both nuclear spins, they acquire quantum-mechanical correlations between themselves from the common electron spin. In other words, the two nuclear spins can now get into lockstep in their precession—even though they do not talk to each other directly—because of the “directions” they receive from the ESR pulses via the common electron.

The current experiment points to a new way to enable nuclear-spin qubits in nanostructured systems, going beyond the complete reliance on the contact hyperfine interaction in past nuclear-spin qubit proposals [2]. Indeed, the presence of AHF allows complete control in the three-spin system, making malonic acid crystal at low temperature an interesting test bed of nuclear-spin qubit manipulations in a solid-state environment. In addition, with AHF one uses only microwave pulses (instead of both microwave and radio-frequency pulses) to control the nuclear spins, which speeds up the nuclear-spin manipulations significantly. In the current room-temperature experiment, nuclear-spin entanglement cannot be measured because they are pushed only slightly away from their initial thermal state. However, if the experiment is done at low temperature, the same experimental procedure would have led to nuclear-spin entanglement, which is the hallmark of quantum-mechanical correlation. Measurement of such correlation can be done using the microwave toolkit developed in the current experiment as well.

The future of the technique described in this paper seems bright. While malonic acid molecules may only be used to demonstrate the principle of nuclear-spin control and coupling, the same type of interactions are present in other more scalable systems. Among the most promising qubit candidates are the phosphorus donor nuclear spins in silicon ( $Si$ ) [2] and NV centers in diamond [5]. The former takes advantage of the strong contact hyperfine interaction between the phosphorus nuclear spin and the localized donor electron spin, and is set in silicon, the dominant semiconductor of today. The latter uses the spin states of a strongly localized defect in diamond, has extremely good coherence properties even at room temperature, and can be manipulated optically. Like malonic acid molecules, both these spin qubit systems have hyperfine interactions that are strongly anisotropic, which has been clearly revealed in spin-echo experiments [8,9]. Furthermore, an experiment has already been done to perform error correction encoding between an NV-center electron spin and an environmental $^{13} C$ nuclear spin, and to couple two nuclear spins through the NV-center electron, helped by both microwave and radio-frequency pulses and optical readout [10]. Using the technique developed in the current paper, these and other experiments in $Si:P$ and NV centers can potentially be done with less complexity, more efficiency, and faster speed, giving these materials systems a distinct advantage in terms of maneuverability.

From a broader perspective, AHF is already being used in biology and chemistry for the purpose of single nucleus recognition [1]. An all-microwave approach that can controllably couple multiple nuclear spins would enhance the toolkit of all the electron- and nuclear-spin spectroscopists. It is not clear whether such a new tool could be useful to applications such as magnetic resonance imaging, but that should hardly be an impediment to further explorations.

References

A. Schweiger and G. Jeschke, Principles of Pulse Electron Paramagnetic Resonance (Oxford University Press, New York, 2001)[Amazon][WorldCat]
B. E. Kane, Nature 393, 133 (1998)
J. R. Petta, A. C. Johnson, J. M. Taylor, E. A. Laird, A. Yacoby, M. D. Lukin, C. M. Marcus, M. P. Hanson, and A. C. Gossard, Science 309, 2180 (2005)
M. Pioro-Ladriere, T. Obata, Y. Tokura, Y. S. Shin, T. Kubo, K. Yoshida, T. Taniyama, and S. Tarucha, Nature Phys. 4, 776 (2008)
R. Hanson and D. D. Awschalom, Nature 453, 1043 (2008)
A. Morello, J. J. Pla, F. A. Zwanenburg, K. W. Chan, K. Y. Tan, H. Huebl, M. Mottonen, C. D. Nugroho, C. Y, Yang, J. A. van Donkelaar, A. D. C. Alves, D. N. Jamieson, C. C. Escott, L. C. L. Hollenberg, R. G. Clark, and A. S. Dzurak, Nature 467, 687 (2010)
Y. Zhang, C. A. Ryan, R. Laflamme, and J. Baugh, Phys. Rev. Lett. 107, 170503 (2011)
W. M. Witzel, X. Hu, and S. Das Sarma, Phys. Rev. B 76, 035212 (2007); E. Abe, A. M. Tyryshkin, S. Tojo, J. J. L. Morton, W. M. Witzel, A. Fujimoto, J. W. Ager, E. E. Haller, J. Isoya, S. A. Lyon, M. L. W. Thewalt, and K. M. Itoh, Phys. Rev. B 82, 121201R (2010)
L. Childress, M. V. Gurudev Dutt, J. M. Taylor, A. S. Zibrov, F. Jelezko, J. Wrachtrup, P. R. Hemmer, and M. D. Lukin, Science 314, 281 (2006)
L. Jiang, J. S. Hodges, J. R. Maze, P. Maurer, J. M. Taylor, D. G. Cory, P. R. Hemmer, R. L. Walsworth, A. Yacoby, A. S. Zibrov, and M. D. Lukin, Science 326, 267 (2009)

About the Author

Xuedong Hu is an associate professor of physics at the University at Buffalo, The State University of New York. He received his doctoral degree from the University of Michigan in 1996 under the supervision of Franco Nori, studying squeezed phonons. His postdoctoral work was on coherent control in semiconductor quantum wells and spin-based quantum computing in semiconductor nanostructures. He joined the University of Buffalo faculty in 2002 and became an associate professor in 2007. His current research interests are on quantum coherence and control in semiconductor and superconductor nanostructures, especially on problems related to solid-state quantum computing.