Chiral Quantum Textures
Chiral materials, in which the mirror reflection symmetry is broken, are ubiquitous in nature. Even the quantum vacuum of the standard model of particle physics is chiral [1], so that the behavior of the left-handed and right-handed elementary particles (quarks and leptons) are essentially different. One of the consequences is the chiral anomaly—the anomalous nonconservation of a chiral current, as first described by Adler and by Bell and Jackiw [2]. The chiral anomaly provides one explanation for the baryogenesis in the early Universe and the huge excess of matter over antimatter in the present Universe. A typical example of chiral materials is a cholestric liquid crystal, which consists of chiral molecules—objects that are not identical to their mirror image. Similarly, there are chiral magnets whose chirality is caused by the handedness in the arrangement of atoms.
In superfluid helium- 3, which often is referred to as the hydrogen atom of condensed matter [3], chirality appears as a more complex phenomenon. Liquid helium- 3 is a homogeneous system of spherical atoms, whose arrangement does not possess any handedness. Nevertheless, an ensemble of helium- 3 atoms in the anisotropic superfluid A phase is a macroscopically coherent quantum state, which, similar to the quantum vacuum, experiences broken mirror symmetry and also broken time-reversal symmetry. Orbital order parameter states, or textures, of different chirality and of large mm size have been expected to exist in this quantum liquid but have not been reliably experimentally identified. Now, Paul Walmsley and Andrei Golov from the University of Manchester, UK, report in Physical Review Letters that their torsional oscillator measurements distinguish between states of given chirality in a cylindrical disk-shaped volume of 10mm diameter [4]. In principle, a similar situation of chiral textures is also expected in nonconventional superconductors like Sr2RuO4 [5], but owing to defects and nonideal crystalline structure, experimental identification of extended orbital textures of different chirality are difficult to form and to identify.
In coherent systems with chirality, physical properties can be exotic. For example, in the ground state of a chiral superfluid there is a spontaneous superfluid current along the boundary. For chiral superconductors this means a persistent ground-state electric current along the surface without any resistance. Such a surface supercurrent transfers electric charge only in one direction, which is determined by the chirality. This is similar to the quantum Hall effect but occurs without an external magnetic field. In the Manchester torsional oscillator measurements, the direction of this surface current has now been determined. Another example of the unusual consequences from chirality is the intrinsic Magnus force, a lift force which acts on an object moving in a chiral superfluid. Thus an electron bubble drifting under an electric field in the chiral superfluid will be deflected differently, depending on the direction of windedness of the orbital texture, even in the absence of any magnetic field.
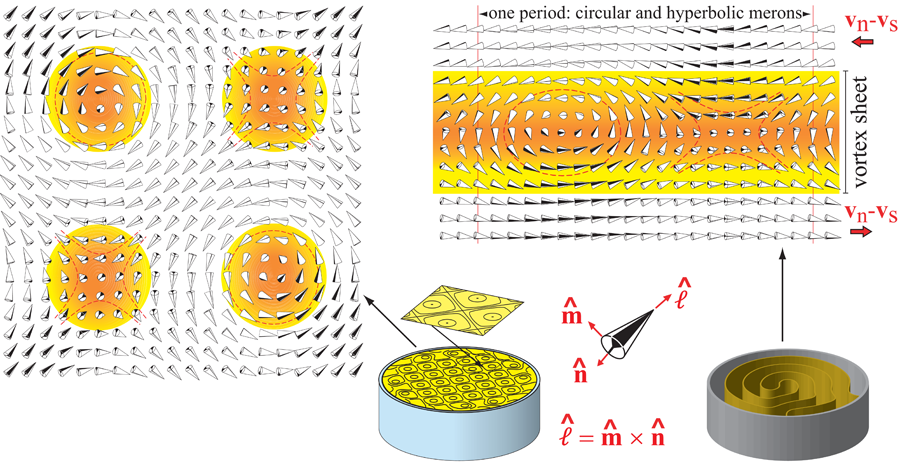
The fact that the first experimental identification of chirality in orbital textures of coherent quantum systems is performed in superfluid 3He- A is not surprising: the A and B phases of superfluid 3He are among the most universal representatives of condensed-matter physics. They display properties similar to those of many different classes of materials, ranging from ordered magnets to liquid crystals. Owing to this versatility they have been used to simulate different phenomena from high-energy physics and cosmology [3]. One example is analog experiments on the mechanism of baryogenesis in the early Universe, as explained to result from the chiral anomaly. However, the 3He superfluids exist only at very low temperatures of order 1 millikelvin, making the experiments challenging, especially while applying rotation. Rotation is needed to grow large-size ordered textures and to identify their direction of windedness. In particular, to study the structure of topological defects, such as the vortex textures in Fig. 1, rotation is imperative. The measurements of Walmsley and Golov were performed in a rotating refrigerator, where a scheme was worked out to grow two different single-domain orbital textures in a volume of macroscopic size.
The Manchester experiments are concerned with vortex structures called skyrmions, originally denoting topologically twisted continuous field configurations in quantum field theory, as first described by Tony Skyrme [6]. In 3He- A, vortex skyrmions are linear objects, which, in rotation, are arranged in a lattice or confined within a domain wall as a vortex sheet (Fig. 1). Their quantized circulation is related to the topological winding number of the skyrmion texture, as explained by David Mermin and Jason Ho [7]. Skyrmion lattices have also been discovered in magnetic materials [8]. In both 3He- A and in chiral magnetic systems, an effective electrodynamics emerges, although from different origins, but in both cases this leads to an extra force acting on the skyrmions. In 3He- A this force gives rise to the analog of baryogenesis. Observations of skyrmions have also been reported in Bose-Einstein condensates formed in cold atomic gas clouds, and they have been discussed in the context of the fractional quantum Hall effect in many materials, lately also in graphene.
Walmsley and Golov made use of the properties of skyrmion textures to control the chirality. In 3He- A the Cooper pairs each have angular momentum ħ that tends to be ferromagnetically ordered. The common direction of momentum in this orbital ferromagnet is denoted by the unit vector ˆl. These combine to produce the total angular momentum L. As in ferromagnets, also in 3He- A with broken time-reversal symmetry, the time-reversal operation changes the direction of the orbital momentum to the opposite. When 3He- A is confined in a slab geometry between two walls, as in the Manchester torsional oscillator experiment, the ˆl vector is oriented normal to the boundaries, ˆl=±ˆz. In the absence of rotation, these two states are equivalent: they transform to each other by time-reversal operation. In rotation, the twofold degeneracy is lifted, so that the two textures have slightly different energies. Therefore, when cooled through Tc in rotation, only one of the two states is realized—the one which is energetically preferable.
To resolve which state emerges, the Manchester team studied the critical rotation velocities to see where ˆl starts to be deflected away from ±ˆz, first by vortex-free rotation and then by the formation of vortices. This dependence on angular velocity is different when rotation is applied in different directions. The ground state proves to be that where ˆl is antiparallel to the initial bias rotation Ω at which the superfluid transition was crossed. This result is counterintuitive, since one would expect that the total angular momentum L would be oriented along the angular velocity Ω. However, looking at the vortex-skyrmion textures (Fig. 1) that emerge under rotation, small differences in the total energy are to be expected, and the situation with ˆl∥-Ω arises from introducing the circular skyrmion in the disk, which has lower energy.
In contrast, introduction of the hyperbolic skyrmion enhances the tendency to split the single-domain sample with domain walls to a multidomain structure in subsequent rotations. Domain walls easily trap more circulation so that multidomain samples display low critical velocity and often nearly nonhysteretic behavior with increasing or decreasing rotation, owing to the emergence of the vortex sheet structure (Fig. 1, right).
The high-resolution torsional oscillator measurements of Walmsley and Golov [4] (with approximately single-vortex detection sensitivity) have shown that it is possible to create coherent quantum states with prescribed chirality. This confirms earlier expectations from numerical calculations and will help in future studies of different novel phenomena attributed to the chiral nature of 3He- A or its electron analog Sr2RuO4, a chiral p-wave spin-triplet superconductor [5]. Better control and measurement of order-parameter textures will be of importance to resolve the long-standing dispute about the magnitude of the total angular momentum L in a macroscopically coherent system, or to resolve the Weyl, Majorana, and Dirac fermions, which originally were introduced in particle physics. The Manchester disk-shaped sample geometry, for instance, allows probing of the Majorana fermions, which are present only in surface states on the lateral cylindrical wall and whose energy spectrum forms a Fermi arc. Other Majorana fermions are expected in the core of novel topological objects, such as the not-yet-observed half-quantum vortex, known as an Alice string in particle physics, whose fermionic energy spectrum has no dispersion [9].
References
- T. D. Lee and C. N. Yang, “Question of Parity Conservation in Weak Interactions,” Phys. Rev. 104, 254 (1956)
- S. Adler, “Axial-Vector Vertex in Spinor Electrodynamics,” Phys. Rev. 177, 2426 (1969); J. S. Bell and R. Jackiw, Nuovo Cimento A 60, 47 (1969)
- G. E. Volovik, The Universe in a Helium Droplet (Clarendon Press, Oxford, 2003)[Amazon][WorldCat]
- P. M. Walmsley and A. I. Golov, “Chirality of Superfluid 3He-A,” Phys. Rev. Lett. 109, 215301 (2012)
- J. Xia, Y. Maeno, P. T. Beyersdorf, M. M. Fejer, and A. Kapitulnik, “High Resolution Polar Kerr Effect Measurements of Sr2RuO4: Evidence for Broken Time-Reversal symmetry in the Superconducting State,” Phys. Rev. Lett. 97, 167002 (2006)
- T. Skyrme, “A Unified Field Theory of Mesons and Baryons,” Nucl. Phys. 31, 556 (1962)
- N. D. Mermin and T.-L. Ho, “Circulation and Angular Momentum in the A Phase of Superfluid Helium-3,” Phys. Rev. Lett. 36, 594 (1976)
- S. Mühlbauer, “Skyrmion Lattice in a Chiral Magnet,” Science 323, 915 (2009)
- T. T. Heikkilä, N. B. Kopnin, and G. E. Volovik, “Flat Bands in Topological Media,” JETP Lett. 94, 233 (2011)
- V. B. Eltsov and M. Krusius, in Topological defects and the non-equilibrium dynamics of symmetry breaking phase transitions, edited by Y. M. Bunkov and H. Godfrin, Proceedings of the NATO Advanced Study Institute (Kluwer Academic, Netherlands, 2000), pp. 325-344[Amazon][WorldCat]