Peering Back to the Beginning of Time

Lawrence M. Krauss

Viewpoint

Peering Back to the Beginning of Time

Lawrence M. Krauss

Department of Physics and School of Earth and Space Exploration, Arizona State University, Tempe, AZ 85287, USA
Mount Stromlo Observatory, Research School of Astronomy and Astrophysics, Australian National University, Weston, ACT 2611, Australia

June 19, 2014• Physics 7, 64

The BICEP2 collaboration reports the detection of

B

-mode polarization of the cosmic microwave background—a signal that might originate from gravitational waves created by inflation during the very earliest moments in the evolution of our Universe.

Figure caption — **Figure 1:** (Top) $B$ -mode polarization pattern observed by the BICEP2 telescope [1]. The twisting pattern in the polarization of the cosmic microwave background (CMB) might originate from gravitational waves created by inflation in the very first moments of the universe’s expansion. The line segments display the magnitude and orientation of linear polarization at different spots of the sky. (Bottom) Power of CMB polarization modes decomposed into spherical harmonic modes ( $l$ ). Higher $l$ modes correspond to smaller angular scales of the sky. The BICEP signal is above expected backgrounds (solid line) for $l$ less than about 150 and follows well the predicted shape of an inflationary signal (dashed line). Note that dust foreground effects are poorly constrained at the moment and could be significant.

Inflation—the hypothesis that the Universe underwent a phase of superluminal expansion in a brief period following the big bang—has the potential of explaining, from first principles, why the Universe has the structure we see today. It could also solve outstanding puzzles of standard big-bang cosmology, such as why the Universe is, to a very good approximation, flat and isotropic (i.e., it looks the same in all directions). Yet we do not yet have a compelling model, based on fundamental particle physics principles, that explains inflation. And despite its explanatory power and a great deal of suggestive evidence, we still lack an unambiguous and direct probe of inflation. Theorists have developed different models for inflation, which all share a common, robust prediction: Inflation would have created a background of gravitational waves that could have an observable effect. These waves would cause subtle, characteristic distortions of the cosmic microwave background (CMB)—the oldest light in the Universe, released when photons decoupled from matter and the Universe became transparent to radiation.

Until now, such a background of gravitational waves has escaped detection. It is therefore very exciting that the BICEP2 (Background Imaging of Cosmic Extragalactic Polarization) collaboration has now reported in Physical Review Letters the observation of polarization modes of CMB radiation [1] that are consistent with such a background. If confirmed, BICEP2’s finding would provide the first direct probe of physical processes that occurred a mere $10^{- 36}$ seconds after the big bang. The result also offers a rare opportunity to directly test theoretical ideas, including inflation and grand unification (the theory that merges the electromagnetic, weak, and strong forces into a single force), at energies 13 orders of magnitude greater than we can currently access at the Large Hadron Collider.

Inflation is based on the possibility that a scalar field in the early Universe got momentarily stuck in a “false vacuum state”—a metastable condition in which the Universe wasn’t at its global potential minimum. The energy stored in this vacuum state could drive an exponential expansion of spacetime (similar to, but much faster than, the expansion due to dark energy seen today) before a phase transition to the true vacuum state occurs, releasing this energy into particles and radiation. This creates an initially hot state for a subsequent big-bang-like expansion from which our Universe subsequently evolved [2]. If the Universe grew by at least a factor of $10^{26}$ , inflation can explain both the apparent flatness and isotropy of the Universe [2]: The expansion would stretch any initial curvature of the Universe to near flatness, and smooth out any initial inhomogeneities, just as stretching a piece of fabric would do. Isotropy is justified by the fact that the Universe, before inflation, was once much smaller and could have been contained in a causally connected region that was in thermal equilibrium, resulting in the approximately uniform, isotropic temperature distribution observed on large scales today. Importantly, the theory can explain the origin of structure in the Universe: Microscopic quantum fluctuations associated with the scalar field driving inflation could have been “frozen in” during the exponential expansion, resulting, after inflation, in density fluctuations that might have seeded all observed large-scale structures in the Universe, including all visible galaxies and clusters of galaxies.

The signs of such primordial fluctuations have indeed been detected through their effect on the CMB, first by the Cosmic Background Explorer (COBE) [3] and later by other missions. These observational studies detected small temperature variations (anisotropies) in the CMB and found a spectrum compatible with that predicted by inflation. The magnitude of these fluctuations suggests an energy scale of inflation (loosely speaking, the energy stored throughout space in the scalar field potential that drove inflation) close to $10^{16}$ giga-electron-volts (GeV). But while consistent with inflation, such fluctuations are not conclusive evidence: Their predicted shape and magnitude is known to vary with the details of the inflationary potential (whose form is not yet experimentally constrained). Hence many different observations could be accommodated, and any agreement between theory and observation is at best suggestive. There is also a possibility that other, noninflationary sources might contribute.

However, gravitational waves—the propagating ripples in spacetime predicted by Einstein in 1916—might provide direct evidence of inflation. During inflation, gravitational fields would also fluctuate because of quantum effects. These fluctuations would similarly get “frozen in” and re-emerge after inflation as classical gravitational waves, whose amplitude depends only on the energy stored in the field driving inflation, and not on other model-dependent details [4]. If the scale of inflation were indeed as high as a few times $10^{16}$ GeV, this background could produce specific temperature anisotropies in the CMB [5]. These anisotropies have thus far not been detected by the most recent searches by the WMAP and PLANCK satellites, and their absence was used to put upper limits on the scale of inflation somewhat below $10^{16}$ GeV.

But a more sensitive CMB probe of gravitational waves exists. Large-wavelength gravitational waves produced by inflation would have alternatively compressed and expanded space, producing spatial variations in the intensity of radiation bombarding free electrons in the early Universe (see, e.g., Ref: [6]). As photons decoupled from matter and CMB radiation was released, scattering from the undulating sea of electrons would have created patterns in the polarization of CMB. Such polarization anisotropies are predicted in inflationary models to be most visible when looking at the sky on spatial scales comparable to or somewhat larger than the size of the Universe’s horizon at the time when the CMB was created. This corresponds to about 1 degree of the field of view of the sky. Over the past decade, various CMB polarization experiments have thus chased the observation of polarization anisotropies on these scales but, so far, also yielded merely upper limits on a possible gravitational background.

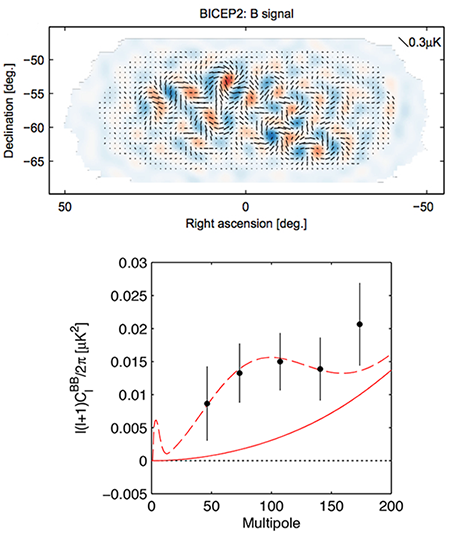
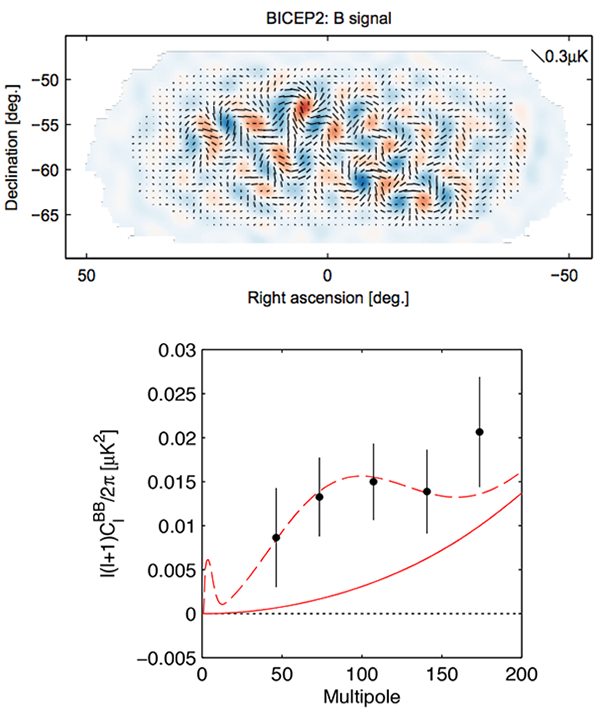
All of this has now changed with the BICEP2 collaboration’s [1] report of a signal that has the predicted characteristics of such a background at precisely the angular scales where the inflationary signal is expected (see Fig. 1, top). The BICEP2 Collaboration uses a microwave telescope located at the South Pole, with an array of polarization-sensitive detectors pointing at a small field (a solid angle of about $1$ percent of the full sky) with a resolution of approximately $0.5$ degrees, thus sufficient to probe the relevant angular scales for the detection of polarization anisotropies originating from inflation.

BICEP2’s search is based on two expected features of primordial gravitational waves. First, gravitational waves would produce a characteristic spatial polarization pattern on CMB—a consequence of their quadrupole nature. One can decompose any spatial polarization pattern into two orthogonal components, a “curl-free” component and a component involving a twisting pattern. In analogy to electromagnetic modes, these polarization patterns are called “ $E$ modes” and “ $B$ modes,” respectively (see Fig. 1). Scalar fluctuations in the density of matter would produce $E$ modes, while gravitational waves would instead generate a $B$ -mode pattern. The second feature is the aforementioned characteristic spatial scale of expected CMB polarization signatures, including wavelength scales comparable to or larger than the horizon scale at the time the CMB was created.

Since inflation would produce both gravitational waves and scalar density perturbations, BICEP2, like all CMB-polarization experiments, quote their results in terms of the ratio $r$ of the amplitude of tensor perturbations (associated with gravitational waves) to the amplitude associated with scalar density fluctuations (reflected in the magnitude of CMB temperature fluctuations measured by experiments like COBE). A nonzero $r$ implies the detection of a $B$ -mode pattern and primarily measures the strength of any possible gravitational wave signal which, if it comes from inflation, is then determined by its energy scale (e.g., see Ref. [7] for a review). BICEP2 reported a nonzero value of $r = 0.2 (+ 0.07 - 0.05)$ , on scales ranging from the horizon scale up to 4–5 times larger, with a claimed significance of better than $5 σ$ . If confirmed, a value of 0.2 corresponds to an energy density during inflation of $\sim 1.2 \times 10^{16}$ GeV.

The claimed signal is far larger than expected, partly because the PLANCK satellite, despite not having yet reported its polarization measurements, has previously put an upper limit on $r$ ( $< 0.11$ ). PLANCK’s estimate is based on the absence of a gravitational wave signature on the CMB temperature anisotropy on its largest scales (PLANCK observes the whole sky and therefore angular scales up to $360$ degrees). The apparent discrepancy between the PLANCK upper bound and the BICEP2 detection might be a cause for concern. However, the two bounds probe different spatial scales, and the BICEP collaboration has noted that, while tensor perturbations are expected to be roughly scale independent, some scale dependence of scalar density perturbations could relax the PLANCK constraint and bring the two results into agreement. Of greater concern, of course, is the possibility that the signal could be determined by foreground contamination—any source along the line of sight of the detector that could distort or contaminate the primordial CMB signal. BICEP2 tested for a wide variety of possible foregrounds, claiming they cannot be responsible for the observed signal. One obvious candidate arises from gravitational lensing: The intervening mass of astrophysical bodies could distort the spatial pattern of polarization in a way that that could transform an $E$ -mode signal into a $B$ -mode signal. The BICEP collaboration has analyzed this possibility extensively. Based on measurements of CMB temperature (and hence density perturbations) and on models of the mass distribution, they argue that such effects would only be significant on smaller angular scales than those contributing to their measurements of $r$ .

But since BICEP2’s first announcement on March 17, 2014, a number of scientists have raised another foreground concern due to the possible impact of our Galaxy’s dust. BICEP2 modeled dust contamination scenarios based on a variety of theoretical models, using data from its predecessor BICEP1 and preliminary dust estimates from the PLANCK satellite. They concluded that dust could not reproduce the magnitude of the observed signal. However, PLANCK has in the meanwhile released more complete measurements of variation in polarized dust foregrounds in many regions of the Galaxy. Their analysis increases the uncertainty associated with the dust contribution to the BICEP2 result and also accommodates a potentially larger polarized dust contribution. Some researchers argued that dust polarization at a level 2–3 times higher than that assumed by BICEP2 is possible and could therefore plausibly be responsible for the entire signal (e.g., see Ref: [8]). While the BICEP2 collaboration continues to maintain that this is extremely unlikely based on their estimates, many other researchers are more skeptical. In any case, a variety of upcoming measurements, from PLANCK and other detectors, are expected to resolve this important ambiguity within the coming year.

Even if the BICEP2 signal does arise from primordial gravitational waves, various consistency checks will be required before it can be definitively argued that the signal arises from inflation. Inflation is certainly the best-motivated possibility, and the spectral shape of the signal, at least for the five largest-scale modes observed by BICEP2, agrees extremely well with inflationary predictions (see Fig. 1, bottom). Several results have already appeared that strongly constrain other possible sources, ruling out, for example, the possibility of gravitational waves generated by other possible phase transitions after inflation [9]. This conclusion is reinforced by more careful analyses of the scale dependence of the predicted signal (e.g., Ref. [10]). But it will nevertheless be important to assure that all possible alternative sources of gravitational waves are ruled out.

If it can be established that the BICEP2 represents a gravitational wave signal from inflation, there is one additional exciting implication. Standard calculations suggest that the gravitational wave signal from inflation is due to quantum fluctuations in the gravitational field, and this appears to require the existence of gravitons—the hypothetical elementary particles that mediate the force of gravity. Research by Frank Wilczek and myself, based on a dimensional analysis argument [11], suggests that, independently of the method used to calculate the gravitational wave spectrum, a quantum gravitational origin is required. If so, the BICEP2 result then implies that gravity is ultimately a quantum theory, a result of fundamental importance for physics.

It is hard to imagine a more important or wider-ranging cosmological observation. If confirmed by upcoming experiments, the BICEP2 results will push the frontiers of empirical physics forward in an unprecedented way, offering the first direct observation of gravitational waves and of quantum gravity effects as well as a new probe of the earliest moments in the Universe’s life and of physics at grand-unified energy scales. This will thus constrain both our understanding of fundamental interactions and the evolution of our Universe as a whole.

References

P. A. R. Ade et al. (BICEP2 Collaboration), “Detection of $B$ -Mode Polarization at Degree Angular Scales by BICEP2,” Phys. Rev. Lett. 112, 241101 (2014)
A. H. Guth, “Inflationary Universe: A Possible Solution to the Horizon and Flatness Problems,” Phys. Rev. D 23, 347 (1981M); A. Linde, “A New Inflationary Universe Scenario: A Possible Solution of the Horizon, Flatness, Homogeneity, Isotropy and Primordial Monopole Problems,” Phys. Lett. B 108, 389 (1982)
G. F. Smoot et al., “Structure in the COBE Differential Microwave Radiometer First-Year Maps,” Astrophys. J. 396, L1 (1992)
V. A. Rubakov, M. V. Sazhin, and A. V. Veryaskin, “Graviton Creation in the Inflationary Universe and the Grand Unification Scale,” Phys. Lett. B 115, 189 (1982); A. A. Starobinskii, “Spectrum of Relict Gravitational Radiation and the Early State of the Universe,” JETP Lett. 30, 682 (1979)
L. M. Krauss and M. White, “Grand Unification, Gravitational Waves, and the Cosmic Microwave Background Anisotropy,” Phys. Rev. Lett. 69, 869 (1992)
M. Kamionkowski, A. Kosowsky, and A. Stebbins, “A Probe of Primordial Gravity Waves and Vorticity,” Phys. Rev. Lett. 78, 2058 (1997); U. Seljak, and M. Zaldarriaga, “Signature of Gravity Waves in the Polarization of the Microwave Background,” 78, 2054 (1997)
L. M. Krauss, S. Dodelson, and S. Meyer, “Primordial Gravitational Waves and Cosmology,” Science 328, 989 (2010)
M. Mortonson and U. Seljak, “A Joint Analysis of Planck and BICEP2 $B$ Modes Including Dust Polarization Uncertainty,” arXiv:1405.5857V1; R. Flauger, J. C. Hill, and D. N. Spergel, “Toward an Understanding of Foreground Emission in the BICEP2 Region,” arXiv:1405.7351
J. B. Dent, L. M. Krauss, and H. Mathur, ““Killing the Straw Man: Does BICEP Prove Inflation at the GUT Scale?,” arXiv:1403.5166
R. Durrer, D. G. Figueroa, and M. Kunz, “Can Self-Ordering Scalar Fields Explain the BICEP2 $B$ -Mode Signal?,” arXiv:1404.3855; J. Lizarraga, J. Urrestilla, D. Daverio, M. Hindmarsh, M. Kunz, and A. R. Liddle, “Can Topological Defects Mimic the BICEP2 $B$ -Mode Signal?,” Phys. Rev. Lett. 112, 171301 (2014); A. Moss and L. Pogosian, “Did BICEP2 See Vector Modes? First $B$ -Mode Constraints on Cosmic Defects,” 112, 171302 (2014)
L. M. Krauss and F. Wilczek, “Using Cosmology to Establish the Quantization of Gravity,” Phys. Rev. D 89, 047501 (2014)

About the Author

Lawrence M. Krauss is Director of the Origins Project and Foundation Professor in the School of Earth and Space Exploration, and Department of Physics at Arizona State University. He is a theoretical physicist with wide research interests, primarily at the interface between particle physics and cosmology. Specific areas include neutrino astrophysics and cosmology, gravitational waves, general relativity, dark matter, dark energy, primordial nucleosynthesis, quantum field theory, and the physics of the very early Universe. Awards from US physics societies include, the Lilienfeld Prize from the APS, the Gemant Award from the AIP, the Oersted Medal from the AAPT, and the Public Welfare Medal from the National Science Board. More information is available at www.krauss.faculty.asu.edu.