High Chern Numbers in Photonic Crystals
The romance with topological physics is now more than thirty years old and remains as fiery as ever [1]. Some see the primary importance of the class of materials called topological insulators as enabling revolutionary technologies such as quantum computers and spintronic devices, others as the realization of a new type of order that goes beyond conventional phases of matter, or as a real-world manifestation of the most abstract mathematical theories of topology.
But in my view, the most enticing feature of topological behavior is its universality, the fact that the physical properties of a system are characterized by a single topological number, rather than by specific system-dependent details. This implies that systems made of entirely different microscopic elements, but whose topological numbers are the same, are fundamentally equivalent. Now, Marin Soljačić at the Massachusetts Institute of Technology, Cambridge, and colleagues report a striking example of this universality [2]. The authors investigate the propagation of photons in two-dimensional photonic crystals known to exhibit an optical version of the electronic “quantum anomalous Hall effect” [3]—a prototypical topological phenomenon. Their experiments demonstrate that the universal number that captures the properties of such crystals—the “Chern number,” which characterizes the number of topologically protected states—takes on unprecedentedly large values. The availability of multiple topological channels might enable novel photonic devices and effects that would be impossible with Chern numbers limited to 1.
The use of abstract topological concepts to describe condensed-matter systems was first put forward in the description of the integer quantum Hall effect by David Thouless, Mahito Kohmoto, Peter Nightingale, and Marcel den Nijs [4]. They showed that the Hall conductance of a two-dimensional electron system under a large magnetic field can be quantized, that is, fixed to be an integer times a fundamental constant, . That integer, known as the Chern number, corresponds to the number of edge states that carry current, without scattering, across the system. The Chern number indicates topological behavior in the sense that small deformations of the system (such as disorder, strain, and localized defects) have little effect on its properties. This makes the system’s physics independent of its minute details (such as hopping parameters, number of dopants, or atomic constituents) and only dependent on this global quantity, which must be an integer. In other words, it depends on the system’s topology rather than on its geometry.
Although the initial interest in topological phenomena focused on electrons, topological behavior can be generalized to any type of wave, including light, as first predicted in Ref. [5]. A magnetic photonic crystal—a photonic crystal made of materials that break Lorentz reciprocity due to their response to an external magnetic field—can have a nonzero Chern number (and associated nonscattering edge states) for electromagnetic modes, that is, photons, rather than electrons. Soljačić’s group demonstrated this in 2009, showing that a topological protection of microwave photons could be observed in photonic crystals [6]. The finding launched a search for the realization of topological insulators for photons at optical wavelengths [7], motivated by the prospect of engineering robust optical devices, immune to disorder. Using similar mechanisms as in Ref. [6] proved challenging because of the weakness of magnetic effects in the optical regime. But alternative mechanisms later enabled robust topological edge states for light in a photonic lattice [8] and silicon ring resonator arrays [9].
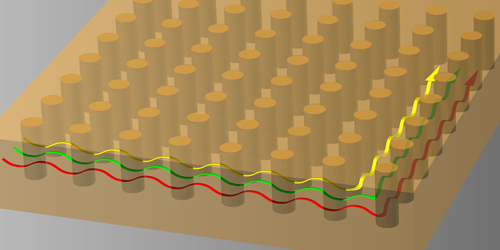
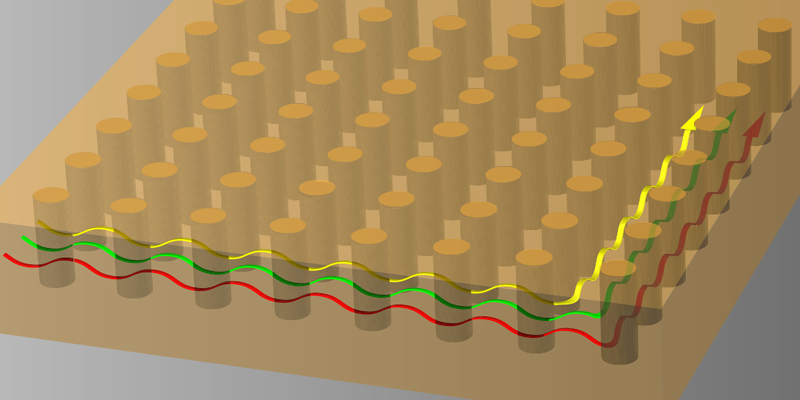
Employing a microwave photonic crystal similar to the one they used previously [6], Soljačić’s team now reports another important discovery, that of high-Chern number bands in a photonic quantum anomalous Hall system. The authors utilized a scheme (see Fig. 1) based on two-dimensional photonic crystals made of ferromagnetic garnet rods sandwiched between two copper plates. Similar models have previously been realized in photonics [6, 8, 9], cold atomic systems [10], and condensed matter [11], but they were limited to Chern numbers of 1.
By analyzing the dispersion curves of the modes propagating at the edge of the crystal, the group proved that the system behaves like a quantum anomalous Hall system with Chern numbers up to 4. This may give rise to effects and applications that would have been impossible for systems with Chern numbers of 1. For instance, information could be multiplexed over several spatial channels provided by the topological edge modes, leading to faster, higher-capacity, and more robust on-chip communication. Robust nonlinear mode conversion could be achieved by using nonlinear effects to mix the scatter-free modes. Finally, the authors show that the availability of multiple edge states allows a unique routing geometry, in which the states can be split at a corner and sent to different “ports.” Because of topological protection, this rerouting circuit wouldn’t suffer from any losses due to backscattering.
But one of the most interesting aspects of the work lies in the entirely new experimental procedure used to map out the dispersion relation of the topological edge states. The authors’ method can be loosely seen as a photonic analog of photoemission spectroscopy—the method used to characterize the dispersion of two-dimensional electronic states on the surface of three-dimensional topological insulators. For two-dimensional systems, which have one-dimensional topological states at their edges, photoemission spectroscopy would be extremely challenging because an incoming optical probe beam cannot access an extremely thin one-dimensional edge. Two-dimensional quantum topological behavior has thus been typically characterized via transport measurements.
The authors use microwave photons to provide a characterization similar to photoemission, as it can directly access the band structure of the one-dimensional edge states. They scanned the edges of the photonic crystal with microwaves—injected and collected by antennae—determining the amplitude and phase of the electromagnetic field transmitted by the crystal. (An advantage of the microwave regime is that phase information is much easier to collect than in optics.) By Fourier transforming the spatial profile of the edge states, they determined their lattice momentum as a function of frequency, mapping out their band structure and determining the Chern numbers of each band. As a result of the higher-Chern-number bands, there are multiple edge states that cross the band gaps of the photonic crystal [2]. To my knowledge, the data collected by Soljačić’s team are the first direct experimental characterization of the band structure of a one-dimensional topological edge state.
This work demonstrates the surprising generality of topological physics and the fact that alternative wave-based systems, such as photonics, can host new phenomena and observables that would have been inaccessible in other systems. The results pose another significant step towards the dream of “topological photonics,” in which quantum Hall physics and other forms of topological behavior will provide the basis for photonic devices.
This research is published in Physical Review Letters.
References
- M. Z. Hasan and C. L. Kane, “Colloquium : Topological insulators,” Rev. Mod. Phys. 82, 3045 (2010).
- S. A. Skirlo, L. Lu, Y. Igarashi, J. Joannopoulos, and M. Soljačić, “Experimental Observation of Large Chern Numbers in Photonic Crystals,” Phys. Rev. Lett. 115, 253901 (2015).
- F. D. M. Haldane, “Model for a Quantum Hall Effect without Landau Levels: Condensed-Matter Realization of the ‘Parity Anomaly’,” Phys. Rev. Lett. 61, 2015 (1988).
- D. J. Thouless, M. Kohmoto, M. P. Nightingale, and M. den Nijs, “Quantized Hall Conductance in a Two-Dimensional Periodic Potential,” Phys. Rev. Lett. 49, 405 (1982).
- F. D. M. Haldane and S. Raghu, “Possible Realization of Directional Optical Waveguides in Photonic Crystals with Broken Time-Reversal Symmetry,” Phys. Rev. Lett. 100, 013904 (2008); S. Raghu and F. D. M. Haldane, “Analogs of Quantum-Hall-Effect Edge States in Photonic Crystals,” Phys. Rev. A 78, 033834 (2008).
- Z. Wang, Y. D. Chong, J. D. Joannopoulos, and M. Soljačić, “Reflection-Free One-Way Edge Modes in a Gyromagnetic Photonic Crystal,” Phys. Rev. Lett. 100, 013905 (2008); Z Wang, Y. Chong, J. D. Joannopoulos, and M. Soljačić, “Observation of Unidirectional Backscattering-Immune Topological Electromagnetic States,” Nature 461, 772 (2009).
- R. O. Umucalılar and I. Carusotto, “Artificial Gauge Field for Photons in Coupled Cavity Arrays,” Phys. Rev. A 84, 043804 (2011); M. Hafezi, E. A. Demler, M. D. Lukin, and J. M. Taylor, “Robust Optical Delay Lines with Topological Protection,” Nature Phys. 7, 907 (2011); K. Fang, Z. Yu, and S. Fan, “Realizing Effective Magnetic Field for Photons by Controlling the Phase of Dynamic Modulation,” Nature Photon. 6, 782 (2012); A. B. Khanikaev, S. Hossein Mousavi, W.-K. Tse, M. Kargarian, A. H. MacDonald, and G. Shvets, “Photonic Topological Insulators,” Nature Mater. 12, 233 (2012).
- M. C. Rechtsman, J. M. Zeuner, Y. Plotnik, Y. Lumer, D. Podolsky, F. Dreisow, S. Nolte, M. Segev, and A. Szameit, “Photonic Floquet Topological Insulators,” Nature 496, 196 (2013).
- M. Hafezi, S. Mittal, J. Fan, A. Migdall, and J. M. Taylor, “Imaging Topological Edge States in Silicon Photonics,” Nature Photon. 7, 1001 (2013).
- G. Jotzu, M. Messer, R. Desbuquois, M. Lebrat, T. Uehlinger, D. Greif, and T. Esslinger, “Experimental Realization of the Topological Haldane Model with Ultracold Fermions,” Nature 515, 237 (2014).
- C.-Z. Chang et al., “Experimental Observation of the Quantum Anomalous Hall Effect in a Magnetic Topological Insulator,” Science 340, 167 (2013).