An Inside View of Magnetic Skyrmions

Christopher  H. Marrows

Viewpoint

An Inside View of Magnetic Skyrmions

Christopher H. Marrows

School of Physics and Astronomy, University of Leeds, Leeds LS2 9JT, United Kingdom

May 1, 2015• Physics 8, 40

Atomic-scale imaging reveals the shape and size of a technologically interesting magnetic quasiparticle.

Figure caption — **Figure 1:** A skyrmion is a pointlike region of reversed magnetization in a uniform magnet. The skyrmion’s “core”—here, pointed up—is surrounded by an axially symmetric twist that returns the spin texture to the background direction (down). Romming *et al.* applied a magnetic field (red arrow) opposite to the core direction and used a spin-polarized scanning tunneling microscope to track the skyrmion’s shape as it was compressed. The measured shape change agreed with a two-decades old theory, suggesting the theory will be useful for describing skyrmions in devices.

A magnetic skyrmion is the smallest possible perturbation to a uniform magnet: a pointlike region of reversed magnetization, surrounded by a whirling twist of spins. Such skyrmions are quasiparticles—they do not exist in the absence of a magnetic state–and they give rise to emergent electrodynamics that cannot be simply described by Maxwell’s equations. The particles are also attractive candidates for transporting information because they are only a few nanometers in size, very stabile, and easily manipulated with spin-polarized currents that consume little power. Although skyrmions are often described as particlelike, a nearly two-decades old theory [1] makes detailed predictions for a skyrmion’s size and shape. These properties determine the particle’s response to a spin current and an external magnetic field, but are difficult to test because skyrmions are often only a few tens of atoms wide. Niklas Romming and colleagues at the University of Hamburg, Germany, have now imaged the internal structure of magnetic skyrmions in a metal film using a magnetically sensitive scanning tunneling microscopy technique [2]. The high spatial resolution of the technique allowed the researchers to confirm that both the size and shape of the skyrmions are consistent with theory.

The mathematical concept of the skyrmion was invented over fifty years ago by high-energy physicist Tony Skyrme [3]. In quantum field theory, particles are wavelike excitations with a finite lifetime. Skyrme proposed that the stability of hadrons (protons, neutrons, and mesons) could be explained if they were described as topological defects in a quantum vector field. These defects could be considered “protected” because they were characterized by a topological integer that cannot be changed through any continuous deformation of the field. Skyrme’s idea for hadrons did not ultimately become part of mainstream particle physics theory, but several examples of skyrmionlike topologies exist in condensed-matter systems [4]. The concept can, for example, describe quantum Hall systems, certain liquid-crystal phases, and Bose-Einstein condensates.

Skyrmions only form in magnets in which spin interactions favor a magnetic structure with chiral symmetry, such as a twist that is either left- or right-handed. Two decades ago, theorists predicted such twists could occur when spins felt a competition between aligning with their neighbors and being perpendicular to them [1]. In this picture, the strongest interaction between spins is Heisenberg exchange. The energy of this interaction, which favors the collinear alignment of neighboring spins and drives the ordering of many magnets, is given by the Heisenberg exchange integral $J$ . A weaker interaction that favors perpendicular neighboring spins can occur in certain Heisenberg magnets in which electrons experience spin-orbit coupling. The energy of this so-called Dzyaloshinkii-Moriya (DM) interaction is proportional to the DM exchange $D$ and causes spins in a Heisenberg magnet to cant, leading in a natural way to a chiral structure.

The DM interaction only occurs in a material whose atomic structure lacks inversion symmetry, a condition satisfied in the $B 20$ crystal lattice, which is a deformed rock-salt structure. Skyrmions have, for example, been observed in the $B 20$ forms of $MnSi$ [5] and ${Fe}_{0.5} {Co}_{0.5} Si$ crystals [6]. Another way to create a structure that cannot be inverted is to form an interface between two different materials. Forming such an interface between a ferromagnet and a strong spin-orbit metal gives rise to the necessary DM interaction, even in the case when both metals have inversion-symmetric lattices [7]. If the ferromagnet is sufficiently thin, then these interfacial effects can dominate.

In 2013, the University of Hamburg group discovered that a palladium-iron ( $PdFe$ ) bilayer grown on a ( $111$ ) iridium surface can support individual skyrmions [8]. The skyrmions can be created, imaged, and annihilated using a spin-polarized scanning tunneling microscope (STM) tip. Like all STM techniques, this method measures the quantum-mechanical current that tunnels from a surface to the probe tip. In this case, the current depends on the angle between a spin on the probe tip and the spin on the surface, so the technique can map the orientation of spins at each point on the surface. Similarly, the current from the tip is spin polarized and can be used to exert a torque on the spins in the magnet to either create or annihilate a skyrmion.

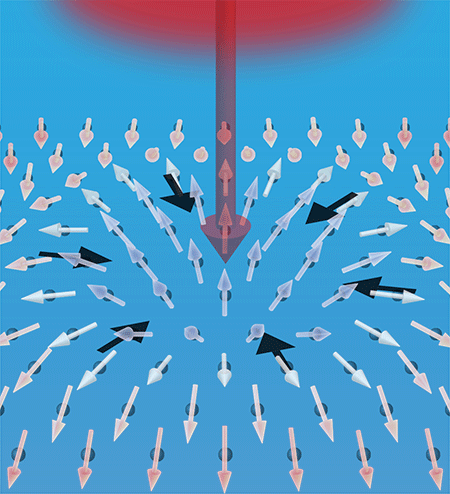
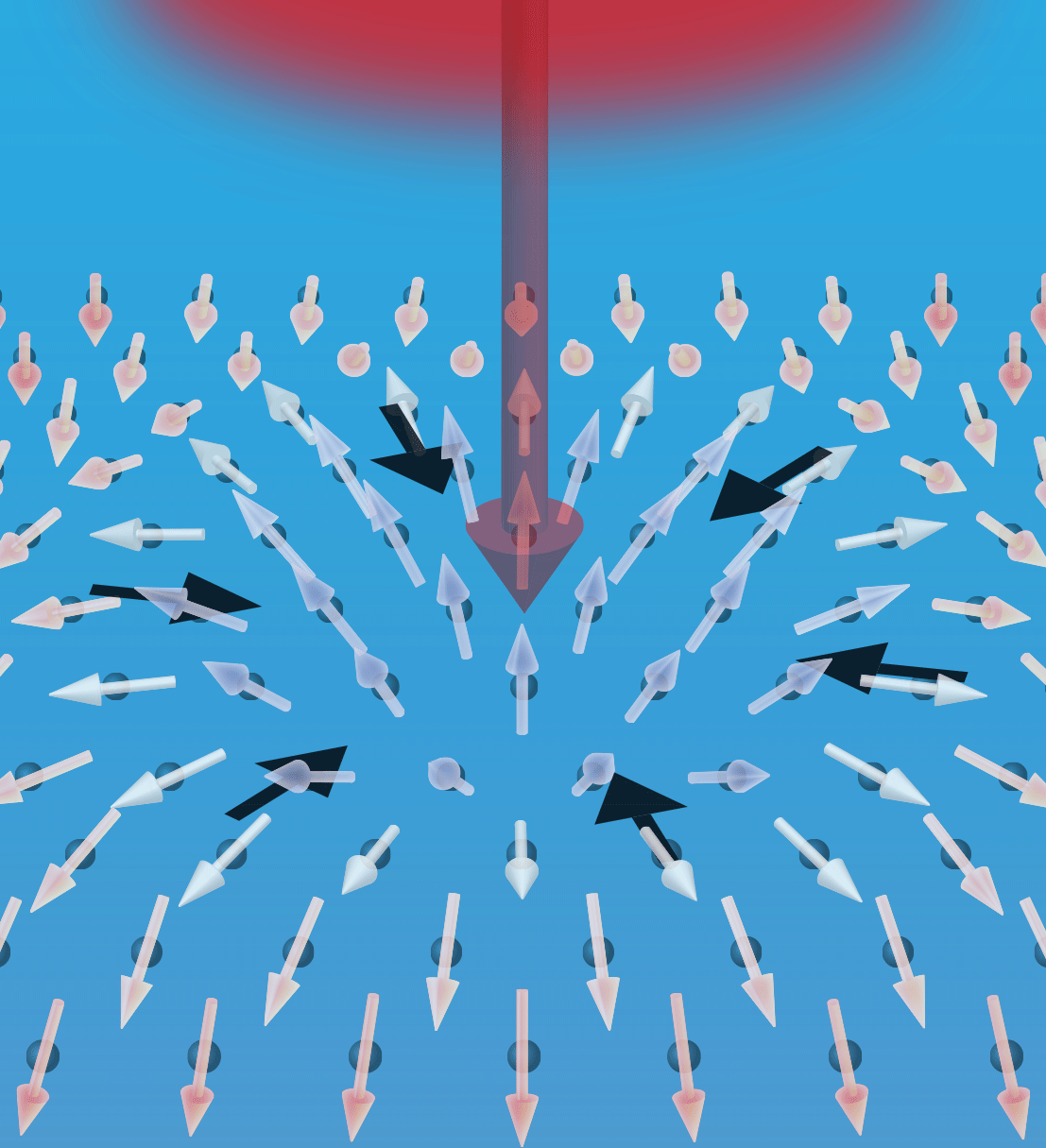
In their new work, Romming et al. use the same technique to image the internal structure of a skyrmion in the $PdFe$ bilayer, and its response to an applied magnetic field. The skyrmion can be thought of as a tiny, 2D bubble-like region whose magnetization points in the opposite direction to the surrounding material. Its core can be considered truly pointlike. But the skyrmion has to have a finite size because it takes a short distance (in this case about 2 nanometers) for the magnetization to twist back to the background direction. Applying a magnetic field in the opposite direction to the skyrmion core magnetization compresses the bubble (Fig. 1), changing its shape. Using the STM, Romming et al. mapped the skyrmion’s shape versus magnetic field as the bubble was compressed to approximately half its equilibrium size. Their results fit the existing theory [1], thus confirming it. These fits also allowed the researchers to determine the values of $J$ and $D$ in their material. These values, which are difficult to measure in other ways, determine the skyrmion’s size, and researchers will need to know them for any technology that makes use of skyrmions.

The physics of skyrmions is fascinating because of their special topological properties [4]. From a technological perspective, skyrmion-based devices have the potential to store and process information at unprecedentedly small sizes and levels of energy consumption. For example, the presence/absence of the skyrmion could serve as the $1$ and $0$ in a data bit in a racetrack memory [9]. The work of Romming et al. shows that existing theories provide a sound basis for the micromagnetic design of such devices. This is a remarkable thing in itself, since these theories are continuum models, yet the new work shows they remain valid at atomic length scales. Moreover, the results show skyrmions are robust: they can withstand a reverse field of almost $3$ teslas. So far, magnetic skyrmions have only been observed at low temperatures. (Romming et al. performed their experiments at $4.2 K$ ). But very recently, researchers have observed skyrmions in a room-temperature magnet, making their promise for future technologies more realistic [10].

This research is published in Physical Review Letters.

References

A. Bogdanov and A. Hubert, “Thermodynamically Stable Magnetic Vortex States in Magnetic Crystals,” J. Magn. Magn. Mater. 138, 255 (1994); “The Properties of Isolated Magnetic Vortices,” Phys. Status Solidi (b) 186, 527 (1994)
Niklas Romming, André Kubetzka, Christian Hanneken, Kirsten von Bergmann, and Roland Wiesendanger, “Field-Dependent Size and Shape of Single Magnetic Skyrmions,” Phys. Rev. Lett. 114, 177203 (2015)
T. H. R. Skyrme, “A Unified Field Theory of Mesons and Baryons,” Nucl. Phys. 31, 556 (1962)
N. Nagaosa and Y. Tokura, “Topological Properties and Dynamics of Magnetic Skyrmions,” Nature Nanotech. 8, 899 (2013)
S. Mühlbauer, B. Binz, F. Jonietz, C. Peiderer, A. Rosch, A. Neubauer, R. Georgii, and P. Böni, “Skyrmion Lattice in a Chiral Magnet,” Science 323, 915 (2009)
X. Z. Yu, Y. Onose, N. Kanazawa, J. H. Park, J. H. Han, Y. Matsui, N. Nagaosa, and Y. Tokura, “Real-Space Observation of a Two-Dimensional Skyrmion Crystal,” Nature 465, 901 (2010)
A. Fert, “Magnetic and Transport Properties of Metallic Multilayers,” in Materials Science Forum, Vol. 59, edited by A. Chamberod and J. Hillairet (Trans Tech, Zurich, 1989)[Amazon][WorldCat]
N. Romming, C. Hanneken, M. Menzel, J. E. Bickel, B. Wolter, K. von Bergmann, A. Kubetzka, and R. Wiesendanger, “Writing and Deleting Single Magnetic Skyrmions,” Science 341, 636 (2013)
N. S. Kiselev, A. N. Bogdanov, R. Schäfer, and U. K. Rößer, “Chiral Skyrmions in Thin Magnetic Films: New Objects for Magnetic Storage Technologies?,” J. Phys. D 44, 392001 (2011); A. Fert, V. Cros, and J. Sampaio, “Skyrmions on the track,” Nature Nanotech. 8, 152 (2013)
C. Moreau-Luchaire et al., “Skyrmions at Room Temperature: From Magnetic Thin Films to Magnetic Multilayers,” arXiv:1502.07853 (2015); S. Woo et al., “Observation of Room Temperature Magnetic Skyrmions and Their Current-Driven Dynamics in Ultrathin Co Films,”arXiv:1502.07376 (2015)

About the Author

Christopher Marrows is Professor of Condensed Matter Physics at the University of Leeds. He received his Ph.D. from the University of Leeds in 1997, where he was a Research Fellow of the Royal Commission for the Exhibition of 1851 at the same institution from 1998 to 2000. He is a Fellow of the Institute of Physics and a Senior Member of the IEEE. He gave the 2011 Wohlfarth Lecture in recognition of his work on nanomagnetism. His current research interests include many aspects of nanomagnetism and spintronics, with the general theme of emergent properties of condensed-matter systems, including emergent quasiparticles such as magnetic monopoles and skyrmions.