Taming Ultracold Molecules

Susanne Yelin

Viewpoint

Taming Ultracold Molecules

Physics Department, University of Connecticut, Storrs, CT 06269, USA

June 3, 2016• Physics 9, 60

Riding the coattails of cold atomic physics, researchers have demonstrated the ability to steer cold molecules into desired quantum states.

Figure caption — **Figure 1:** In addition to electronic states, molecules have vibrational, rotational, and hyperfine states. Researchers have characterized the energy levels and transition frequencies between the lowest rovibrational state, $J = 0$ , and the first rotationally excited state, $J = 1$ , which is split into three projection states $(m_{J} = 1, 0, - 1)$ . Both of these states have considerable hyperfine substructure. By mapping out this complicated state space, the researchers were able to perform precise transitions between states using microwave laser light.

Ultracold atoms have become a favorite tool in physics because they can be precisely placed in a quantum state using optical and magnetic fields. This quantum control has been crucial for understanding fundamental quantum-mechanical behavior and for creating metrological devices such as the atomic clocks that keep time for GPS systems. Current efforts are devoted to using these controllable systems to simulate, for example, superconductivity, but this and other future applications will likely require that the particles within the system interact with each other. Ultracold atoms do not interact very strongly, so an obvious alternative is to turn to molecules. As opposed to atoms, molecules can have an electric dipole, which lets them naturally interact strongly with each other through dipole forces. But molecules are not a straight substitute for atoms. They are much more complicated and thus significantly harder to cool and control than atoms. Martin Zwierlein and co-workers at the Massachusetts Institute of Technology, Cambridge [1], report the first quantum control of an ultracold gas of stable dipolar molecules (sodium potassium, or NaK). This is an important step towards the goal of cooling and manipulating molecules with the same ease and precision as is possible with atoms.

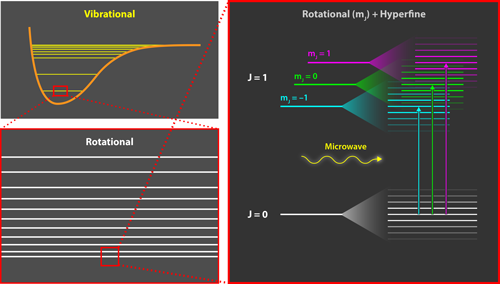
Molecules took center stage in ultracold physics about a decade ago, with several research groups looking for ways to cool and control them by using traps and fields. These techniques—routinely used for atoms—have to be much more refined for molecules. Why? In an atom, the electrons live in discrete orbits that are well-separated in energy. At room temperature, the electrons are in the ground state, and transitions to higher energy states follow clear selection rules. For the last 20 years, physicists have used these favorable properties to cool atoms to their absolute ground state, where they can form a Bose condensate, for example. Molecules, on the other hand, have orders-of-magnitude-more states based on electronic, vibrational, and rotational degrees of freedom, as well as hyperfine and Zeeman splitting. Researchers need detailed and hard-to-acquire knowledge of the molecular configuration to excite specific transitions in this dense patchwork of states. The traditional atomic tools of laser and evaporative cooling are not easily applicable to molecules because both types of cooling rely on the fact that particles by themselves relax into predictable states, which is not the case for most molecules.

In previous experiments, several molecules have been prepared in their absolute ground states. The prime example was potassium rubidium (KRb) in a 3D optical lattice [2], in which the hyperfine sublevels were also controlled [3]. However, an optical lattice gives a different environment in terms of how dense and how ordered the molecules are. In particular, the phase-space density (i.e., the density in both space and frequency) that is necessary to reach condensation can, so far, only be reached in a gas.

In recent work, Zwierlein’s team demonstrated that they could cool a gas of fermionic NaK molecules to submicrokelvin temperatures [4]. They did this by combining a tunable magnetic field with well-timed polarized laser pulses, as was previously done in [3]. And like previous work, Zwierlein’s team showed that they could hold their NaK molecules for several seconds, a necessary condition for quantum control. This ultracold molecular gas (with a density of 10¹¹cm^-3 and a temperature below 1 $𝜇$ K) is the starting point of their latest work [1] in which the authors manipulate the lowest transitions between rotational, hyperfine, and Zeeman states of NaK molecules in their vibrational ground state. To achieve such control, the team gathered detailed knowledge of the properties of all transitions and their selection rules. From basic molecular theory it is known that the lowest $(J = 0)$ rotational state has a hyperfine substructure of 36 states, whereas the first excited $(J = 1)$ rotational state has a substructure of 108 states (see Fig. 1). To characterize all the transitions between these states in terms of exact frequencies and polarizations, Zwierlein’s team performed five steps. First, they used a theoretical model to calculate the “best guess” resonances; second, they measured resonances in the molecules while varying external magnetic and electric fields; third, they used the field dependencies of the measured resonances to assign them to a theoretical counterpart; fourth, they employed statistical methods to adjust the theoretical model; and finally, they assigned all the resonances with this updated model.

Having tabulated all the transitions, the researchers could now steer the NaK molecules into the desired quantum states. As a demonstration, they used a laser pulse (a $𝜋$ -pulse) to transfer all the population from the $J = 0$ ground state to a well-defined hyperfine state of $J = 1$ . Remarkably, the lifetime in $J = 1$ is similar to the one in $J = 0$ , due to stability against collisions at low temperature. The team also performed Rabi oscillations, in which a continuous laser cycles the population between two states. Rabi oscillations and $𝜋$ -pulses are the basic building blocks for constructing any target molecular state, such as a superposition between rotational levels. This type of superposition is crucial for creating a well-defined dipole that allows tuning of the interactions between molecules.

While these results were extremely challenging to reach, they are not surprising in the sense that full quantum state control of ultracold molecular gases had been “the next important goal” for some time. This achievement should be put into context with other cutting-edge research in the field of ultracold molecules, divisible into two roughly equally large subfields. The first subfield is quantum state control of bialkali molecules, such as NaK and KRb. These are the easiest species to understand and their control builds on extensive experience with alkali atoms. The other subfield is everything else [5–7]: cooling, production, and trapping of selected molecules, as well as collisions, chemistry, and precision measurements [8]. These subfields are highly complementary, but it should be noted that the techniques used for cooling, control, etc., are currently only applicable to relatively small classes of molecules.

As this field progresses, researchers will likely set their sights on two goals. The first is to engineer dipole-dipole interactions in ensembles of ultracold molecules in order to (finally!) simulate complex many-body states of matter. This is clearly something that Zwierlein and his colleagues are gearing up to do. These simulations could, for example, elucidate the ever-elusive inner workings of high-temperature superconductivity. In addition, this controllability will also allow ultracold coherent chemistry, which is different from the traditional statistical equilibrium-based chemistry in that it could be deterministic (that is, one-way). The second goal is to perform evaporative cooling, which is the necessary last step towards reaching the condensate temperature or the temperature of Fermi degeneracy. Control over elastic and inelastic collisions is key to reach this goal, and most of the techniques outlined by the authors and promoted in the other research noted above will probably be employed to see a molecular condensate. Obtaining a condensate would be a similar milestone for molecules as it was for atoms more than 20 years ago.

This research is published in Physical Review Letters.

References

S. A. Will, J. W. Park, Z. Z. Yan, H. Loh, and M. W. Zwierlein, “Coherent Microwave Control of Ultracold ²³Na⁴⁰K molecules,” Phys. Rev. Lett. 116, 225306 (2016).
A. Chotia, B. Neyenhuis, S. A. Moses, B. Yan, J. P. Covey, M. Foss-Feig, A. M. Rey, D. S. Jin, and J. Ye, “Long-Lived Dipolar Molecules and Feshbach Molecules in a 3D Optical Lattice,” Phys. Rev. Lett. 108, 080405 (2012).
S. Ospelkaus, K.-K. Ni, G. Quéméner, B. Neyenhuis, D. Wang, M. H. G. de Miranda, J. L. Bohn, J. Ye, and D. S. Jin, “Controlling the Hyperfine State of Rovibronic Ground-State Polar Molecules,” Phys. Rev. Lett. 104, 030402 (2010).
J. W. Park, S. A. Will, and M. W. Zwierlein, “Ultracold Dipolar Gas of Fermionic ²³Na⁴⁰K Molecules in Their Absolute Ground State,” Phys. Rev. Lett. 114, 205302 (2015).
M. Lemeshko, R. V. Krems, J. M. Doyle, and S. Kais, “Manipulation of Molecules with Electromagnetic Fields,” Mol. Phys. 111, 1648 (2013).
L. D. Carr, D. DeMille, R. V. Krems, and J. Ye, “Cold and Ultracold Molecules: Science, Technology and Applications,” New J. Phys. 11, 55049 (2009).
D. S. Jin and J. Ye, “Introduction to Ultracold Molecules: New Frontiers in Quantum and Chemical Physics,” Chem. Rev. 112, 4801 (2012).
J. Baron et al., “Order of Magnitude Smaller Limit on the Electric Dipole Moment of the Electron,” Science 343, 269 (2014).

About the Author

Susanne Yelin works in theoretical atomic and molecular physics. She received her diploma from the University of Stuttgart, Germany, and a Ph.D. from the Ludwig-Maximilians University in Munich, Germany. After a postdoctoral fellowship at the Hanscom Air Force laboratory/MIT and then at the Institute of Theoretical Atomic and Molecular Physics at the Harvard/Smithsonian Center for Astrophysics, she is now a Professor of Physics at the University of Connecticut. She also joined the faculty of the Harvard Physics Department as a Senior Research Fellow, where she is a Co-PI of the Center for Ultracold Atoms, an NSF Physics Frontier Center. Recently, she received the Willis E. Lamb Award for Laser Science and Quantum Optics. Her research is centered around many-body and nonlinear quantum optics. Her interests include superradiant systems, many-body quantum information science, ultracold molecules, and atomic metamaterials. In 2008 she was recognized as an Outstanding Referee by the American Physical Society.