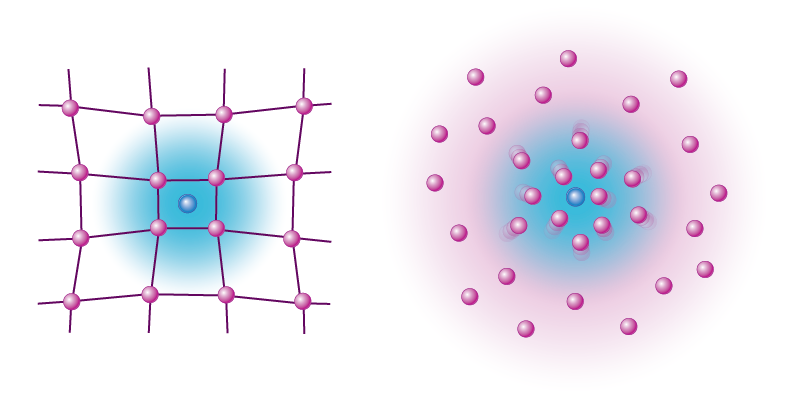
Bose Polarons that Strongly Interact
An electron moving through a solid-state crystal will attract nearby atoms in the lattice causing them to vibrate. Building on earlier work, Herbert Fröhlich proposed in 1950 a model in which this interaction gives rise to a quasiparticle called a polaron, made up of an electron dressed by a cloud phonons, the quanta of acoustic vibration [1]. The physical properties of the polaron, for instance its mobility or its effective mass, can be very different from those of the bare electron, leading to strong modifications of the electrical and thermal transport properties of the material. The problem is that even the simplest case, as described by Fröhlich’s model, is too complex to be solved analytically, so approximations are often made. Researchers are thus trying to study polaron physics using analog systems consisting of gases of ultracold atoms. The results of these simulations provide insights into the underlying physics, as well as offer verification for the approximations made in polaron models.
In two independent works, groups from Denmark [2] and Colorado [3] have demonstrated an atomic implementation of polaronic physics by immersing an impurity inside a Bose-Einstein condensate (see Fig. 1). The impurity interacts with the condensate in a way that is similar to an electron in a crystal. Since, like solids, the low-energy modes of a Bose-Einstein condensate (BEC) are phonons, this system can be described by Fröhlich's model in the regime of weak interactions. But by tuning the strength of interactions, the research teams could also access the regime of strong interaction between the impurity and the BEC, revealing the influence of many-body correlations. The strongly interacting regime of Bose polarons is largely an unchartered territory that may provide insight into superfluid physics, such as in superconductors and liquid helium.
Both these works build on decade-old studies that used ultracold atomic systems to study the fermionic polaron, which is an impurity interacting with an ensemble of spin-polarized fermions [4]. These fermions obey the Pauli principle, which prevents two or more identical fermions from being at the same place. This principle does not apply to bosons, which makes the case of bosonic polarons more challenging, both theoretically and experimentally. Unlike in a Fermi gas, a Bose gas allows interactions between three atoms. These three-body correlations are both a blessing and a curse. On the one hand, they lead to fascinating physical phenomena, such as the celebrated Efimov bound states, a family of universal trimers existing even in the absence of two-body bound states [5]. On the other hand, they allow the formation of bound molecules that effectively remove atoms from the gas. These three-body recombination losses can reduce significantly the lifetime of the polaron system in the strongly interacting regime.
To reduce the effect of these losses during their measurement, the two groups have used a scheme previously developed for the study of fermionic polarons [6]. They first prepared the impurity in a spin state that essentially doesn’t interact with the surrounding condensate. Then, using a short radio frequency pulse, they flipped the spin of the impurity, placing it in a spin state that interacts with the condensate. In their experiment, Ming-Guang Hu and colleagues from the University of Colorado, Boulder, added impurities in the form of potassium atoms to a BEC of rubidium atoms. Nils Jørgensen from Aarhus University, Denmark, and his colleagues actually create the impurities with the radio frequency pulse. They start with a potassium BEC and then use the radio frequency pulse to excite a small fraction of potassium atoms in another spin state, which makes them behave as impurities.
In ultracold-atom systems, it is possible to tune the interaction strength between atoms using an external magnetic field owing to the Fano-Feshbach resonance phenomenon. Using this tool, the two groups varied the interaction between the impurity and the surrounding BEC. As the interaction was made stronger, it altered the resonance condition for the spin flip used to turn on the interactions. Both teams measured this resonance shift by varying the radio frequency of their pulses and observing where the peak occurred in the absorption spectrum. The shifts in the spectra were consistent with the impurities behaving as polarons.
The Boulder group observed that the absorption peaks broadened as the interaction strength increased. They argue that these widths could be used to determine the polaron lifetime once other broadening effects are accounted for. They also imagine that future work could observe the polaron’s effective mass and how it depends on the interaction strength.
To interpret their data, the Danish experimentalists teamed up with theoreticians at Aarhus University and Monash University in Australia, who have developed advanced theoretical methods capable of capturing the three-body correlations. They showed that their radio spectroscopy data were not affected by three-body recombinations and that they could obtain a remarkably good quantitative agreement with experiment. They proved that, as expected, Fröhlich’s model provided an accurate description of the system in the weakly attractive and repulsive regimes, but they also demonstrated the importance of three-body correlations in the strongly interacting regime.
These works pave the way for further studies of strongly correlated Bose gases that would bridge the gap between the physics of ultracold vapors and that of denser systems, like solids or liquid helium mixtures.
This research is published in Physical Review Letters.
Correction (8 August 2016): The text was updated to correct a statement that said Fröhlich was the first to study the interaction giving rise to polarons. In fact, other papers by Solomon Pekar and Lev Landau preceded Fröhlich's work.
References
- H. Fröhlich, H. Pelzer, and S. Zienau, “Properties of Slow Electrons in Polar Materials,” Philos. Mag. 41, 221 (1950).
- N. Jørgensen, L. Wacker, K. Skalmstang, M. Parish, J. Levinsen, R. Christensen, G. Bruun, and J. Arlt, “Observation of Attractive and Repulsive Polarons in a Bose-Einstein Condensate,” Phys. Rev. Lett. 117, 055302 (2016).
- M. G. Hu, M. J. Van de Graaff, D. Kedar, J .P. Corson, E. A. Cornell, and D. S. Jin, “Bose Polarons in the Strongly Interacting Regime,” Phys. Rev. Lett. 117, 055301 (2016).
- F. Chevy and C. Mora, “Ultra-Cold Polarized Fermi Gases,” Rep. Prog. Phys. 73, 112401 (2010).
- F. Ferlaino, A. Zenesini, M. Berninger, B. Huang, H.-C. Nägerl, and R. Grimm, “Efimov Resonances in Ultracold Quantum Gases,” Few-Body Syst. 51, 113 (2011).
- C. Kohstall, M. Zaccanti, M. Jag, A. Trenkwalder, P. Massignan, G. M. Bruun, F. Schreck, and R. Grimm, “Metastability and Coherence of Repulsive Polarons in a Strongly Interacting Fermi Mixture,” Nature 485, 615 (2012).