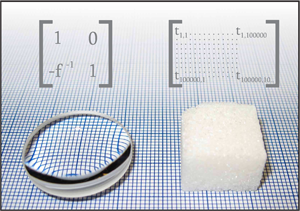
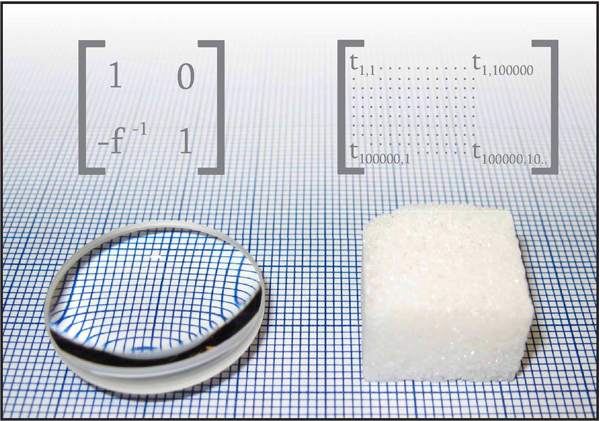
The information age in optics: Measuring the transmission matrix

Elbert G. van Putten; Allard P. Mosk

Viewpoint

The information age in optics: Measuring the transmission matrix

Complex Photonic Systems, Faculty of Science and Technology and MESA+ Institute for Nanotechnology, University of Twente, P.O. Box 217, 7500 AE Enschede, The Netherlands

March 8, 2010• Physics 3, 22

The transmission of light through a disordered medium is described in microscopic detail by a high-dimensional matrix. Researchers have now measured this transmission matrix directly, providing a new approach to control light propagation.

Figure caption — **Figure 1:** Two optical elements fully characterized by their transmission matrix, which relates the incident wave front to the transmitted one. In the case of a thin lens, the transformation of the wave front is described by a $2 \times 2$ matrix operating on a vector describing the wave front curvature [27]. For more complex elements such as a sugar cube the transmission matrix operates in a basis of transversal modes, which is very large. Full knowledge of the transmission matrix enables disordered materials to focus light as lenses.

Optical elements such as lenses and polarizers are used to modify the propagation of light. The transformations of the optical wave front that these elements perform are described by simple and straightforward transmission matrices (Fig. 1). The formalism of transmission matrices is also used to microscopically describe the transmission through more complex optical systems, including opaque materials such as a layer of paint in which light is strongly scattered. A microscopic description of this scattering process requires a transmission matrix with an enormous number of elements. Sébastien Popoff, Geoffroy Lerosey, Rémi Carminati, Mathias Fink, Claude Boccara, and Sylvain Gigan of the Institut Langevin in Paris now report in Physical Review Letters an experimental approach to microscopically measure the transmission matrix for light [1]. Knowledge of the transmission matrix promises a deeper understanding of the transport properties and enables precise control over light propagation through complex photonic systems.

At first sight, opaque disordered materials such as paper, paint, and biological tissue are completely different from lenses and other clear optical elements. In disordered materials all information in the wave front seems to be lost due to multiple scattering. The propagation of light in such materials is described very successfully by a diffusion approach in which one discards phase information and considers only the intensity. An important clue that phase information is very relevant in disordered systems was given by the observation of weak photon localization in diffusive samples [2,3]. Even extremely long light paths interfere constructively in the exact backscattering direction, an interference effect that can be observed in almost all multiple scattering systems. Interference in combination with very strong scattering will even bring diffusion to a halt when conditions are right for Anderson localization [4]. Since light waves do not lose their coherence properties even after thousands of scattering events, the transport of light through a disordered material is not dissipative at all, but coherent, with a high information capacity [5].

A propagating monochromatic light wave is characterized by the shape of its wave front. By choosing a suitable basis, the wave front incident on a sample can be decomposed into orthogonal modes. Typical choices for this basis of modes are the orthogonal modes of a waveguide or a basis of plane waves in free space. As only propagating waves need to be considered, the number of modes is finite and they form the basis in which the transmission matrix is written. The transmission matrix of the sample specifies the transmitted field amplitude for each combination of incident and transmitted modes. From a theoretical viewpoint, transmission matrices are useful tools to understand correlations in transport of light and other waves. Much insight into the properties of the transmission matrix has been gained in the framework of mesoscopic transport theory [6]. The transmission matrix has played a less important role in experiments due to its enormously high dimensionality: it is an $N \times$ $N$ matrix of complex numbers, where $N$ represents the number of modes of the incident (and transmitted) light field coupled to the sample. Each incident mode corresponds to a discrete incident angle, and the number of resolvable discrete angles is $N = 2 π$ $A / λ^{2}$ [7], with $A$ the illuminated surface area, $λ$ the wavelength, and where the factor $2$ accounts for two orthogonal polarizations. Hence, a $1$ - ${mm}^{2}$ sample has about a million transversal optical modes. Until recently, measuring a matrix with the corresponding large number of elements was beyond technological capabilities. Progress in digital imaging technology has now enabled measuring and handling such large amounts of data. In particular, spatial light modulators—computer-controlled elements that control the phase in each pixel of a two-dimensional wave front—are now creating a digital revolution in optics and are at the heart of the experiment by Popoff and colleagues.

In their experiment they used a spatial light modulator to precisely control the wave front of a monochromatic laser beam, which permitted them to address different incident modes of a strongly disordered sample. By cleverly using part of the transmitted light as a phase reference, they were able to capture amplitude and phase information on a two-dimensional CCD array of $16 \times 16$ pixels. Thanks to this parallel detection, they measured $16^{4}$ elements of the transmission matrix in only $16^{2}$ steps. Their method enables a deep characterization of light transport through turbid media, which enables them to control light propagation, as they demonstrated by transforming their sample into a focusing and detection element. To focus light they used the information in the transmission matrix to construct wave fronts that formed a tight focus after being scattered by the sample. Their approach is more flexible than first-generation “opaque lens” experiments [8] since the data to produce a focus at any desired position is already in the transmission matrix. To detect objects placed in front of the scattering sample they compared the transmitted field with the information stored in the transmission matrix.

Direct access to the individual elements of the matrix makes it possible to perform statistical analysis on them. The statistical properties of the transmission matrix are described using random matrix theory, an analytic approach that focuses on symmetries and conservation laws rather than detailed interactions (for an introduction see Ref. [9] and references therein). For example, the transmission matrix elements are correlated due to the fact that none of the matrix elements or singular values can ever be larger than unity, since in that case more than $100 %$ of the incident power would be transmitted [10]. However, this correlation is subtle and can only be observed if the complete transmission matrix is measured.

In the current experiments the number of measured matrix elements is impressive ( $65536$ ), yet the transmission matrix of the full area of the sample is even larger. Nevertheless, the matrix measured by Popoff et al. was sufficiently large to test an important baseline prediction of random matrix theory: The histogram of its singular values should have a peculiar quarter-circle shape [11,12]. The fact that the data follows this quarter-circle law means that the matrix elements are not significantly correlated, which is a good indication that the experimental procedure does not introduce spurious correlations. By measuring considerably larger matrices, intrinsic correlations can be brought to light. In a large enough matrix, the singular value distribution will deviate from the quarter-circle law and converge to a bimodal distribution consisting primarily of completely transmitting (open) and completely reflecting (closed) channels (for reviews, see Refs. [13–15]). Using the information in such a matrix it will be possible to create a perfect wave front that couples only to the open channels and is transmitted through an opaque medium for a full $100 %$ .

Another interesting experiment will be to measure the transmission matrix of samples with extreme disorder. As three-dimensional samples approach the Anderson localization threshold, the transmission matrices will give direct insight in the localized regime, where the modes of the transmitted light should have intriguing properties [13,16–19] . Similarly, it would be extremely interesting to study the transmission matrix of a so-called Lévy glass [20], in which light propagates according to a strongly modified diffusion law, or of photonic crystals, which have inevitable disorder [21] in addition to intricate band structure.

In relatively transparent materials, the transmission matrix can be used to obtain a tomographic reconstruction of the sample [22], which can be used to track processes inside living cells. It is not yet clear whether this approach can be generalized to stronger scattering materials, but it is hoped that information can be obtained from inside nontransparent biological tissue [23,24]. Algorithms to gain information on hidden targets from ultrasound measurements (see, e.g., Ref. [25]) could be ported to optics.

The approach of Popoff and colleagues marks the beginning of a highly exciting road towards a deeper understanding of light transport. Technological progress will enable the measurement of larger and larger matrices that contain all available information about the samples. Ongoing developments in random matrix analysis (see, e.g., Ref. [26]) will allow one to make sense of these enormous quantities of information. When the information in the transmission matrix is fully known, any disordered system becomes a high-quality optical element (Fig. 1). From a technological point of view this has great promise: quite possibly disordered scattering materials will soon become the nano-optical elements of choice.

References

S. M. Popoff, G. Lerosey, R. Carminati, M. Fink, A. C. Boccara, and S. Gigan, Phys. Rev. Lett. 104, 100601 (2010)
M. P. van Albada and A. Lagendijk, Phys. Rev. Lett. 55, 2692 (1985)
P. E. Wolf and G. Maret, Phys. Rev. Lett. 55, 2696 (1985)
A. Lagendijk, B. van Tiggelen, and D. S. Wiersma, Phys. Today 62, No. 8, 24 (2009)
S. E. Skipetrov, Phys. Rev. E 67, 036621 (2003)
C. W. J. Beenakker, Rev. Mod. Phys. 69, 731 (1997)
R. P. Feynman, R. B. Leighton, and M. Sands, The Feynman Lectures on Physics, Vol. 3 (Addison-Wesley, New York, 1965)[Amazon][WorldCat]
I. M. Vellekoop and A. P. Mosk, Opt. Lett. 32, 2309 (2007)
P. J. Forrester, N. C. Snaith, and J. J. M. Verbaarschot, J. Phys. A 36, R1 (2003)
J. Pendry, A. MacKinnon, and A. Pretre, Physica A 168, 400 (1990)
V. A. Marčenko and L. A. Pastur, Math. USSR-Sbornik 1, 457 (1967)
E. P. Wigner, SIAM Rev. 9, 1 (1967)
J. B. Pendry, Physics 1, 20 (2008)
J. Miller, Phys. Today 61, No. 9, 20 (2008)
C. W. J. Beenakker, arXiv:0904.1432 (2009)
C. M. Soukoulis and E. N. Economou, Phys. Rev. Lett. 52, 565 (1984)
A. A. Chabanov, M. Stoytchev, and A. Z. Genack, Nature 404, 6780 (2000)
M. Störzer, P. Gross, C. M. Aegerter, and G. Maret, Phys. Rev. Lett. 96, 063904 (2006)
S. Faez, A. Strybulevych, J. H. Page, A. Lagendijk, and B. A. van Tiggelen, Phys. Rev. Lett. 103, 155703 (2009)
P. Barthelemy, J. Bertolotti, and D. S. Wiersma, Nature 453, 495 (2008)
A. F. Koenderink, A. Lagendijk, and W. L. Vos, Phys. Rev. B 72, 153102 (2005)
W. Choi et al., Nat. Methods 4, 717 (2007)
Z. Yaqoob, D. Psaltis, M. S. Feld, and C. Yang, Nature Photon. 2, 110 (2008)
I. M. Vellekoop, E. G. van Putten, A. Lagendijk, and A. P. Mosk, Opt. Express 16, 67 (2008)
M. Fink, Phys. Today 50, No. 3, 34 (1997)
A. Edelman and N. Raj Rao, Acta Numerica 14, 233 (2005)
H. Kogelnik and T. Li, Proc. IEEE 54, 1312 (1966)

About the Authors

Elbert van Putten is working towards a Ph.D. in the Complex Photonics Systems group at the MESA+ Institute for Nanotechnology at the University of Twente in the Netherlands. His research is focused on gaining active control over light propagation in strongly disordered materials.

Allard Mosk is an associate professor in the Complex Photonics Systems group at the MESA+ Institute for Nanotechnology at the University of Twente in the Netherlands. He received his Ph.D. in 1999 from the University of Amsterdam, where he did experimental research on quantum gases. Currently he is working on methods to control both emission and propagation of light in complex nanophotonic environments. He is supported by a Vidi fellowship from the Netherlands Organization for Scientific Research (NWO).