Geometric phase kicks x rays down a new path

Bernhard W. Adams

Viewpoint

Geometric phase kicks x rays down a new path

Argonne National Laboratory, 9700 S. Cass Avenue, Lemont, IL 60564, USA

June 14, 2010• Physics 3, 50

A small crystal distortion can dramatically translate the path of an x-ray beam, an effect that could be useful in the development of x-ray optics.

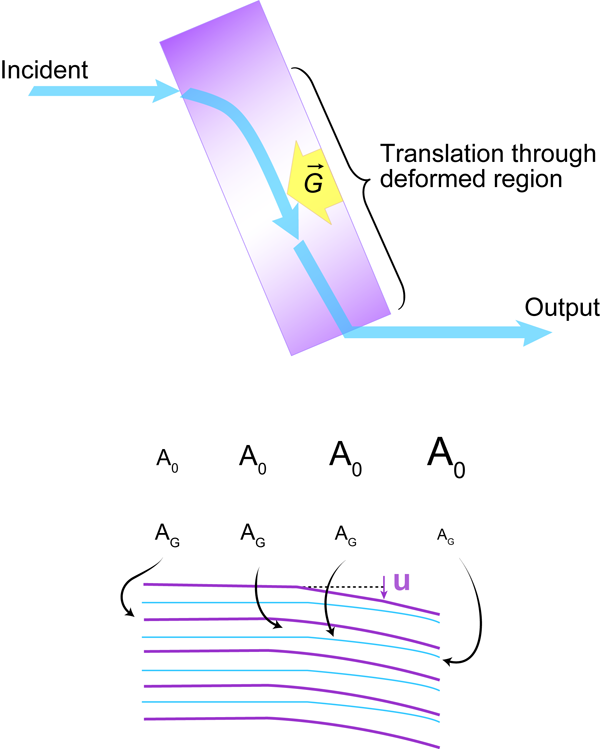
Figure caption — **Figure 1:** (Top) A small deformation of the lattice planes in a perfect crystal has a wave-guiding effect on an x-ray wave packet, effectively translating it by several mm through the crystal ( $G$ is a reciprocal lattice vector). (Bottom) A more detailed picture of how the curved lattice planes translate the x-ray “ $α$ mode” that propagates through the crystal. The purple lines indicate the lattice planes ( $u$ denotes the displacement as a function of space) and the blue lines denote the phase of the propagating $α$ mode. As the $α$ mode curves, the amplitudes of its constituent $A_{0}$ and $A_{G}$ waves changes [6], with the amplitude of $A_{0}$ increasing with respect to $A_{G}$ . Since the Poynting vector is parallel to ${| A_{0} |}^{2} k_{0} + {| A_{G} |}^{2} (k_{0} + G)$ , the exiting wave is mainly along $k_{0}$ .

The first impression one gets walking into an optics lab is of the endless maze of mirrors, lenses, etc., that can bend and guide the light along practically any desired path. Controlling x rays in the same manner, however, is much harder. In a paper appearing in Physical Review Letters [1],Yoshiki Kohmura, Kei Sawada, and Tetsuya Ishikawa at the SPring-8 synchrotron in Hyogo, Japan, show they can displace an x-ray beam propagating inside a crystal by a drastic amount—of order a millimeter—by deforming a single crystal less than 100 nanometers (Fig. 1). They argue that the displacement arises from a geometric, or Berry, phase picked up by the waves constituting the x ray, an effect predicted several years ago [2]. Experimentally, much remains to be done towards a full demonstration of the geometric-phase effect on the beam propagation. This statement is not meant to be derogatory—the experiments are challenging—but rather to stimulate further work in precision x-ray optics and, at the same time, investigate further what could be a toy model for many other fields of physics.

Berry phases appear in many contexts of physics, including quantum systems, classical oscillators, and classical wave-propagating media. In the 1980s, Michael Berry observed that a quantum system, which is described by multiple parameters, picks up a phase beyond that of its dynamical evolution if the parameters are changed slowly along a closed loop in the parameter space (where each axis represents one parameter). This additional phase depends only on the geometrical properties of the loop. In particular, the Berry phase does not depend on the time it takes to traverse the loop, as long as it is done sufficiently slowly (adiabatically). The classic example is the polarization of a light beam as it is transmitted through a twisted optical fiber.

Geometric phases are closely related to another ubiquitous concept—that of avoided crossings (see Refs. [3,4] for examples). The term “avoided crossings” refers to energy levels in spectroscopy that rise or fall under the influence of an external field, but the concept applies generally to interacting parameter-dependent oscillatory modes, or wave modes in a parameter-dependent medium. As parameters are varied, and two modes become similar (say, they approach the same frequency), oscillatory averaging of the interaction no longer applies. Because the modes are coupled, they can no longer be degenerate and they repel each other by splitting into two coupled-wave modes with oppositely phased contributions from the original modes.

Avoided crossings occur for x rays and matter waves in a perfect crystal, which is what underlies the effect seen by Kohmura et al. An x-ray wave $A_{0} (r) = A_{0} exp (i k \cdot r)$ with an arbitrary wave vector $k_{0}$ propagating in a crystal will be scattered with little overall effect. But near the Bragg condition, that is when $| k_{0} + G | \approx | k_{0} |$ for one of the crystal’s reciprocal lattice vectors $G$ , the scattered waves add constructively to a sizeable amplitude $A_{G} (r) = A_{G} exp (i (k_{0} + G) \cdot r)$ . The two waves $A_{0} (r)$ and $A_{G} (r)$ form a standing-wave pattern that has the same periodicity as the lattice. In a perfect crystal $A_{G}$ is coherent with $A_{0}$ and scatters back into it, and vice versa over a characteristic propagation length called the pendellösung length that varies inversely with the strength of the scattering coupling the waves (pendellösung is German for “pendulum solution” because the problem is analogous to two coupled pendulums). For this case, it is much simpler to consider the propagation of the coupled-wave modes and their dispersion, rather than treating each of them individually.

Close to the Bragg condition there is an avoided crossing in momentum space that splits the coupled-wave modes into two bands separated by a gap [5]: of these, modes on the so-called $α$ branch have antinodes between the lattice planes, and thus experience less-than-average scattering. The opposite is true for the other branch, containing the $β$ modes. Because, differently from light in glass, etc., x rays in matter experience a refractive index of slightly less than 1, an $α$ mode has a higher-than-average refractive index (while the $β$ mode is lower than average). Which of these two modes (both, only one, or none) are excited by a wave incident on the crystal depends on the boundary conditions with the usual provision that wave vectors must be coupled along the surface normal vector. If no propagating mode is excited, total reflection occurs, also known as Bragg-case reflection. As the angle of incidence is changed from Bragg-case reflection towards smaller incidence angles, the $α$ mode is excited. More precisely, an actual x-ray beam will excite many closely spaced modes on the $α$ branch.

These concepts pertain to a perfect crystal. A detailed explanation of how the x-ray modes evolve in a deformed crystal involves some equation-gazing (see Ref. [6] for detail), but the gist of it is this: Due to its anomalously high refractive index, an $α$ mode is stable to perturbations, similarly to light guided by the high-index core of a glass fiber. By the same reasoning, a $β$ mode is unstable, and quickly leaves the scene. Provided it is not too large, the crystal deformation will guide the standing-wave pattern of the $α$ modes constituting a beam along the curved lattice planes. This has, however, the effect of strongly changing the amplitude ratio of the constituent coupled waves (Fig. 1, bottom). Since the beam direction is given by the Poynting vector, which is parallel to ${| A_{0} |}^{2} k_{0} + {| A_{G} |}^{2} (k_{0} + G)$ , the amplitude change leads to a change in beam direction, that is, beam bending that is much stronger than the curvature of the lattice planes.

In their experiment, Kohmura et al. oxidized a 100-micron-thick silicon crystal on a wax backing to induce a deformation of 80 nanometers or more. When the incident x-ray beam direction was detuned from the Bragg condition by $Δ Θ = - 2$ arcsec, they observed a transmitted beam that was parallel to the original beam, but displaced by about 1.5 mm from its original trajectory. This can be explained by a curved ray inside the crystal that splits into the constituent waves $k_{0}, k_{0} + G$ upon exiting (Kohmura et al. report only the $k_{0}$ wave).

One may now ask where in the experiment the Berry phase has become evident. The answer is that it only does so indirectly. The observation is a beam displacement, which is evidence of beam bending inside the crystal. This, in turn, suggests a Berry curvature, which, according to the theory of Ref. [2], is related to the Berry phase as a gauge field [7–9]. To actually measure a phase, one would have to insert a sample crystal into an interferometer, similarly to, but much more complex than, experiments on forward diffraction in unstrained crystals [10,11]. To demonstrate the geometric-phase nature, one would also have to demonstrate that different strain-field profiles leading to the same crystal displacement will yield the same beam displacement. This is, of course, easier said than done.

Synchrotron-radiation optics and brilliant sources, such as x-ray free-electron lasers have already come a long way, and will go much further in the next few years. Therefore, the demonstration of Kohmura et al. of an x-ray-bending effect similar to that in visible-light optics is possibly of both fundamental and practical importance.

References

Y. Kohmura, K. Sawada, and T. Ishikawa, Phys. Rev. Lett. 104, 244801 (2010)
K. Sawada et al., Phys. Rev. Lett. 96, 154802 (2006)
S. Oh, Z. Huang, U. Peskin, and S. Kais, Phys. Rev. A 78, 062108 (2008)
W. Wernsdorfer, M. Soler, G. Christou, and D. Hendrickson, J. Appl. Phys. 91, 7164 (2002)
A. Authier, Dynamical Theory of X-Ray Diffraction (Oxford University Press, Oxford, 2001)[Amazon][WorldCat]
B. Adams, Acta Crystallogr. Sect. A 60, 120 (2004)
M. Kohmoto, J. Phys. Soc. Jpn. 62, 659 (1993)
M. Berry, Proc. R. Soc. London A 392, 45 (1984)
B. R. Holstein, Am. J. Phys. 57, 1079 (1989)
K. Hirano and A. Momose, Phys. Rev. Lett. 76, 3735 (1996)
B. Adams (unpublished)

About the Author

Bernhard Adams received his diploma in x-ray interferometery from the University of Munich. Following his Ph.D. at the University of Munich on the influence of a surface on phase-transition kinetics, he completed post-doctoral work at DESY in Hamburg, studying atom-resolving x-ray holography and parametric down conversion of x rays. He is currently a staff scientist at Argonne National Laboratory, where his main scientific interests include ultrafast (picosecond) x-ray spectroscopy and x-ray quantum optics, such as x-ray parametric down conversion, nuclear $γ$ -ray superradiance, and the Casimir force.