Friction’s Role in Shear Thickening
Concentrated suspensions of hard particles in liquid—most famously, cornstarch in water—respond differently than normal liquids to shear forces. Vigorously stirring or impacting these fluids can dramatically increase their resistance to flow and make them feel like solids. What has not been known is how interactions between the particles in suspension lead to this behavior, called discontinuous shear thickening (DST). And so far, simulations haven’t been able to predict the jump in viscosity with shear rate that is characteristic of these systems or how this transition depends on the concentration of particles. Now, Ryohei Seto and colleagues at City College in New York report in Physical Review Letters that they have, for the first time, accurately reproduced the DST transition with numerical simulations by including direct friction between the solid particles [1]. Their model could be used to help industries develop better ways of processing suspensions like slurries and inks.
Fluids are characterized by their viscosity, which is a measure of the force required to make the fluid flow at a given shear rate. DST suspensions are examples of “non-Newtonian” fluids because their viscosity varies with shear rate. At a critical shear rate, the viscosity in a DST fluid jumps—in some cases, by several orders of magnitude—and solidlike behavior sets in [2]. This jump looks like a second-order phase transition: as a function of shear rate, it becomes steeper when the concentration of particles, called the packing fraction, increases, and becomes discontinuous at a critical packing fraction [3]. The DST transition can produce a fluid so resistant to flow that a person could run on it or bounce a bowling ball off its surface (see Video 1). The transition is also completely reversible: once the shear is removed, the suspension relaxes and behaves like a normal liquid.
Until recently, attempts to model DST assumed the jump in viscosity came from an increase in viscous drag forces from the liquid between the particles [4,5]. (Higher drag forces can occur when particles rearrange and the gaps between them are smaller.) But these hydrodynamic models weren’t able to accurately reproduce DST in simulations: the viscous drag turns out to be too weak to explain the strongest shear thickening effects seen in experiments [2].
Seto et al.’s work builds on a relatively new picture in which the dominant force in DST is solid friction between the particles. Three key insights have led to this view. The first is the similarity between DST and the physics of jamming—a phase transition from a liquidlike to a solidlike state that can occur in emulsions, foams, and other particle systems when the packing fraction is increased [6]. In jammed systems, the particle packing structure becomes rigid because of the formation of irregular networks of particles in contact that span the entire system and are capable of withstanding high stress forces. Since the jamming and DST transitions have the same critical packing fraction for a given suspension (the value is around for nearly spherical particles [3]), it’s reasonable to expect that rigid particle networks may also underlie the DST transition.
The second important insight into DST was that particle contact networks can dynamically form and break up because of what is called “dilatancy,” which is the tendency of granular materials and sufficiently concentrated suspensions to expand under shear. Dilation causes the particle networks to press against the boundaries, which then in turn support the networks. The force that can be supported is then only limited by the stiffness of the particles or the boundary, whichever is weakest [7]. Finally, experiments have shown that the main force between the particles is frictional rather than viscous drag [8].
An anology helps to explain why jammed contact networks lead to a solidlike response in a DST suspension. Imagine walking on a pile of blocks that are stacked from the bottom of a pool of water to the top. This pile of blocks transmits the force of your weight to the bottom boundary of the pool and is capable of holding you up, thus allowing you to walk across. If, instead, the blocks were only floating and not piled all the way to the bottom, you would sink when you stepped on the blocks.
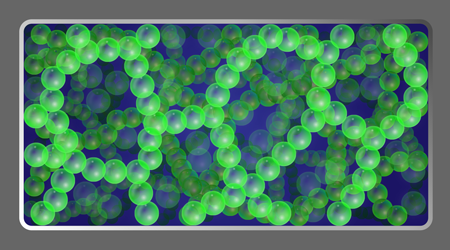
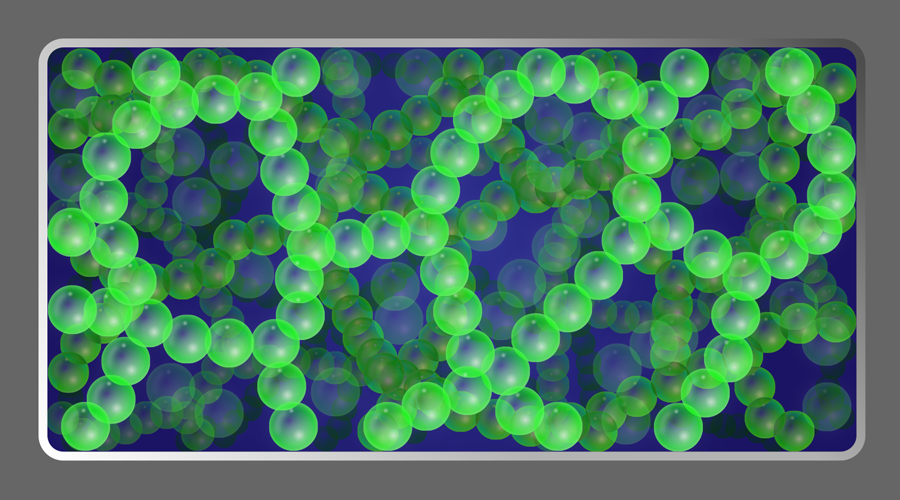
Experiments can, however, only indirectly infer the existence of frictional contacts and force chains in a shear thickened suspension—the contacts networks are too hard to see in three dimensions. Seto et al.’s simulations of the DST transition reveal these features clearly. The basis of their simulations is a model of ideal hard spheres in suspension, in which neighboring particles interact by both viscous drag and friction. They assume the viscous drag between neighboring particles is proportional to shear rate and diverges as the size of the liquid-filled gap between neighboring particles goes to zero—a standard assumption in suspension rheology [4]. The new feature in their model is the existence of a cutoff value for the gap size: below this cutoff, the contacts become frictional instead of viscous. Physically, this cutoff may represent gaps that are only a few molecules thick, since on this scale, the liquid between the particles can no longer be considered a continuous medium, and hydrodynamic models break down [2].
With this model, Seto et al. are able to accurately reproduce experimental observations of the steep viscosity versus shear rate curves and their evolution with packing fraction, both of which are firsts for numerical simulations. They confirm that DST is due to friction by showing that when the friction coefficient is reduced to , the shear thickening behavior completely disappears, which is in line with what other recent simulations have found [9,10]. They also show the first simulated images of evolving frictional contact networks in the shear-thickening regime. These contact networks become larger as the shear rate and packing fraction increase and eventually fill the system once the solidlike frictional regime is reached (see Fig. 1).
The availability of an accurate model of DST has several applications. Industries that handle suspensions would benefit from knowing under what conditions these fluids become solidlike, as the change can damage mixer blades and other machinery. Seto et al.’s model may also help answer some open questions. In particular, why is there a transition from liquidlike to solidlike behavior at a critical shear rate, which is unusual given that most liquid-solid phase transitions don’t depend on speed. Granular and even suspension models of dilatancy do not predict any such transition at a critical shear rate or corresponding timescale (the units of shear rate are the inverse of a timescale). While hydrodynamic models predict a critical shear rate [4], it is still unclear if they correctly explain this transition, and they fail to be applicable once particles come in contact and the physics becomes interesting. Perhaps only the marriage of hydrodynamic and granular flow models, as in Seto et al.’s simulations, will be able to explain this transition.
References
- R. Seto, R. Mari, J. F. Morris, and M. M. Denn, “Discontinuous Shear Thickening of Frictional Hard-Sphere Suspensions,” Phys. Rev. Lett. 111, 218301 (2013)
- E. Brown and H. Jaeger, “Shear Thickening in Concentrated Suspensions: Phenomenology, Mechanisms, and Relations to Jamming,” arXiv:1307.0269 (2013)
- E. Brown and H. M. Jaeger, “Dynamic Jamming Point for Shear Thickening Suspensions,” Phys. Rev. Lett. 103, 086001 (2009)
- J. Brady and G. Bossis, “The Rheology of Concentrated Suspensions of Spheres in Simple Shear Flow by Numerical Simulation,” J. Fluid Mech. 155, 105 (1985)
- N. Wagner and J. Brady, “Shear Thickening in Colloidal Dispersions,” Phys. Today 62, No. 10, 27 (2009)
- M. Cates, J. Wittmer, J.-P. Bouchaud, and P. Claudin, “Jamming, Force Chains, and Fragile Matter,” Phys. Rev. Lett. 81, 1841 (1998)
- E. Brown and H. Jaeger, “The Role of Dilation and Confining Stresses in Shear Thickening of Dense Suspensions,” J. Rheol. 56, 875 (2012)
- D. Lootens, H. V. Damme, and P. Hébraud, “Giant Stress Fluctuations at the Jamming Transition,” Phys. Rev. Lett. 90, 178301 (2003)
- C. Heussinger, “Shear Thickening in Granular Suspensions: Inter-particle Friction and Dynamically Correlated Clusters,” arXiv:1307.2133 (2013); Phys. Rev. E (to be published)
- N. Fernandez, R. Mani, D. Rinaldi, D. Kadau, M. Mosquet, H. Lombois-Burger, J. Cayer-Barrioz, H. J. Herrmann, N. D. Spencer, and L. Isa, ”Microscopic Mechanism for Shear Thickening of Non-Brownian Suspensions,” Phys. Rev. Lett. 111, 108301 (2013)