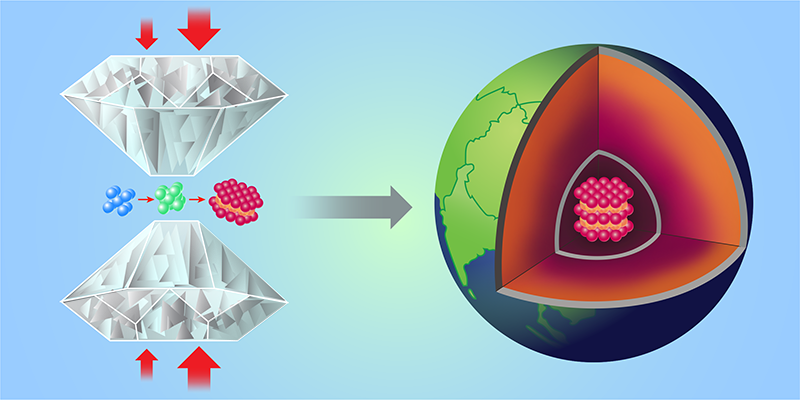
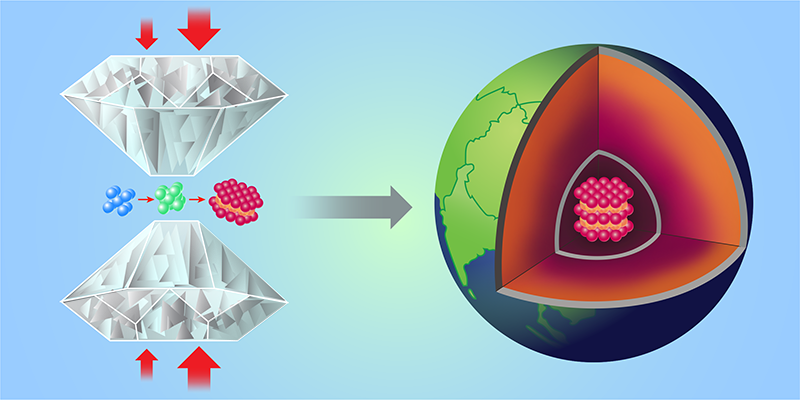
Measuring the Elasticity of Pressurized Iron

Shanti Deemyad

Viewpoint

Measuring the Elasticity of Pressurized Iron

Department of Physics and Astronomy, University of Utah, Salt Lake City, UT, US

July 17, 2023• Physics 16, 109

Laboratory experiments elucidate the directions and speeds at which acoustic waves propagate in the type of iron that likely makes up Earth’s core.

Figure caption — **Figure 1:** By compressing a specific crystalline orientation of iron in a diamond-anvil cell, researchers have for the first time created a version of the metal in the structure that it likely takes in Earth’s core.

By exploring uncharted pathways within the pressure–temperature phase space, scientists have achieved a groundbreaking milestone: the synthesis of single-crystalline iron in the structure that it likely assumes in Earth’s core [1]. This accomplishment allows for precise measurements of the elastic properties of iron in various crystalline directions. Additionally, the study helps to identify a theoretical approach that could uncover the underlying mechanisms responsible for the observed anisotropy in seismic-wave propagation throughout Earth. By elucidating the properties of iron in its core structure, this research takes us one step closer to unravelling the secrets of our planet’s innermost regions.

Our understanding of Earth’s composition and structure relies on seismological studies, which analyze how elastic waves propagate through the planet. These studies require knowledge of material properties at relevant densities. The current model of Earth’s interior is based on an analysis by Sir Harold Jeffreys and Inge Lehmann, who proposed that Earth’s core consists of a solid inner core surrounded by a liquid outer core [2, 3]. In the 1980s, researchers discovered that seismic waves exhibit anisotropic behavior, traveling faster in the polar direction than in the equatorial direction [4]. One popular explanation for this phenomenon assumes that the solid inner core is predominantly composed of iron in a hexagonal close-packed structure known as $𝜀$ -iron [5–7]. This material consists of crystals with preferred orientations that together cause sound waves to propagate differently along different directions [8].

Iron has been extensively studied under high pressure because of its abundance in Earth’s core [9]. Even so, there has been a crucial lack of experimental data on the elastic properties of $𝜀$ -iron along different crystalline orientations. Determining the elastic properties of anisotropic solids requires measuring the elasticity tensor, which represents the linear relationship between stress and strain in a material and characterizes the speed of sound propagation in different crystalline orientations. However, measuring the elasticity tensor under pressure is challenging and requires synchrotron x-ray techniques performed on high-quality single crystals.

Unfortunately, when iron is compressed from its initial body-centered cubic crystalline phase ( $𝛼$ -phase) to $𝜀$ -iron, the samples typically fracture into numerous small crystals that undergo plastic deformation. Their tiny size renders them unsuitable for detailed crystallographic analysis and has been a major hindrance in accurately determining the anisotropy in the elastic properties of $𝜀$ -iron.

Agnès Dewaele of the University of Paris-Saclay and her colleagues successfully met this challenge [1]. They employed an innovative experimental approach, taking an alternative pathway in the iron phase diagram to synthesize pure single-crystalline $𝜀$ -iron. Instead of pressurizing the $𝛼$ -phase on an isothermal path, the researchers heated the sample while still in the $𝛼$ -phase on an isobaric, or constant pressure, path to reach the face-centered cubic phase of iron ( $𝛾$ -phase). They then transitioned to $𝜀$ -iron through isothermal pressurization of the $𝛾$ -phase followed by an isobaric cooling. Lastly, using inelastic x-ray scattering, they measured the elastic constants of $𝜀$ -iron along different crystalline directions.

Unlike previous studies that relied on powdered iron samples, Dewaele and colleagues’ findings provide precise estimations of the anisotropy present in the elastic constants of $𝜀$ -iron. The results of this study qualitatively agree with prior works in identifying the direction at which waves propagate the most rapidly through $𝜀$ -iron’s structure—the material’s fast axis [10]. But they quantitatively show significant deviations from previously obtained data, highlighting the importance of their experimental approach and its impact on our understanding of $𝜀$ -iron’s properties.

The study directly verifies that longitudinal waves propagate faster along the lines that connect $𝜀$ -iron lattice nodes in an orientation known as the c-direction, and at a velocity that is approximately 4.4% higher than waves traveling in the lattice’s basal plane. Furthermore, the research successfully demonstrates the pressure dependence of changes in the elastic properties of $𝜀$ -iron, suggesting that these trends persist during pressurization. It’s important to note that the experiments in this study were performed at room temperature and were limited to pressures up to 30 GPa, which is an order of magnitude lower than conditions in Earth’s core. However, the experimental data obtained provides a crucial test for theoretical models.

The experimental data not only allow the researchers to identify the most appropriate theoretical approach—one with superior predictive power for calculating the elasticity tensor of $𝜀$ -iron—but also enable them to extend this knowledge to conditions resembling those within Earth’s core. In particular, Dewaele and her colleagues show how the observed anisotropy could persist at a consistent magnitude from lower pressures to the extreme densities characteristic of Earth’s inner core.

So long as we cannot physically access Earth’s core, laboratory-based measurements of material properties under extreme conditions are crucial for ensuring the accuracy of our models. This research brings us closer to realizing the long-standing aspiration of a virtual “journey to the center of Earth.” The study not only opens new doors for understanding Earth’s core but also exemplifies the power of combining experimentation and theory in pushing the boundaries of scientific understanding.

References

A. Dewaele et al., “Synthesis of single crystals of $𝜖$ -iron and direct measurements of its elastic constants,” Phys. Rev, Lett. 131, 034101 (2023).
H. Jeffreys, The Earth (Cambridge University Press, New York, 1929), p. 265.
I. Lehmann, “P´,” Bur. Central Seismol. Int. Ser. A 14, 3 (1936).
S. Tateno et al., “The structure of iron in Earth’s inner core,” Science 330, 359 (2010).
B. Buffett, “Earth’s enigmatic inner core,” Phys. Today 66, 37 (2013).
A. Morelli et al., “Anisotropy of the inner core inferred from PKIKP travel times,” Geophys. Res. Lett. 13, 1545 (1986).
H. K. Mao et al., “Static compression of iron to 300 GPa and Fe_0.8Ni_0.2 alloy to 260 GPa: Implications for composition of the core,” J. Geophys. Res.: Solid Earth 95, 21737 (1990).
A. Deuss, “Heterogeneity and anisotropy of Earth’s inner core,” Annu. Rev. Earth Planet. Sci. 42, 103 (2014).
F. Birch, “Density and composition of mantle and core,” J. Geophys. Res. 69, 4377 (1964).
W. L. Mao et al., “Experimental determination of the elasticity of iron at high pressure,” J. Geophys. Res.: Solid Earth 113, 89 (2008).

About the Author

Shanti Deemyad is an experimental condensed-matter physicist currently serving as an associate professor of physics and head of the high-pressure research laboratory at the University of Utah. Deemyad completed her undergraduate studies at Sharif University of Technology, Iran, and went on to earn a PhD in physics from Washington University in St. Louis. Following the completion of her doctoral studies, she conducted postdoctoral research at Harvard University. Deemyad’s research is centered around the exploration of quantum effects in lattice and electronic properties of condensed-matter systems. She is particularly interested in investigating exotic states of matter that emerge under extreme conditions of pressure and temperature.