Active Particles Push the Boundaries of Two-Dimensional Solids
If you compress a liquid slowly enough at low temperatures, it will freeze into an ordered solid: a crystal. Or at least that’s what we’re used to seeing in three dimensions. If you instead consider particles confined to a two-dimensional (2D) plane, the outcome is quite different. For equilibrium systems, a 2D solid stabilizes into a structure that lacks long-range order—it becomes less ordered further away from a central lattice site. The behavior of systems far from equilibrium, such as self-propelled particles, remains, however, an open question. In a numerical study of bacteria-like particles, Xia-qing Shi of Soochow University in China and his colleagues now show that active crystals follow a slightly different set of rules than their nonmotile counterparts [1]. Like 2D equilibrium crystals, 2D active systems stabilize into an ordered solid-like phase but with extremely large particle fluctuations around the configuration of a perfect crystal lattice. The finding could help to guide future materials design based on active particles.
A defining feature of crystal structures is their periodicity. They exhibit long-range order, meaning a regular arrangement of particles that repeats periodically over the entire crystal. In equilibrium, 2D solids cannot display true long-range order [2]. Instead, the correlation function of particle position decays with distance, according to a power law that is captured by the famous Berezinskii-Kosterlitz-Thouless-Halperin-Nelson-Young (BKTHNY) theory [3]. Interestingly, despite their lack of long-range positional order, these 2D solids still feature true long-range orientational order: the particles are perfectly aligned throughout the system. For any system that breaks time-reversal symmetry, however, established theories cannot say whether true 2D crystals can be stabilized at all, much less what this stabilization would look like. This theoretical challenge is particularly relevant to active matter systems (those that transform energy into mechanical work at microscopic scales, such as collections of bacteria or chemically propelled colloidal particles) when they shift from a fluid to a dense, solid-like phase [4–7].
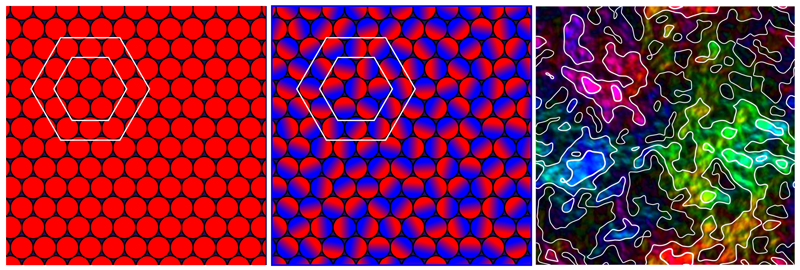
Shi and team now show, numerically and analytically, how positional and orientational order play out in a 2D active system. Their study examines a common toy model of active matter, a system of mutually repulsive particles. The particles self-propel along a direction defined by their internal polar axes, which align on the short range with the axes of neighbors but also fluctuate. The researchers first showed that this system exhibits a dense, ordered phase, much like an equilibrium solid, for sufficiently small self-propulsion speed, alignment degree, and rotational diffusion. Then, they focused their attention on how this dense phase changes as one tunes various system parameters.
The team numerically demonstrated that the system displays quasi-long-range positional order and true long-range orientational order throughout the volume, much like an equilibrium solid. However, the twist is that the power-law exponents describing the positional order cover a very wide range, predicting values that reach as high as 20, which may sound shockingly large to someone accustomed to equilibrium physics. Not only does the usual BKTHNY theory establish an upper bound of 1/3 for the power-law exponent in 2D equilibrium solids [3], but also it is highly unusual to see a critical exponent taking such large values in general. Indeed, typical critical exponents for correlation length versus temperature or magnetic order versus temperature are of order 1.
To back up their numerical observations, Shi and his colleagues devised a simple linear elastic theory describing the displacement of particles with respect to a perfectly ordered lattice. To do so, they replaced the equilibrium term that describes fluctuations as white noise (whose variance is linked to temperature) with an active term that describes those fluctuations in terms of varying self-propulsion directions. This leads to the definition of an “effective active temperature” that replaces the one used in the BKTHNY power-law expression, allowing the accepted theory to escape equilibrium limitations. This effective temperature also helps to explain an intriguing feature of 2D active solids: their particle positions display very large spontaneous fluctuations about the positions they’d have in a perfect crystal. These gigantic spontaneous deformations are much larger than those occurring in any equilibrium setting. But, as in a 2D equilibrium crystal, the particles retain true long-range orientational order.
Shi’s study highlights how being far from equilibrium allows systems to break rules that we often take for granted. At the fundamental level, the use of a minimal elastic theory and of an effective temperature elegantly connects active systems to equilibrium ones in a way reminiscent of effective temperatures in glasses [8]. Beyond toy models, this concept could help to explain the behavior of dense, confined biological systems like tissues [9].
On more practical grounds, a better understanding of the interplay between order and fluctuations in active solids is necessary to start integrating active elements into materials and fabrication processes. For example, macroscopic mechanical metamaterials could use active elements to intermittently generate larger amplitudes of deformations without affecting the material’s integrity [10]. Likewise, in the context of colloidal materials [11], introducing activity at crucial steps during preparation could facilitate the self-assembly of a broader variety of structures.
References
- X.-q. Shi et al., “Extreme spontaneous deformations of active crystals,” Phys. Rev. Lett. 131, 108301 (2023).
- N. D. Mermin, “Crystalline order in two dimensions,” Phys. Rev. 176, 250 (1968).
- R. R. Nelson and B. I. Halperin, “Dislocation-mediated melting in two dimensions,” Phys. Rev. B 19, 2457 (1979); A. P. Young, “Melting and the vector coulomb gas in two dimensions,” 19, 1855 (1979).
- G. Briand and O. Dauchot, “Crystallization of self-propelled hard discs,” Phys. Rev. Lett. 117, 098004 (2016).
- L. F. Cugliandolo and G. Gonnella, “Phases of active matter in two dimensions,” in Active Matter And Non-Equilibrium Statistical Physics, Lecture Notes of the Les Houches Summer School, edited by J. Tailleur et al. (Oxford University Press, Oxford, 2022)[Amazon][WorldCat].
- L. Caprini et al., “Entropons as collective excitations in active solids,” J. Chem. Phys. 159 (2023).
- P. Baconnier et al., “Selective and collective actuation in active solids,” Nat. Phys. 18, 1234 (2022).
- L. F. Cugliandolo, “The effective temperature,” J. Phys. 44, 483001 (2011).
- S. Kim et al., “Embryonic tissues as active foams,” Nat. Phys. 17, 859 (2021).
- J. U. Surjadi et al., “Mechanical metamaterials and their engineering applications,” Adv. Eng. Mater. 21, 1800864 (2019).
- M. He et al., “Colloidal diamond,” Nature 585, 524 (2020).