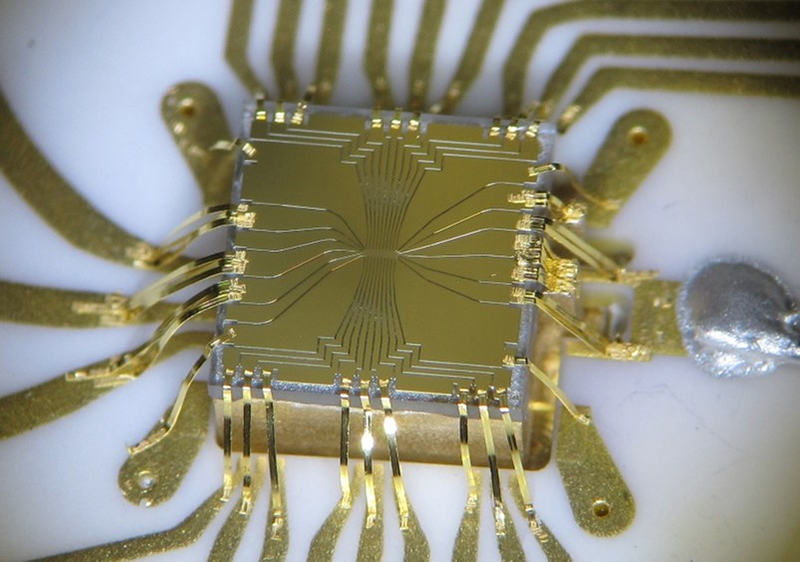
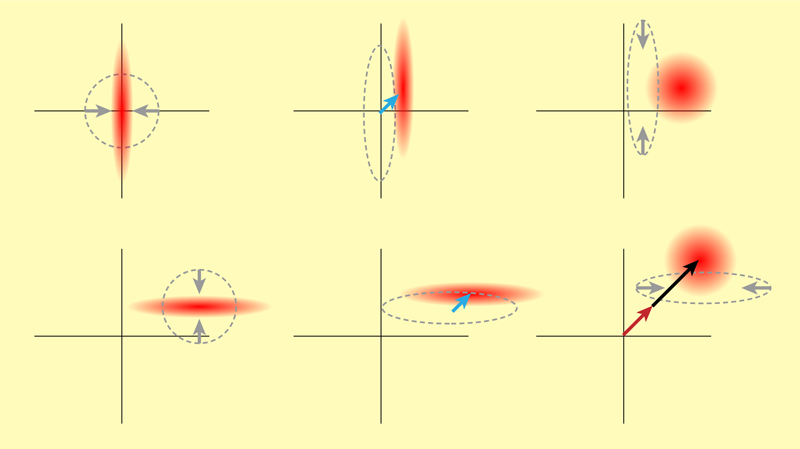
Enhanced Interactions Using Quantum Squeezing
Squeezed states are an important class of nonclassical states, where quantum fluctuations can be reduced in one property of a system, such as position. However, at the same time, according to the Heisenberg uncertainty principle, quantum fluctuations increase in the conjugate property, in this case momentum. The ability to suppress noise in at least one variable is valuable in a wide range of areas in quantum technologies. Now Shaun Burd at the National Institute of Standards and Technology, Colorado, and colleagues have experimentally demonstrated a squeezing-based enhancement method that requires no preknowledge of the system’s parameters [1]. The researchers use a trapped-ion system (Fig. 1) and show that they can amplify the motion of the ion using a combination of squeezing procedures. This experimental research can stimulate other novel applications of squeezing, for example, in dark matter searches.
For decades, quantum squeezing has played a central role in high-precision quantum measurements, such as gravitational-wave detection [2, 3] and nondemolition qubit readout [4–6]. The methods typically involve applying a field or inserting an optical element that reduces the fluctuations in one observable. The measurements of this squeezed observable can beat the standard quantum limit and thus enable a significant improvement in the detection sensitivity or the readout signal-to-noise ratio.
In addition to the reduced fluctuations in the squeezed observable, the amplified fluctuations in the antisqueezed observable have also attracted intense interest as a way to enhance a particular interaction [7]. Typically, the interaction involves a quantum harmonics oscillator, and the squeezed and antisqueezed observables are the orthogonal components, or “quadratures,” of the oscillator’s motion. A representative example is the optomechanical interaction between a mass on a spring and a light field, with the position and momentum of the mass being the two quadratures. The strength of that interaction depends on the amplitude of the quantum fluctuations in the oscillator’s motion. It is therefore possible to significantly or even exponentially enhance the interaction strength by squeezing one quadrature while correspondingly antisqueezing the other quadrature.
Recent experiments have demonstrated this squeezing-based enhancement using trapped ions and superconducting circuits [8, 9]. However, precise knowledge of the system parameters is usually required to reach optimal results. For example, in one of the experiments [8], it was shown that the phase difference between the squeezing operation and the rest of the system dynamics must be chosen properly; otherwise, the desired interaction becomes reduced rather than enhanced.
This preknowledge requirement poses a problem in cases where the system parameters may be unknown in advance or vary with time. To address this issue, an approach called Hamiltonian amplification was proposed [10]. The key idea is that the time evolution of the system is divided into small steps, each amplified individually by the squeezing and antisqueezing operations. In this case, the system Hamiltonian can be amplified in the absence of precise knowledge of the system parameters.
Burd and colleagues report an experimental implementation of this Hamiltonian amplification using a trapped-ion system. The experiment uses a radial mode of motion of a single magnesium ion (25Mg+) as a quantum harmonic oscillator, with the ion being trapped approximately 30 µm above an electrode plane. As a first demonstration, the researchers moved the ion from one location to another. Typically, such displacement can be done by measuring the phase of the ion’s oscillations and then timing when to apply a push in the desired direction. However, the researchers showed that they can perform a phase-insensitive displacement using a sequence of squeezing operations—where each operation involves a time variation of the electrode potential at twice the motional frequency of the ion. The team divided the displacement into two steps. In each step, the displacement push is sandwiched between squeezing and antisqueezing operations (Fig. 2). The resulting displacement is enhanced by a factor of 2 relative to the displacement of the ion without squeezing.
The researchers also demonstrated a phase-insensitive amplification of an interaction (called a Jaynes-Cummings coupling) between a qubit and an external degree of freedom. They first established a two-level qubit state in the 2S1/2 electronic ground-state hyperfine manifold of the 25Mg+ ion, and they coupled this qubit to the ion’s motion via so-called motional sideband transitions. In order to enhance the qubit–motion coupling in a phase-insensitive manner, Burd and colleagues performed a time-slicing technique called Trotterization, which they combined with their squeezing protocols. The researchers measured the strength of the qubit–motion coupling by observing the Rabi oscillations between the two ion levels, and they found that squeezing can increase the coupling strength by a factor of about 1.5, relative to the case without squeezing.
Burd and colleagues used a trapped-ion system, but the proposed Hamiltonian-amplification procedures are also suitable for other physical systems, such as superconducting circuits and microwave cavities. Applications that might benefit from Hamiltonian amplification include quantum computation and dark matter detection. For example, this technique may be helpful in dark matter axion searches by amplifying the predicted interaction between axions and photons in a microwave cavity. Another interesting future direction to explore is whether Hamiltonian amplification might help mitigate the problem of single-photon loss, which is the main source of noise in many quantum systems.
References
- S. C. Burd et al., “Experimental speedup of quantum dynamics through squeezing,” PRX Quantum 5, 020314 (2024).
- J. Abadie (LIGO Scientific Collaboration), “A gravitational wave observatory operating beyond the quantum shot-noise limit,” Nat. Phys. 7, 962 (2011).
- H. Grote et al., “First long-term application of squeezed states of light in a gravitational-wave observatory,” Phys. Rev. Lett. 110, 181101 (2013).
- A. Eddins et al., “Stroboscopic qubit measurement with squeezed illumination,” Phys. Rev. Lett. 120, 040505 (2018).
- W. Qin et al., “Beating the 3 dB limit for intracavity squeezing and its application to nondemolition qubit readout,” Phys. Rev. Lett. 129, 123602 (2022).
- W. Qin et al., “Exponentially improved dispersive qubit readout with squeezed light,” arXiv:2402.12044.
- W. Qin et al., “Quantum amplification and simulation of strong and ultrastrong coupling of light and matter,” arXiv:2401.04949.
- S. C. Burd et al., “Quantum amplification of boson-mediated interactions,” Nat. Phys. 17, 898 (2021).
- M. Villiers et al., “Dynamically enhancing qubit-photon interactions with anti-squeezing,” arXiv:2212.04991.
- C. Arenz et al., “Amplification of quadratic Hamiltonians,” Quantum 4, 271 (2020).