Connecting a Thin-Shell’s Stiffness with Its Geometry
A sheet of material formed into a curved surface, like a dome or an eggshell, can support a surprisingly large load. Researchers have known for decades how to calculate the way spherical shells respond to external forces. Now, two separate teams writing in Physical Review Letters have used experimental and theoretical methods to better understand how the geometry of nonspherical shells relates to their stiffness. In addition to guiding structural designs, the results can help researchers interpret mechanical measurements on objects ranging from viruses to blimps.
Shells appear in natural forms ranging from the protective protein enclosures around viruses to birds’ eggs and in manufactured forms ranging from table tennis balls to architectural domes. Their combination of light weight and high load-bearing capacity results directly from their geometry, but scientists have not found it easy to work out a full mathematical description of this apparently simple connection.
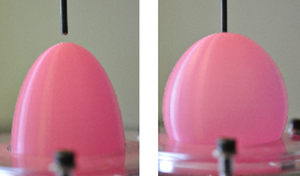
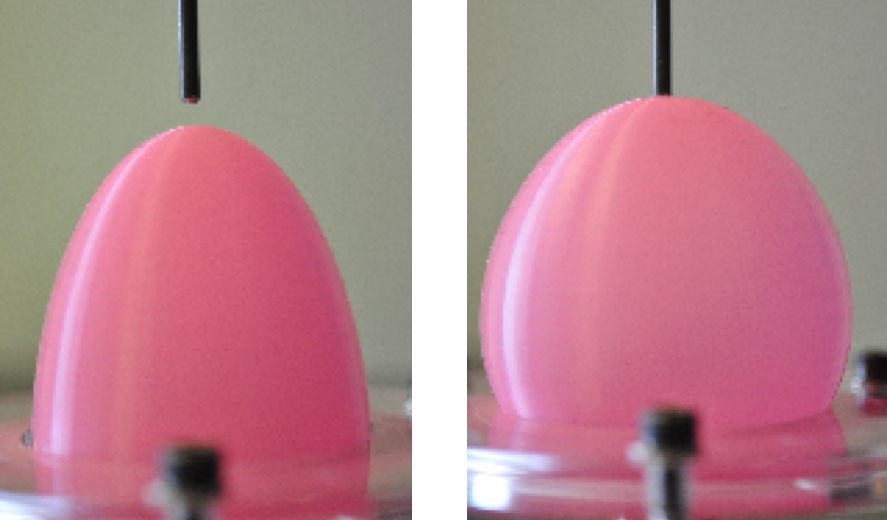
Pushing a pencil point gently against an egg triggers a complex mechanical reaction. The material of the eggshell bends rather easily, so a tiny depression forms at the point of contact. However, flattening out the convex surface pushes the nearby shell outward, squeezing the shell material in directions radiating away from the point of contact. The eggshell resists this lateral compression much more strongly than it resists bending. As a result, it’s harder to deform the more highly curved surface at the tip of an egg than the more gently curved surface of the side of the egg.
For strictly spherical shells, the relationship between bending, compression, and geometry was described mathematically more than sixty years ago. But for more complicated shapes, engineers have come to rely on computer simulations.
Pedro Reis and his team at the Massachusetts Institute of Technology (MIT) in Cambridge set out instead to find basic rules for these deformations experimentally, using new three-dimensional printing techniques to create molds for making precisely shaped “eggshells.” “Being able to 3D-print molds and using fast-curing elastomers allowed us to move much faster in the experiment,” says Reis. The researchers made a series of ellipsoidal shells from different materials and with different shapes, injected them with pressurized fluid, and measured the deformation as they poked them with a narrow tip. Initially, the team intended to explore large deformations and breakage, expecting that for small deformations, “everything must be known,” Reis says. “Little by little, we realized that was not quite the case.”
The simplest case, beyond the sphere, is the “polar” region of a symmetric ellipsoid of revolution, which, like the tip of an egg, has the same curvature in all directions. In this case, the measurements confirmed that the indentation of an ellipsoid is the same as for a sphere with matching curvature. Pressing on the side of the egg is subtly different, however, because the curvature is no longer the same in all directions. The researchers found that the deformation in this case was equivalent to that of a sphere with curvature equal to the average of the curvatures along the “waist” and the long axis of the egg. This way of characterizing the curvature allowed the team to model the stiffness of the ellipsoidal shell by using the known relationship between applied force and depth of indentation for a spherical shell. Moreover, says Reis, their analysis applies to any nonspherical convex shell, since the average curvature at any location can be defined in a similar way.
A second international collaboration has also been studying this problem, supplementing theoretical analysis with numerical simulations. Their original motivation, says Arezki Boudaoud of the University of Lyon in France, was to see if they could determine the internal pressure of plant cells by observing how they indented when prodded. They had previously calculated how internal pressure modifies the indentation response of a spherical shell. “I thought that this would have been worked out somewhere in the literature,” says Boudaoud. “It wasn’t the case.”
Learning of the work by the MIT team, Boudaoud and his colleagues developed a more sophisticated mathematical analysis that applies to nonspherical, pressurized shells. Overall, says Boudaoud, their conclusions agree well with the simpler arguments of the MIT team. But he says “it’s still an open issue” how best to quantify the curvature when it is not the same in all directions. In some cases, instead of the simple average used by the MIT team, the simulations and theoretical analysis by Boudaoud and his colleagues favor using an average based on the product, not the sum, of the curvatures in the principal directions. Experiments of the type conducted by the MIT team cannot easily distinguish between the two possibilities because it’s hard to make long, thin ellipsoids where the two averages would differ significantly.
“This is an exciting time for the mechanics community, because there’s a bunch of new experimental tools … to make structures in all shapes and sizes and materials,” says Douglas Holmes of Virginia Tech in Blacksburg. The new work suggests ways in which it may be possible to exploit geometry to modify structural properties. “Instead of trying to come up with better, stronger, and tougher materials to use, maybe if we redesigned the shape of the material, that would have the same effect.”
–Don Monroe
Don Monroe is a freelance science writer in Murray Hill, New Jersey.