Piecing a Virus Together
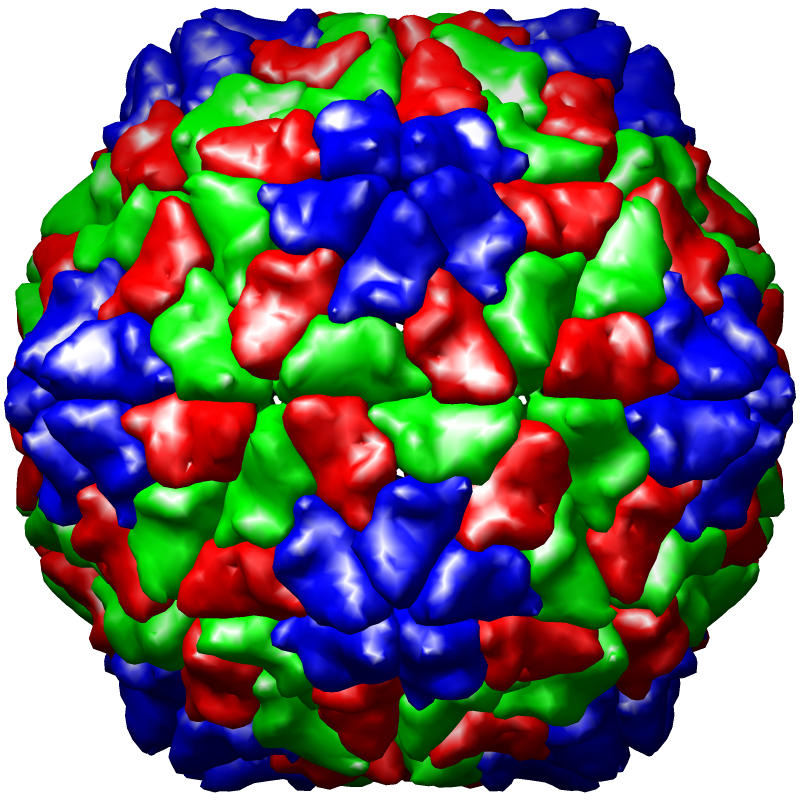
A virus’s genome is protected in a protein shell called a capsid. Capsids have a wide variety of shapes and sizes, but in the May Physical Review E researchers report that capsid structures from many virus families can be constructed using just a single shape, a trapezoid. Their analysis provides an explanation for this common structure based purely on geometry and topology, suggesting that mathematics could be a guiding principle behind capsid design and evolution.
Most capsids have the symmetry of an icosahedron, a 20-sided shape made of triangles. But in capsids, more complex shapes and patterns replace the triangular faces, often with many protruding pieces, so they may not resemble simple icosahedra. The entire structure is made from a small number of different repeating proteins, or “subunits,” consisting of perhaps thousands of atoms each. In 1962, Donald Caspar of Harvard University and Aaron Klug of Cambridge University in England came up with a set of rules for generating geometrical shapes with the same symmetries as capsids [1]. Based on the limited data of the time, this theory generates polyhedra made of hexagons and pentagons, with the soccer ball shape as one example. In the capsid, each hexagonal and pentagonal face represents several protein subunits.
In 2004 Reidun Twarock of the University of York in England extended the Casper-Klug work by representing a capsid as a mosaic of tiles that fit together on a spherical surface, with each tile representing one or more subunits [2]. She found in 2006 that capsids from one particular family could be assembled with just two tile shapes [3].
To better understand capsid geometry, Charles L. Brooks III of the University of Michigan in Ann Arbor and Ranjan Mannige of the Scripps Research Institute in La Jolla, California, wondered if any capsid structures could be represented as mosaics containing only a single tile shape–“monohedral” tilings. Even when a capsid is composed of only one repeated protein, the molecule can be twisted and bent in a variety of ways within the capsid, so monohedral tiling isn’t automatic. The team tapped a database of atomic-scale structures and found 12 virus families (65 viruses) whose capsids were composed of a single protein subunit and were not so simple as to be obviously monohedral. To meet their definition of monohedral tiling, the researchers looked for structures with few gaps or overlaps between neighboring subunits and with subunits whose atomic structure was relatively fixed throughout the capsid. They found that 8 of the 12 families they analyzed had monohedral tilings, regardless of the capsids’ complexity or size.
Then, by applying the Caspar-Klug rules and other geometric principles–such as standard relationships between edges, faces, and vertices of a polyhedron–they discovered that a monohedral tiling can only involve a specific tile shape. It must have five sides, including two equal-length pairs. But the “bisected trapezoid” common in capsids counts as an example, because its long edge is double the length of its short edge and touches two adjacent trapezoids. “Nature is somehow being forced by geometry and topology to give these proteins this very special shape,” Brooks says.
Scientists generally believe that viruses evolved from a common ancestor, the researchers say. But many capsids feature this trapezoidal subunit shape despite having disparate characteristics such as host type and size. This suggests that pure geometry might have triggered certain characteristics of viruses to evolve toward a common design. According to the researchers, these results also lay the groundwork for further analysis of the dynamics of capsids, which change shape and size while infecting a host.
“It’s surprising that you can make a theory to explain that [trapezoidal] shape,” says Michael Widom of Carnegie Mellon University. He adds that this work represents “very nice progress along the lines of fundamental construction principles of capsids.”
–Marcus Woo
Marcus Woo is a freelance science writer in the San Francisco Bay Area.
References
- D. L. D. Caspar and A. Klug, “Physical Principles in the Construction of Regular Viruses,” Cold Spring Harbor Symp. Quant. Biol. 27, 1 (1962)
- R. Twarock, “A Tiling Approach to Virus Capsid Assembly Explaining a Structural Puzzle in Virology,” J. Theor. Biol. 226, 477 (2004)
- R. Twarock, “Mathematical Virology: A Novel Approach to The Structure, Assembly of Viruses, and ” Philos. Trans. R. Soc. London, Ser. A 364, 3357 (2006)