Soap Film Has Memory
A soap bubble is spherical, but a wire frame dipped in soapy water can make other shapes. A research group has now tracked in detail how a film switches from one shape to another when the wire frame is slowly modified. As predicted, the transition point depends on the starting shape, as if the film “remembered” its past. The simple experiment, detailed in the February Physical Review E, is a clear, visual demonstration of a delicately balanced type of equilibrium known as metastability, and it may provide some insights into the way bubbles evolve in solid foams.
A soap film contorts itself in order to minimize its potential energy, which usually means minimizing its surface area. So researchers can determine the minimum surface inside a 3-dimensional framework by building a wire model and dipping it into a soap solution. The resulting film clinging to the wire edges should be the unique solution.
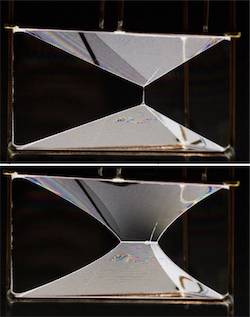
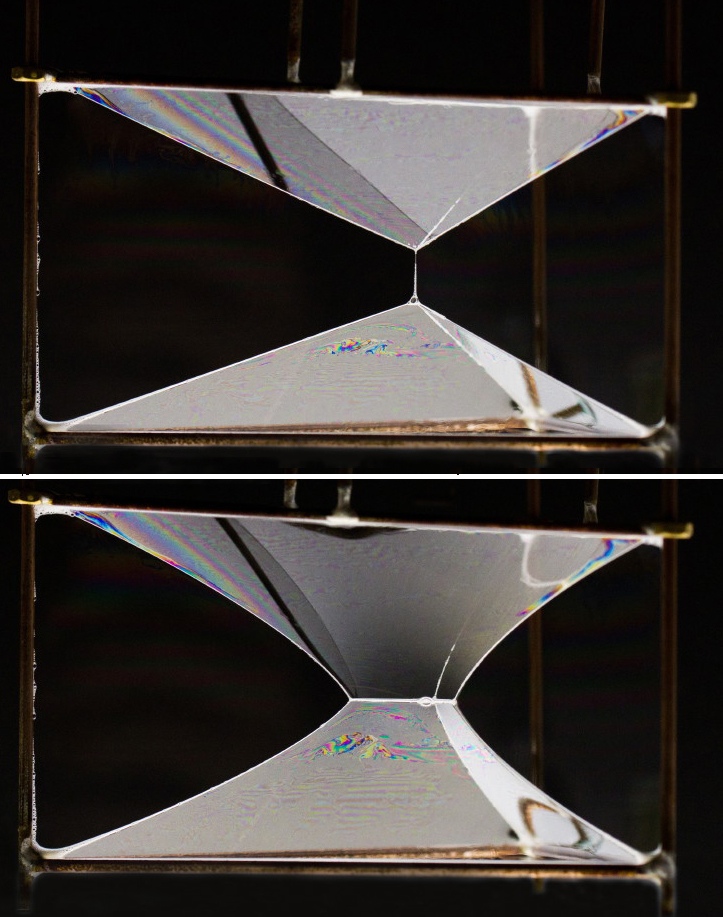
However, 30 years ago researchers found that some frameworks had more than one solution. The simplest example is the triangular prism, which has equilateral triangles for the top and bottom and rectangles for the three vertical sides. When dunked in a soap solution, a film extends toward the center from each of the nine edges, forming three vertical planes and a pair of triangular pyramids–one extending upward from the bottom triangle and a second extending downward from the top triangle. How these pyramids meet in the center depends on the prism’s dimensions.
Numerical calculations show that if the height of the prism is greater than about half of the base length of the triangles, a vertical line where the vertical planes meet connects the tips of the pyramids. But if the height-to-base ratio is less than about 0.4, the pyramid sides take on a slight curve, and their tips merge into a horizontal triangle in the center. In between these two limits, the soap film can be in either configuration. Only one state has the smallest surface area, but the other state can be metastable–stable against small disturbances–because it has less energy than any “nearby” configuration. “You meet metastability in every branch of physics,” says Nicolas Vandewalle of the University of Liege in Belgium. “But if you play with soap films, you can demonstrate it very easily.”
Previous experimentalists have studied this soap film metastability by blowing on a fixed prism to induce transitions from one configuration to the other [1]. To explore the behavior without adding outside energy, Vandewalle and his colleagues constructed a prism whose height could be changed continuously. They started with a soap film in the vertical line configuration and shrunk the prism height until the vertical line length decreased to zero, at a height-to-base ratio of 0.44. At this point, the film switched to the horizontal triangle configuration. When the researchers reversed direction towards a taller prism, the triangle area began to shrink. But the transition back to the vertical line configuration did not occur until a height-to-base ratio of 0.52, showing that the system had some memory of its previous state, an effect known as hysteresis. Hysteresis has been observed in soap films before (e.g., [2]), but this is the first time that a 3D system has been fully characterized.
With some geometric approximations, the researchers calculated the potential energy and showed how it depends on the height-to-base ratio and on the dimensions of the triangle or line in the center of the structure. Plotting this energy as a surface, or “landscape,” this map showed two valleys, or potential wells, corresponding to the two different configurations. If the system started out in one well, it would be trapped there until the height-to-base ratio reached some critical value that pushed it to the other well.
“This is a nice and elegant piece of work,” says Arnaud Saint-Jalmes of the University of Rennes in France. He sees a connection with foams, where metastability causes the foam’s small internal bubbles to rearrange and merge over time, even in solid materials, such as those used for packaging and insulation. Saint-Jalmes says the work could help lead to a better understanding of the relationship between the macroscopic properties of foams and the microscopic evolution of the bubbles or pores.
–Michael Schirber
Michael Schirber is a Corresponding Editor for Physics Magazine based in Lyon, France.
References
- S. Hutzler, M. Saadatfar, A. van der Net, D. Weaire, and S. J. Cox, “The Dynamics of a Topological Change in a System of Soap Films,” Colloids Surf. A 323, 123 (2008)
- D. R. Lovett and J. Tilley, “Illustrating Phase Transitions with Soap Films,” Am. J. Phys. 59, 415 (1991)
More Information
Soapbubble.dk–a “site about soap bubbles, tricks, and their physical attributes”
Hysteresis–a short explanation of the effect in magnetic materials