Tinkering with Superconductivity in a Quasicrystal
A quasicrystal isn’t an obvious home for superconductivity. Conventional theory says that pairs of electrons with opposite momenta are needed for superconductivity, and electron momentum is hard to define in a quasicrystal—a material without long-range periodicity. But in 2018, experimentalists saw the resistance of a quasicrystalline alloy drop to zero—a signature of superconductivity that invited models to describe the unexpected behavior. Using a toy model, researchers now describe how a quasicrystal superconductor responds to a magnetic field and find that the field induces an exotic type of superconductivity that is spatially nonuniform.
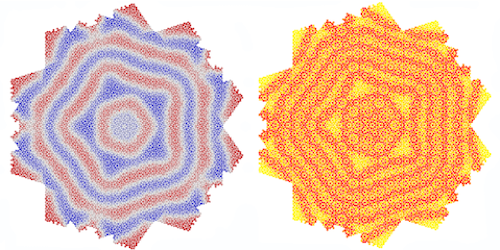
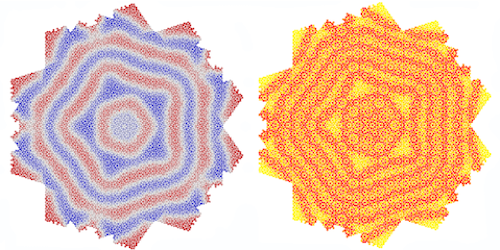
Shiro Sakai and Ryotaro Arita of RIKEN in Japan consider a plane of about 11,000 atoms arranged in a Penrose tiling—one of the simplest quasicrystalline lattices. The team previously predicted superconductivity for this lattice by adapting a simple “Hubbard” model for conventional superconductors. In their new work, they extend the model to include a magnetic field that interacts with the electrons. For a range of field-strength values, they calculate a fundamental parameter of superconductivity—the energy gap—at each point in the lattice.
Sakai and Arita find that when the field strength exceeds a certain value, superconductivity dies, just as in periodic materials. But they also found a range of fields and temperatures where the gap varies in value and sign from point to point in the lattice. This variation is reminiscent of the Fulde-Ferrell-Larkin-Ovchinnikov (FFLO) phase—a spatially inhomogeneous superconductor with a mixture of paired and unpaired electrons. The researchers say quasicrystals may be a new platform for studying the FFLO phase, which has proven tough to see in experiments.
This research is published in Physical Review Research.
–Jessica Thomas
Jessica Thomas is the Editor of Physics.