Quantum trajectories face a transition
When we think about a phase transition, we typically have in mind those that are driven by temperature—say spins locking collectively into the same direction at the Curie temperature of a ferromagnet or molecules forming a rarefied, as opposed to dense, fluid at the boiling point. These phase transitions are associated with critical phenomena that occur in configuration space, but physical systems can also develop critical behaviors in time, that is, in their trajectories through phase space, that closely resemble their thermodynamic analog.
This analogy can reveal important properties of classical nonequilibrium, such as the appearance of a “spacetime” phase transition in glassy systems [1]. Writing in Physical Review Letters [2], Juan P. Garrahan and Igor Lesanovsky at the University of Nottingham in the UK extend this analogy from classical systems to report new dynamical behaviors in quantum systems. They analyze the statistics of events such as photon emissions and uncover critical phenomena, namely, a scale invariance of trajectories as well as a “dynamical phase transition.” These developments provide new insights into the dynamical properties of quantum systems, paving the way for further progress in scenarios ranging from single-electron transistors to superfluid solid states.
Thermodynamic phases are characterized by their free energies, which are governed by extensive observables (energy or magnetization) and their conjugate fields (temperature or magnetic field). A phase transition occurs when the free energy accommodates a nonanalytical behavior in some thermodynamic variable. In the vicinity of a phase transition, other critical behaviors also emerge, such as diverging correlation lengths. Equilibrium statistical mechanics provides an interpretation of macroscopic phases in terms of ensembles of microscopic configurations: the partition function, which measures the number of states accessible to the system under given conditions, characterizes the configuration space. In turn, knowledge of the partition function gives access to the statistical properties (averages and fluctuations) of the thermodynamic variables.
Now, if we are interested in the dynamical aspects of the system, how can we describe ensembles of trajectories? It turns out the statistical mechanics approach can be naturally extended to the time domain by applying a technique called the large deviation (LD) method, developed to describe rare fluctuations or extreme events in random systems. Analogous to the partition function, the large deviation function is a measure of the number of trajectories accessible to the system. Trajectories are categorized by dynamical order parameters (such as the number of configuration changes) or their conjugate fields. Trajectories are given exponential weights similar to Boltzmann factors, with the dynamical order parameters playing the role of energy or magnetization and the conjugate fields the role of temperature or magnetic field.
The LD approach has been very successful in a multitude of problems. For example, Ruelle and Bowen considered large deviations in their analysis of the dynamical properties of chaotic systems [3]. This formalism was further developed to relate the dynamical properties of these systems to their transport properties [4]. More recently, nonequilibrium fluctuations of thermodynamic quantities have been shown to obey universal relationships known as fluctuation theorems (see Ref. [5] for a review). Large deviation relations thus play a fundamental and unifying role in characterizing the dynamical and statistical properties of equilibrium and nonequilibrium systems [6,7].
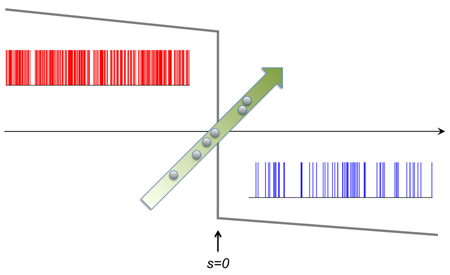
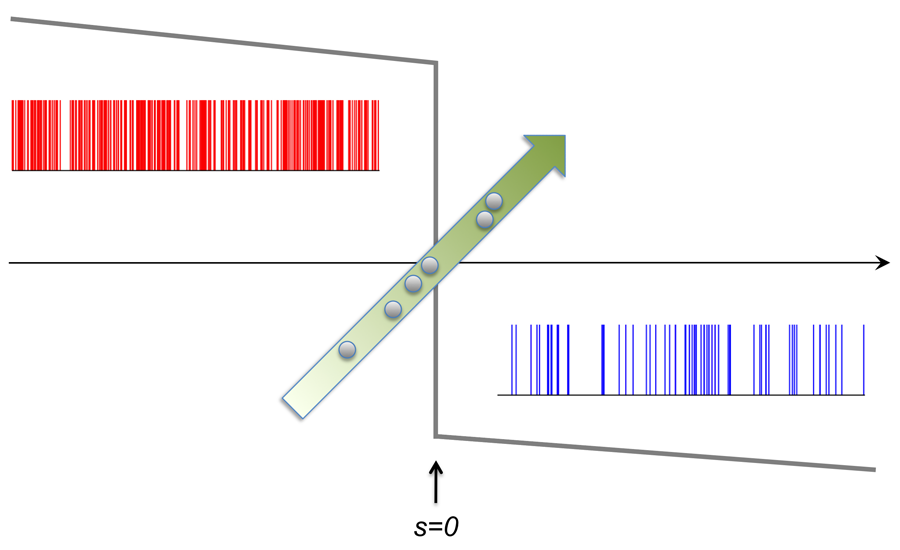
All these results belong to the domain of classical physics. In their paper, Garrahan and Lesanovsky extend these ideas to the quantum realm. As a warm-up problem, they first consider a two-level system driven by an external laser. The random timing of when this two-level system emits photons defines a particular quantum trajectory. Here they find their first surprising result: at some specific driving frequency, trajectories display a scale invariance property. That is, all trajectories would look statistically the same if rescaled by their average emission rate. A spin system analogy would be the following: all spin configurations, scaled by the average magnetization, would look identical for all values of the magnetic field. This strong scale invariance is unanticipated and occurs while all correlation times remain finite.
The authors next consider a three-level system, now driven by two coherent lasers. Remarkably, this seemingly simple generalization completely reshapes the dynamical landscape. Under certain conditions, typical photon emission was observed to be “blinking,” that is, displaying alternating bright and dark periods [8]. Viewed through the lens of the LD formalism, the Nottingham group provides a new explanation of this phenomenon. The trajectories exist in two “flavors”: highly active and irregular on the one hand, or relatively inactive and regular on the other. The system lives precisely at the intersection between these two sets of trajectories (or phases) that are related by a rapid crossover as the conjugate field is varied. The intermittency in the photon emission can thus be understood as reflecting a smoothed phase transition between these two distinct dynamical phases, providing a new context in which to interpret the experimental observations.
Can we actually realize a genuine dynamical phase transition in a quantum setting? Garrahan and Lesanovsky answer this question in the affirmative by considering a microscopic maser, or micromaser. A micromaser consists of excited two-level atoms that pump photons into an optical resonator at a constant rate [9]. Here the statistics of atoms having emitted a photon in the resonant cavity also alternates between an active and an inactive set of trajectories. The transition between the two phases is, however, nonanalytic, with a discontinuity in the first-derivative of the LD function (see Fig. 1). Recalling the parallel with the partition function, this discontinuity is the analog of a first-order thermodynamic phase transition.
For most of the parameters this transition is only visible for nonzero values of the conjugate field (or, equivalently, for rare trajectories), but when the pump parameter (in this case, the rate at which atoms are sent into the resonator) reaches the onset of the micromaser bistability, the phase transition occurs in the physical space and the system lives at the phase coexistence between these two dynamical phases.
The question remains, why did they find these new properties, especially in systems that have already been extensively studied? This fact highlights the power of the LD approach. Looking at the physical space only reveals one facet of the underlying dynamics, whereas LD theory describes the trajectory space from multiple angles through the effect of the conjugate fields. For this reason, and as demonstrated in the present work, the LD approach is likely to be instrumental in studying the statistics of quantum systems.
Garrahan and Lesanovsky’s findings can, in principle, be directly tested experimentally. In two- and three-level systems, photons can be detected with near perfect efficiency, while in the micromaser, the state of emerging atoms is determined by selective ionization in inhomogeneous electric fields. Garrahan and Lesanovsky’s analysis is, however, limited to quantum systems weakly interacting with their environment and it is unclear how these results translate for more entangled systems. Further work is thus necessary to investigate the generality of such critical behaviors.
Perhaps the most surprising finding in their work is the relative simplicity of systems that exhibit rich dynamics. This suggests that similar effects could potentially occur in various scenarios, from Josephson junctions to femtochemistry. Also, the large deviation approach would certainly provide valuable insights into the transport properties of nanoscopic devices. In particular, bidirectional single-electron counting statistics have recently been measured experimentally in structures such as quantum dots [10]. Another important direction of research pertains to the study of many-body quantum systems, which display intricate spacetime dynamics. Dynamical properties of exotic quantum phases could indeed be understood as displaying a critical behavior in the dynamical sense. In any case, the present findings remind us that many more surprising and complex dynamical regimes are waiting to be discovered, even in seemingly simple systems.
References
- L. O. Hedges, R. L. Jack, J. P. Garrahan, and D. Chandler, Science 323, 1309 (2009)
- J. P. Garrahan and I. Lesanovsky, Phys. Rev. Lett. 104, 160601 (2010)
- D. Ruelle, Thermodynamic Formalism: The Mathematical Structures of Classical Equilibrium Statistical Mechanics, (Addison-Wesley Publishing Co., Reading, MA, 1978)[Amazon][WorldCat]
- P. Gaspard, Chaos, Scattering and Statistical Mechanics, (Cambridge University Press, Cambridge, 1998)[Amazon][WorldCat]
- E. M. Sevick, R. Prabhakar, S. R. Williams, and D. J. Searles, Annu. Rev. Phys. Chem. 59, 603 (2008)
- Y. Oono, Prog. Theor. Phys. Suppl. 99, 165 (1989)
- H. Touchette, Phys. Rep. 478, 1 (2009)
- E. Barkai, Y. Jung, and R. Silbey, Annu. Rev. Phys. Chem. 55, 457 (2004)
- G. Raithel, C. Wagner, H. Walther, L. M. Narducci, and M. O. Scully, in Cavity Quantum Electrodynamics, edited by P. R. Berman, Advances in Atomic, Molecular and Optical Physics, Suppl. 2 (Academic, New York, 1994), p. 57[Amazon][WorldCat]
- T. Fujisawa, T. Hayashi, R. Tomita, and Y. Hirayama, Science 312, 1634 (2006)