Turning Down the Volume on Granular Materials
When confronted with a system of many discrete, interacting objects, physicists instinctively reach for ideas from statistical mechanics. Their response to granular materials, such as sand, sugar, or flour, has not been an exception. But these materials possess certain characteristics, such as being inherently dissipative, that render key concepts of statistical mechanics inapplicable, such as thermal equilibrium. To use statistical mechanics, therefore, certain fundamental concepts need to be modified or redefined. But no one knows how to do this in a way that yields a theory that is clear, general, and powerful. There are, however, many proposals. One of the most influential was first presented in 1989 by Sam Edwards and co-workers [1, 2] at Cambridge University, who proposed a statistical mechanics of powders where the volume takes the role of the energy. Now Raphael Blumenfeld—a colleague of Sam Edwards and also at Cambridge (and other institutions)—and collaborators have suggested reformulating Edwards’ proposal by replacing the volume with another function related to the structure of the powder [3]. It is too early to tell where this new proposal will lead, but it does have promising features.
Edwards began by noting that there are many ways of assembling a given set of sand grains into a sand pile. Some of these ways yield a dense, compact pile with a relatively small volume, while others lead to a larger volume. There are also multiple ways of obtaining the same total volume. Furthermore, the total volume can be thought of as the sum of the volumes occupied by each grain. We can therefore think of building a sand pile as dividing the total volume into many pieces and then distributing these pieces among the grains.
Statistical mechanics adopts the same perspective with respect to the energy: a statistical mechanical system has a total energy U that is distributed among the many microscopic degrees of freedom that compose the system. This parallel encouraged Edwards to push the analogy further and define an entropy S(V), analogous to the usual S(U), that is the logarithm of the number of ways of constructing a pile (number of microstates) with the same total volume V. He then followed the formalism of standard statistical mechanics and defined a quantity, the compactivity X=∂S∕∂V, that has the role of the temperature T=∂S∕∂U in the usual formalism. This quantity controls the probability that a given microstate will be realized through a Boltzmann-like factor e−V∕𝜆X, where 𝜆 is a constant that plays the part of Boltzmann’s constant k in the Boltzmann factor e−U∕kT.
It would be quite alarming if this theory were to work in a precise and detailed way because it involves several extraordinary hypotheses. For example, implicit in the role of the compactivity as a control variable is the canonical hypothesis. This says that the probability of a given microstate being realized is proportional to the Boltzmann-like factor. But in the usual statistical mechanics, the Boltzmann factor appears when the canonical ensemble of microstates is constructed. One considers a statistical mechanical system exchanging energy with an enormous “heat bath” that imposes its temperature on the system. Thus the energy of the system fluctuates even though the total energy of the system plus the bath is strictly constant, and the probability of any one system microstate is proportional to the Boltzmann factor even though all allowed microstates of the system plus the bath are equally probable. Although our eyes are fixed on the system, the canonical ensemble is all about the bath. Now, in the case of the proposed volume statistical mechanics of a granular system, interaction with a “volume bath” is replaced by stirring, pouring, or shaking the powder. It would, however, be remarkable if these operations were equivalent to placing the powder in contact with an enormous sand pile with which it exchanges volume. Fortunately, careful numerical simulations [4] have shown that the canonical hypothesis is not verified in one common setup where volume statistical mechanics would seem to apply.
Nevertheless, Edwards’ theory has been successful in that it has raised many questions and suggested new ways of interpreting data. In addition to provoking tests of its assumptions [4], it has prompted experimentalists to develop ways to measure compactivity [5], and the distribution of volume occupied by grains has been scrutinized [6]. The theory also raises the question of the elementary degrees of freedom needed to specify a granular structure and calculate its total volume. These would be the analogies of the velocity components of the molecules in an ideal gas. The usual approach is to use a variant of the Voronoi tessellation that attributes the spaces between the grains to the nearest grain. Then the grain volumes—the grain plus its neighboring empty space—are considered as elementary volumes. The total volume of the packing is thus summed in the same way that it is constructed: grain by grain.
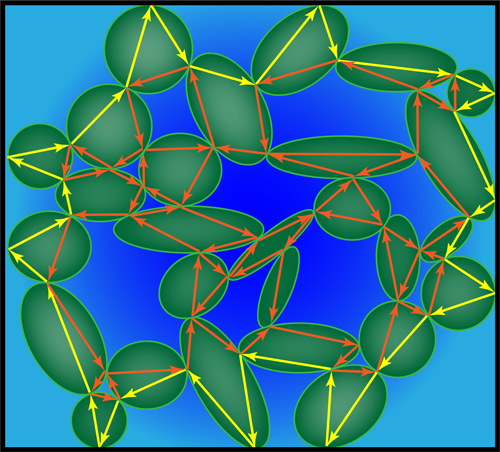
For many years, Blumenfeld and co-workers have been pursuing an alternative approach to calculating the volume based on vectors connecting intragranular contacts [7, 8] (see Fig. 1). These vectors can also be used to partition the volume, and they encode structural information about the packing, such as the average number of contacts. But as the authors point out in their paper [3], considering these vectors as fundamental degrees of freedom for calculating the volume leads to a strange paradox: the volume does not depend on most of these vectors. Indeed, it depends only on those at the surface of the pile, since those in the interior can be modified arbitrarily without affecting the total volume. The researchers conclude that the volume is therefore not a suitable quantity for granular statistical mechanics. Instead, we should consider the sum of the squares of these vectors.
Mathematically, this new quantity, called the connectivity function, resembles many forms of energy, as it is a sum of squares. This might give it many convenient properties, facilitating analogies with other branches of physics. Furthermore, its connection to the contacts means it encodes information about how the packing supports loads, and it could be related to the stored elastic energy or the stress tensor (a quantity that describes the transmission of forces within a material), both of which can be written as sums over contacts. It could thus enlarge the scope of granular statistical mechanics—so far confined to perfectly static piles—to address the important questions of loading and destabilization of granular materials.
That said, the connectivity has no obvious macroscopic meaning, as the volume did. And this highlights a problem with the program of “granular statistical mechanics,” namely that there is no “granular thermodynamics.” Before they were statistical mechanical quantities, the internal energy, entropy, and temperature were macroscopic ones that control the interactions between many very diverse systems (even black holes have a temperature). The analogous quantities in granular statistical mechanics are not so powerful. It is difficult to imagine how two piles of grains would equilibrate by exchanging volume or connectivity, for example. It is clear that a satisfying statistical mechanical description of granular materials has yet to be found, and new ideas are needed. Such a description would indicate which grain-level properties control large-scale behavior of granular flows and packings and would unify many phenomena under a common framework.
This research is published in Physical Review Letters.
References
- S. F. Edwards and R. B. S. Oakeshott, “Theory of Powders,” Physica A 157, 1080 (1989).
- A. Mehta and S. F. Edwards, “Statistical Mechanics of Powder Mixtures,” Physica A 157, 1091 (1989).
- R. Blumenfeld, S. Amitai, J. F. Jordan, and R. Hihinashvili, “Failure of the Volume Function in Granular Statistical Mechanics and an Alternative Formulation,” Phys. Rev. Lett. 116, 148001 (2016).
- F. Paillusson and D. Frenkel, “Probing Ergodicity in Granular Matter,” Phys. Rev. Lett. 109, 208001 (2012).
- E. R. Nowak, J. B. Knight, E. Ben-Naim, H. M. Jaeger, and S. R. Nagel, “Density Fluctuations in Vibrated Granular Materials,” Phys. Rev. E 57, 1971 (1998).
- T. Aste and T. Di Matteo, “Emergence of Gamma Distributions in Granular Materials and Packing Models,” Phys. Rev. E 77, 021309 (2008).
- G. Frenkel, R. Blumenfeld, Z. Grof, and Peter R. King, “Structural Characterization and Statistical Properties of Two-Dimensional Granular Systems,” Phys. Rev. E 77, 041304 (2008).
- R. Hihinashvili and R. Blumenfeld, “Statistical-Mechanical Characteristics of Dense Planar Granular Systems,” Granular Matter 14, 277 (2012).