Type-II Dirac Fermions Spotted
The standard model of particle physics describes all the known elementary particles, like electrons and quarks. Many of these particles have analogs in condensed matter, where they arise as collective states, or quasiparticles. One example is an electronic state in graphene that behaves like a massless Dirac fermion—a spin-1/2 particle that is not its own antiparticle. But condensed-matter physics may offer a longer list of “elementary particles” than found in the standard model. This is due to the fact that—unlike fundamental particles—quasiparticles in solids are not constrained by so-called Lorentz invariance. A Lorentz-violating quasiparticle is one whose momentum-energy relation depends on the direction it travels. Three separate teams [1–3] have collected the first experimental evidence of quasiparticles called type-II Dirac fermions, which break Lorentz invariance. These electronic states, which have no counterpart in the standard model, could be associated with a new type of superconductivity, which has potential applications in thermoelectric devices and quantum computing.
Relativistic quantum field theories provide the framework for our understanding of elementary particles. For many years now, physicists have turned to low-energy condensed-matter systems as a way to investigate quantum field theories without paying the steep price of a high-energy particle collider [4]. These studies have revealed a number of elementary excitations that—like the type-II Dirac fermions—appear in materials but are not present in the standard model. Recent examples include several topological phases [5–9], one of which is the so-called type-II Weyl fermion [9]. A Weyl fermion is a massless spin-1/2 particle whose antiparticle has the opposite chirality, or “handedness.” Weyl fermions have a close relationship to Dirac fermions: a Dirac fermion can be viewed as two superimposed Weyl fermions of opposite chirality that do not mix, because there are crystalline symmetry constraints. Weyl fermions have not been seen in particle physics, but in condensed matter, two different Weyl-like quasiparticles have been observed in the last couple of years. The first quasiparticle is an analog to the typical, or “type-I,” Weyl fermion of quantum field theory [9]. The other quasiparticle, called a type-II Weyl fermion [10], breaks Lorentz invariance in the same way as the type-II Dirac fermion.
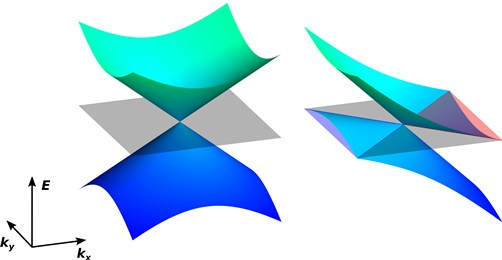
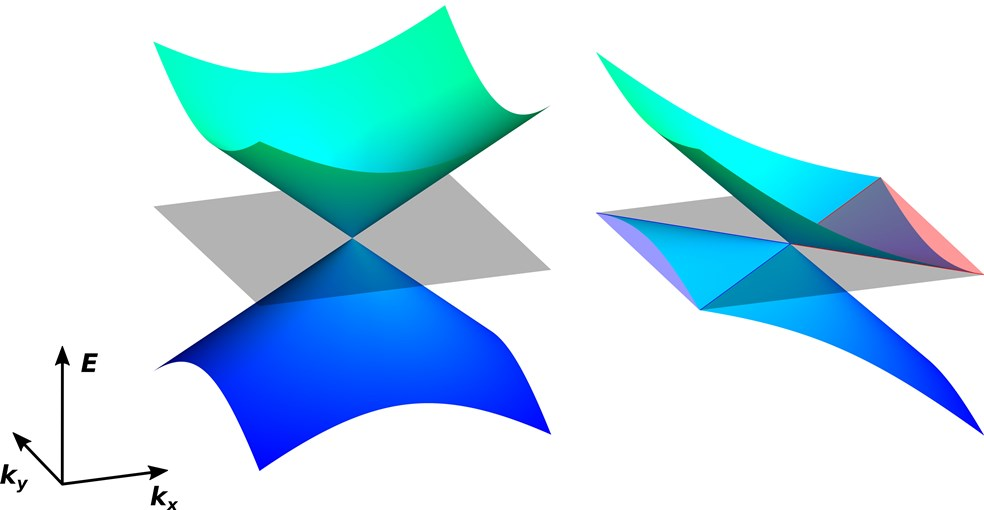
To understand type-II Dirac and Weyl fermions, one can first consider a type-I material, like graphene. The electronic structure of graphene has regions where the valence and conduction bands have cone shapes that meet at a single point, called a Dirac or Weyl point (see Fig. 1). This cone means that the “pocket” for conduction electrons is a small closed region in the energy-momentum diagram just above the crossing point. Similarly, the hole pocket in the valence band is a compact region below the point.
For a type-II material, the energy equation (or Hamiltonian) consists of the type-I linear Hamiltonian and an additional constant (but momentum dependent) term that breaks Lorentz invariance. The Lorentz violation, for both Weyl and Dirac cases, can be represented by a tilted cone in energy-momentum space. As a result of this tilting, the corresponding Dirac points occur at the touching point of the electron and hole pockets. In linear approximation, these open pockets cause significant differences in the behavior of type-II metals compared to the type-I materials with their closed pockets [10]. For example, a type-II material exposed to a magnetic field will conduct electricity only for a certain alignment of the electric and magnetic fields relative to the crystal lattice.
The experimental discovery of type-II Dirac fermions [1–3] came out of studies on two materials, PtTe2 and PdTe2, which are both transition-metal dichalcogenides. Mingzhe Yan from Tsinghua University, China, and colleagues, who were the first to report type-II evidence, worked with single crystals of PtTe2 and performed angle-resolved photoemission spectroscopy (ARPES) measurements [1]. ARPES observations provide an energy-momentum map of the electrons in a solid. When the data were compared to first-principle calculations of the electronic band structure, Yan et al. saw clear signs of the tilted Dirac cones. Similarly, Han-Jin Noh from Chonnam National University, Korea, and colleagues took ARPES measurements of PdTe2 crystals and uncovered type-II structures that matched their calculations [2]. Fucong Fei at Nanjing University, China, and colleagues also studied PdTe2, but they measured the material’s magnetization as a function of an applied magnetic field [3]. They found evidence of type-II Dirac points in a nontrivial Berry phase associated with so-called De Haas-van Alphen oscillations in the magnetization data.
Interestingly, PdTe2 is superconducting at temperatures below 1.7 K. Superconductivity is another condensed-matter phenomenon that has a direct analog with high-energy physics. Inside a conventional superconductor, the electrons pair up through a phonon-mediated coupling, forming bosonic Cooper pairs (quasiparticles) that can be seen as an analog of bosons, like the W and Z particles, in the standard model. A long-standing interest in the field of condensed matter is the interplay between superconductivity and the band topology of electrons. The band structure of Cooper pairs is gapped, but the materials that host superconductivity are gapless metals in the band theory of solids.
How does PdTe2 fit in this picture? It is a metallic material, hosting both superconductivity and topological states in the form of type-II Dirac fermions. This could mean that PdTe2 is a “topological superconductor.” Researchers around the world are trying to create topological superconductors because they are expected to have unique properties not found in normal superconductors. For one, they could conduct heat along their surface, which might be useful in thermoelectric devices. They also could host quasiparticles called anyons. Anyons— of which condensed-matter Majorana fermions are one example—obey special statistical rules that would allow them to perform computations as part of a topological quantum computer. A topological quantum computer would be less prone to environmental decoherence than other types of quantum computers.
Further work is needed to see if PdTe2 is indeed a topological superconductor. Evidence could come from measurements of anomalous behavior in the surface-state thermal conductivity and spin currents, as well as a detection of a conductance peak at zero bias voltage. If PdTe2 does qualify, does it host exotic anyonic excitations? And can it be used for implementing different quantum devices, like the long-sought topological quantum computer? No matter the answers to these questions, this research will stimulate materials scientists to search for more compounds in which band topology intersects with superconductivity—a topic with great technological and scientific promise.
This research is published in Physical Review Letters and Physical Review B.
References
- M. Yan et al., “Lorentz-Violating Type-II Dirac Fermions in Transition Metal Dichalcogenide PtTe2,” arXiv:1607.03643.
- H. J. Noh, J. Jeong, and E.-J. Cho, “Experimental Realization of Type-II Dirac Fermions in a PdTe2 Superconductor,” Phys. Rev. Lett. 119, 016401 (2017).
- F. Fei et al., “Nontrivial Berry Phase and Type-II Dirac Transport in Layered Material PdTe2,” Phys. Rev. B 96, 041201 (2017).
- G. E. Volovik, “The Universe in a Helium Droplet,” (Oxford University Press, Oxford, 2003)[Amazon][WorldCat].
- J. Nissinen and G. E. Volovik, “Type-III and IV Interacting Weyl Points,” arXiv1702.04624.
- Z. Wang, A. Alexandradinata, R. J. Cava, and B. A. Bernevig, “Hourglass Fermions,” Nature 532, 189 (2016).
- B. Bradlyn, J. Cano, Z. Wang, M. G. Vergniory, C. Felser, R. J. Cava, and B. A. Bernevig, “Beyond Dirac and Weyl Fermions: Unconventional Quasiparticles in Conventional Crystals,” Science 353, 6299 (2016).
- T. Bzdušek, Q. Wu, A. Rüegg, M. Sigrist, and A. A. Soluyanov, “Nodal-Chain Metals,” Nature 538, 75 (2016).
- A. A. Soluyanov, D. Gresch, Z. Wang, Q. Wu, M. Troyer, X. Dai, and B. A. Bernevig, “Type-II Weyl Semimetals,” Nature 527, 495 (2015).
- H. Weng, C. Fang, Z. Fang, B. A. Bernevig, and X. Dai, “Weyl Semimetal Phase in Noncentrosymmetric Transition-Metal Monophosphides,” Phys. Rev. X 5, 011029 (2015); S.-Y. Xu et al., “Discovery of a Weyl Fermion Semimetal and Topological Fermi Arcs,” Science 349, 6248 (2015).