Error-Correcting Surface Codes Get Experimental Vetting

Luigi Frunzio; Shraddha Singh

Viewpoint

Error-Correcting Surface Codes Get Experimental Vetting

Department of Applied Physics, Yale University, New Haven, CT, USA

July 11, 2022• Physics 15, 103

Two independent groups have experimentally demonstrated surface-code quantum error correction—an approach for remedying errors in quantum computations.

Figure caption — **Figure 1:** Artistic rendition of the 17-qubit surface code used by Jian-Wei Pan and colleagues to demonstrate surface error correction. The gray disks represent the qubits used to store logical information, while the green and red disks are two different types of “ancilla” qubits used to perform operations that allowed the researchers to detect and correct errors.

For quantum computers to reach their full potential, the need for quantum error correction (QEC) is inevitable. QEC provides a way to fight noise by detecting and correcting the errors in calculations that the noise causes. A promising approach to QEC involves surface codes, where the connections between the elements of the quantum computer can be visualized as forming a 2D checkerboard pattern. These codes are promising because they are experimentally straightforward to implement and because, under certain conditions, they can tolerate relatively large error rates. But until now, demonstrations with the surface code have only detected errors, not corrected them. Now, two groups, led by Jian-Wei Pan at the University of Science and Technology of China in Hefei and Andreas Wallraff at the Swiss Federal Institute of Technology (ETH) in Zurich, have achieved the first-ever demonstration of error correction with surface codes [1, 2]. These results bring us a step closer toward realizing a practical quantum computer.

Error correction requires monitoring the quantum bits, or qubits—the basic units of binary data used for quantum computing—which can simultaneously be in any superposition of $| 0 ⟩$ and $| 1 ⟩$ states. The schemes involve redundantly encoding quantum information of a single “logical” qubit in a many-body entangled state of multiple “physical” qubits so that comparisons between these qubits reveal if one or more of them has changed. Importantly, these comparison measurements do not reveal the actual value of any of the qubits—they only reveal which qubit, if any, has suffered an error. For example, in a simple “repetition code,” to correct for noise that erroneously flips a bit value from $| 0 ⟩$ to $| 1 ⟩$ or vice versa, one can take a 1D chain of multiple qubits and label a logical state $| 0 ⟩$ or $| 1 ⟩$ if the value of all the qubits have a bit value equal to $0$ or $1$ simultaneously. Then if fewer than half of the physical qubits suffer one of these bit-flip errors, the code can catch and correct mistakes before they corrupt the logical data. The number of physical qubits used to encode a logical qubit is called the distance of the code, and a code gets exponentially better at suppressing logical errors as the distance increases (at the cost of increased hardware complexity). Quantum states also suffer phase-flip errors, which affect the superposition of states by converting a $| 0 ⟩ + | 1 ⟩$ qubit state to $| 0 ⟩ - | 1 ⟩$ and vice versa. One can correct for these two types of errors simultaneously by combining bit-flip and phase-flip error-correcting codes. A surface code accomplishes this correction by using a 2D array of qubits.

One of the first steps in demonstrating a successful error-correcting code is achieving “break even,” a condition where the lifetime of the logical qubit is at least as long as that of the best uncorrected physical qubit. This condition has already been achieved in quantum error-correction schemes using a class of codes called bosonic codes [3–7] but not yet in schemes involving a surface code. Doing so will require the error rates of the operations to be significantly lower than they currently are, and it may require using a number of qubits of an order of tens of thousands.

Despite the difficulty in achieving break even, a surface code is still an attractive approach because of its long-term possibility of enabling a fully fault-tolerant quantum computer. If the error rate of a quantum computer is below a certain threshold value, called the fault-tolerant threshold, then the code will be able to deal not only with random qubit errors induced by the environment but also with imperfect operations of the computer and of the error-correcting circuits themselves. Below this threshold value, increasing the code size would decrease error rates of the logical qubit to arbitrarily low values. Surface codes are favorable in this respect: they can tolerate the highest error rate of all fault-tolerant error-correcting schemes. So exploring the advantages and limitations of a surface error-correcting code is essential in determining the future direction of QEC.

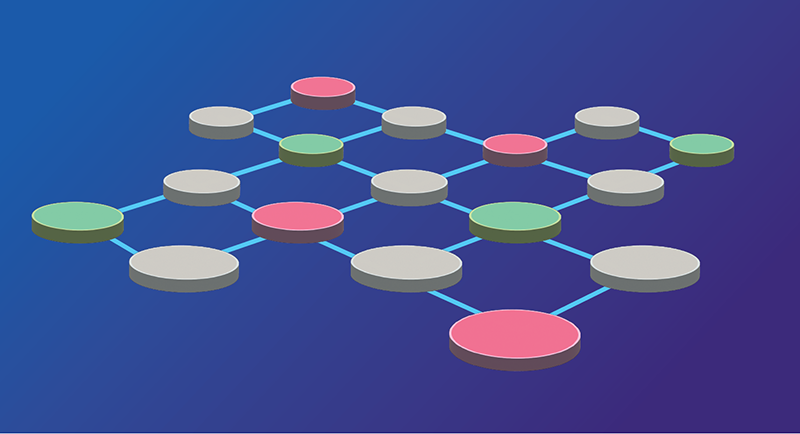
Pan’s and Wallraff’s groups are the first to successfully implement the smallest (with a distance of three) error-correcting surface code. Of the 17 qubits used in their demonstration, nine were used to encode the logical information, while eight were used to read out so-called stabilizer measurements (Fig. 1). These stabilizer measurements revealed which, if any, of the qubits had suffered an error, which Pan and Wallraff could then correct in postprocessing. This result was an incredible feat of engineering that required years of steady reductions of the error rates and of the time required for each cycle of error correction (which together lowered the error-per-cycle rate). The two teams showed that both bit-flip and phase-flip errors could be simultaneously suppressed by multiple rounds of repeated error detection. After applying corrections, Pan’s team reduced the error rate suffered by the logical qubit by 20%.

Wallraff’s team, too, demonstrated an improvement over their uncorrected logical qubit. In addition to the error correction, they employed postselection to remove some instances of leakage errors—those in which the state of a physical qubit “leaks out” of the two-level system composed of the $0$ and $1$ states to some other energy levels of the superconducting circuit. Surface codes cannot correct for leakage errors, and so if these errors are not dealt with, they could significantly limit the ability to scale up the system to large numbers of qubits. Postselection, however, isn’t a long-term strategy for dealing with these errors because by discarding all experimental runs in which a leakage error occurred, it reduces the computational efficiency. How leakage errors will affect the viability of surface codes remains an unresolved question.

These first demonstrations of error correction with a surface code did not achieve break even or fault tolerance, but the results show that surface codes remain promising. The experiments revealed the aspects of current technology that are the biggest limiting factors in achieving a fully fault-tolerant error-correcting code. Some of these factors, such as errors in properly preparing and accurately measuring the qubit states, will surely be improved upon over the next few years. Others, such as leakage errors and unwanted interactions between neighboring qubits, may spell the death of surface error-correcting codes. The jury is still out on whether surface codes will be a feasible approach to fault-tolerant quantum computing but impressive experiments such as those by Pan’s and Wallraff’s teams bring us closer to finding out.

References

Y. Zhao et al., “Realization of an error-correcting surface code with superconducting qubits,” Phys. Rev. Lett. 129, 030501 (2022).
S. Krinner et al., “Realizing repeated quantum error correction in a distance-three surface code,” Nature 605, 669 (2022).
N. Ofek et al., “Extending the lifetime of a quantum bit with error correction in superconducting circuits,” Nature 536, 441 (2016).
L. Hu et al., “Quantum error correction and universal gate set operation on a binomial bosonic logical qubit,” Nat. Phys. 15, 503 (2019).
C. Flühmann et al., “Encoding a qubit in a trapped-ion mechanical oscillator,” Nature 566, 513 (2019).
P. Campagne-Ibarcq et al., “Quantum error correction of a qubit encoded in grid states of an oscillator,” Nature 584, 368 (2020).
B. de Neeve et al., “Error correction of a logical grid state qubit by dissipative pumping,” Nat. Phys. 18, 296 (2022).

About the Authors

Luigi Frunzio is a low-temperature condensed matter experimentalist at Yale University. He specializes in the fabrication and characterization of superconducting quantum circuits for quantum information processing and for fault-tolerant quantum-error-corrected computation. He earned a master’s degree in physics from the University of Naples Federico II, Italy, and worked on superconducting electronics at the Institute of Cybernetics of the National Research Council in Arco Felice, Italy. He holds a Ph.D. in physics from University of Paris-Sud in Orsay, France.

Shraddha Singh is a Ph.D. student at Yale University. She works on the intersection of bosonic error correction and surface codes. During her master’s thesis she was affiliated with Sorbonne University, where she and Elham Kashefi founded the “Quantum Protocol Zoo”—an open repository of protocols for quantum networks. Prior to this, she was part of a combined BS/MS program at UM-DAE Centre for Excellence in Basic Sciences in India, where she worked on methods in statistical physics.