Depletion-Force Measurements Get Active
Depending on the properties of the fluid in which they are suspended, particles called colloids can attract each other. That happens when this so-called colloidal suspension also contains “depletants,” which are typically smaller colloids or polymer molecules. Most of the studied depletants are passive, and the magnitude of the attractive force they induce, known as the depletion force, is well defined [1, 2]. Less studied are “active” depletants, which are present in living systems, for instance in a cell’s cytoplasm, where they move around by some self-propulsion mechanism [3–6]. The depletion interaction of particles within these “active fluids” is much less clear [7, 8]. Now, using experimental data, Clemens Bechinger at the University of Konstanz, Germany, and colleagues establish a simple relationship between induced depletion forces and a colloidal-suspension’s microstructure for a certain class of active depletants [9]. This result marks an important step toward understanding the spatiotemporal organization within active fluids.
In a colloidal suspension, the various constituent particles can self-assemble into suprastructures. This self-assembly is caused by collective interfacial forces among the constituents. The depletion interaction is one example of such a force. The depletion force has a fascinating history and was first described in a 1954 one-page theory paper by physicists Sho Asakura and Fumio Oosawa [1, 10]. In a fluid containing large colloids and small depletants, when two colloids get close enough, the depletants get excluded from the space between the colloids. This exclusion drives a pressure imbalance around the colloids—the depletion force—that pulls the colloids closer.
Asakura and Oosawa derived a constitutive relationship between this depletion force and the density distribution of depletants around the colloids. But it only works for systems where the motion of the depletants is driven purely by thermal buffeting by the fluid, such as in milk [1, 2]. No constitutive relation was previously established for any colloidal solution with active depletants, such as a suspension of motile bacteria.
Active systems remain far from equilibrium because of their constant energy flux. A consequence of this nonequilibrium behavior is that active systems are liberated from the constraints of detailed balance—a fundamental principle related to the reversibility of elementary thermodynamic processes. Hence, the interaction among the depletants and the colloids can lead to generation of steady currents in the system’s phase space. These currents can manifest in the rotation of an asymmetric microgear placed in an active fluid, for example, something that is impossible in a passive solution due to detailed balance [11].
Because of this behavior, there is a nontrivial distribution of the fluid and depletants around a colloid, which has made it difficult to pin down the relation between this distribution and the depletion forces that develop. To date, measurements and theory indicate that this distribution is both different from that seen for passive depletants and—crucially—dependent on the “class” of active system being used, meaning how the propulsion directions of the active depletants affect how they interact with each other [12]. Because of this class dependence, Bechinger and colleagues focused on one class of active depletant—scalar active depletants—in which the interactions between depletant particles does not depend on their respective self-propulsion orientations.
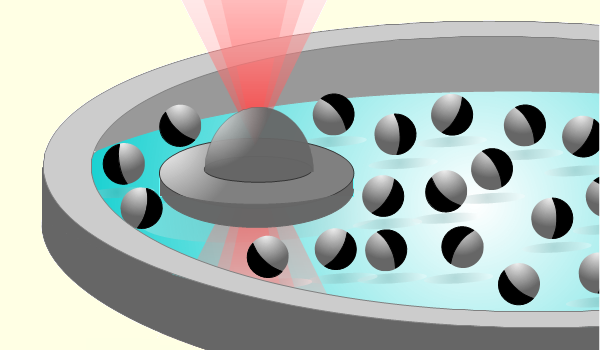
The team performed experiments using a model scalar active depletant, consisting of -diameter silica particles that had one hemisphere coated by a thin layer of carbon. They immersed these so-called Janus particles in a shallow disk of fluid and activated their motion using light. The light triggered an interaction at the carbon caps that propelled the particles at speeds of around [13]. Into this active “bath” the team placed a passive -diameter disk, which they held in place with a laser-based optical trap. The optical trap allowed them to monitor the depletion force experienced by the disk as it was jostled around by the active particles (Fig. 1). They used an optical microscope to measure the distribution of particles as a function of their distance from the center of the disk.
This measurement combination allowed the team to simultaneously capture the microstructure of the depletants and the depletion force experienced by the disk, and thus to understand how the two were related. Using recent theoretical results, they quantified the relationship between the depletion force and both the depletant density around a passive colloid and the depletant polarization [8, 14]. Interestingly, the team recovered a relationship similar to that obtained for passive systems, with activity manifesting as an increased effective temperature of the fluid. The team then used numerical simulations to corroborate these results and to quantify the impact on this relationship of factors such as the surface roughness of the particles and hydrodynamic and phoretic effects.
How active particles generate forces on passive ones is crucial to understanding the spatial organization of objects within active matter systems. This current study provides a significant addition to the growing list of exciting insights into scalar active systems. These measurements also bolster efforts to develop effective coarse-grained descriptions for these systems. Such insight is crucial for the design of active systems that can harness collective forces to perform work or for designing active responsive metamaterials.
This finding, together with other recent developments in understanding the collective behaviors in active systems, raises the tantalizing possibility of a physical description of the spatiotemporal organization within living systems, the archetypal active system. Biological systems are evolved, complex, and inhomogeneous, making them very different from most model physical systems. It would therefore be a significant breakthrough if we could unravel the quantitative relationships underlying their dynamic spatiotemporal organization by studying much simpler physical systems.
References
- S. Asakura and F. Oosawa, “On interaction between two bodies immersed in a solution of macromolecules,” J. Chem. Phys. 22, 1255 (1954).
- H. N. Lekkerkerker and R. Tuinier, Colloids and the Depletion Interaction, Lecture Notes in Physics Vol. 833 (Springer, Dordrecht, 2011)[Amazon][WorldCat].
- W. F. Paxton et al., “Catalytic nanomotors: Autonomous movement of striped nanorods,” J. Am. Chem. Soc. 126, 13424 (2004).
- S. Thutupalli et al., “Swarming behavior of simple model squirmers,” New J. Phys. 13, 073021 (2011).
- J. Deseigne et al., “Collective motion of vibrated polar disks,” Phys. Rev. Lett. 105, 098001 (2010).
- M. Rubenstein et al., “Kilobot: A low cost scalable robot system for collective behaviors,” 2012 IEEE Int. Conf. Robotics and Automation 3293 (2012).
- M. C. Marchetti et al., “Hydrodynamics of soft active matter,” Rev. Mod. Phys. 85, 1143 (2013).
- T. Speck, “Collective forces in scalar active matter,” Soft Matter 16, 2652 (2020).
- S. Paul et al., “Force generation in confined active fluids: The role of microstructure,” Phys. Rev. Lett. 129, 058001 (2022).
- K. Kurihara and B. Vincent, “The discovery of the depletion force,” J. Chem. Phys. 154, 220401 (2021).
- R. Di Leonardo et al., “Bacterial ratchet motors,” Proc. Natl. Acad. Sci. U.S.A. 107, 9541 (2010).
- A. P. Solon et al., “Pressure is not a state function for generic active fluids,” Nat. Phys. 11, 673 (2015).
- J. R. Gomez-Solano et al., “Tuning the motility and directionality of self-propelled colloids,” Sci. Rep. 7, 14891 (2017).
- T. Speck and A. Jayaram, “Vorticity determines the force on bodies immersed in active fluids,” Phys. Rev. Lett. 126, 138002 (2021).