Record Precision for Hydrogen Spectroscopy Measurements
For over a century, hydrogen has been used to test quantum theories, including quantum electrodynamics (QED), the quantum-field theory describing light–matter interactions. An important QED test involves measuring the splitting of an atom’s electron energy levels that is induced by nucleus–electron interactions, a phenomenon known as hyperfine splitting. Now a team led by Dylan Yost of Colorado State University has measured with record-breaking precision one such splitting for hydrogen. The achievement could allow researchers to perform unprecedentedly strict tests of QED.
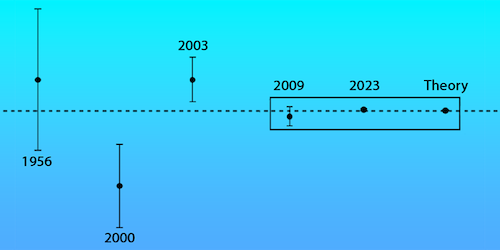
Yost and his colleagues measured the splitting of hydrogen’s first excited electronic states (2S), which has been targeted by experiments since 1956. The previous precision record was achieved in 2009, in an experiment that used an optical “frequency comb” to derive the splitting. This measuring approach uses the ground-to-excited-state transition energies, calculating the small hyperfine splitting by subtracting the energy of the ground state from those of the 2S split states. A better approach is to directly measure the splitting, Yost says. “It’s like measuring a small distance directly rather than obtaining it as the difference of two very large ones.”
To do that, the researchers used an approach called Ramsey spectroscopy, the same one as in the original 1956 experiment. The technique measures the splitting by using resonant radio-frequency fields to directly induce electron transitions between the split states. The value they obtain is 8 times more precise than that measured in 2009 and is consistent with QED predictions. Yost anticipates that they could achieve another tenfold precision improvement by increasing their setup’s size. That would place this kind of measurement among the best precision tests of QED that can be performed with current technology.
–Matteo Rini
Matteo Rini is the Editor of Physics Magazine.
References
- R. G. Bullis et al., “Ramsey spectroscopy of the 2S1/2 hyperfine interval in atomic hydrogen,” Phys. Rev. Lett. 130, 203001 (2023).