Foiling Quantum Hackers
Quantum cryptography holds promise for communication schemes that are, in theory, perfectly secure. But in the last few years, hackers have exploited security loopholes to crack some of the most sophisticated quantum encryption systems. Fortunately, two new papers in Physical Review Letters, one by Allison Rubenok, at the Institute for Quantum Science & Technology in Calgary, Canada, and colleagues [1] and the other by Yang Liu, at the Hefei National Laboratory for Physical Sciences and the University of Science and Technology of China, and colleagues [2] now report a new quantum encryption method that can remove the weakest link of quantum encryption schemes: loopholes associated with defects of the photodetectors used at the receiver end. Code makers have now regained the upper hand against code breakers.
Code makers and code breakers have been fighting for thousands of years. With the rise of the Internet, the importance of communication security is growing. Each time we do online banking or use messaging apps on our smart phones, we should be concerned about communication security. Conventional (classical) cryptographic systems are often based on unproven computational assumptions.
In contrast, quantum encryption methods such as quantum key distribution (QKD)—the use of quantum states to transmit a shared encryption key between communicating parties—offer, in principle, unconditional security based on the laws of physics. This is an ideal solution in the long term, but we are not there yet: while QKD schemes have been demonstrated and have already led to commercially available products, a few research groups, in the last few years, have reported a number of high-profile successful hacks [3] against QKD systems, thus casting doubts on the security of practical QKD. Charles Bennett [4], an IBM Fellow and a co-inventor of quantum cryptography, wrote: “Photon detectors have turned out to be an Achilles’ heel for quantum key distribution (QKD), inadvertently opening the door … to subtle side-channel attacks.”
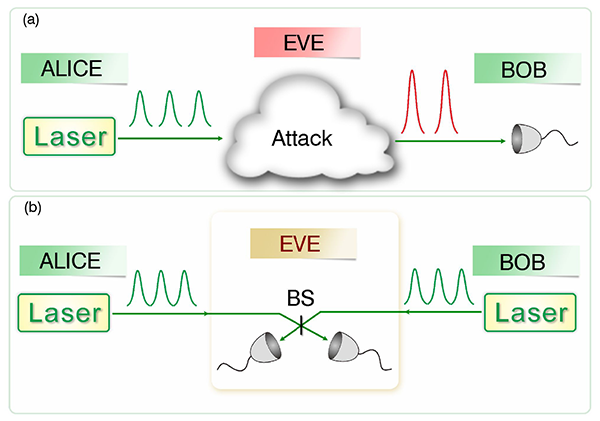
In one hacking example, Eve (the eavesdropper) can blind the detectors of Bob (the receiver) with a low-power, continuous-wave laser. While the detector is blinded, it works as a classical detector and loses the “quantum” protection: Eve can then intercept, unnoticed, Alice’s (the sender’s) signals. Countermeasures for quantum hacking have been proposed—such as security patches [5], teleportation tricks [6], and full device-independent QKD (DI-QKD) [7]—but all have been proven to be either ad hoc or impractical, e.g., not compatible with long-distance communications or with key generation at sufficient speeds.
The two new papers implement a new quantum encryption method called “measurement-device-independent” QKD (MDI-QKD) that was first put forward in 2012 by my research group [8] and appears today to be the only practical solution to quantum hacking at the detector side. A simplified schematic diagram of a possible MDI-QKD setup is shown in Fig. 1. The security of MDI-QKD relies on a time-reversed version [9] of an Einstein-Podolsky-Rosen (EPR) type of QKD protocol. In essence, Bob and Alice generate sequences of randomized pulses and send them to a third party (which may even be an untrusted eavesdropper, Eve) who performs a so-called Bell-state measurement of the two incoming signals: the outcome of the measurement is successful for those combinations of Alice’s and Bob’s photons that are in the same Bell state. These measurements do not need to be secret: once the results are publicly announced, they allow Bob and Alice to pick the sequence of bits associated with successful Bell’s measurements as their private encryption key. What makes MDI-QKD practical is the fact that it can work with attenuated laser pulses (as opposed to ideal single-photon sources) and with so-called decoy state techniques (which use decoy signals to confuse Eve and allow Alice and Bob to obtain additional information to detect Eve’s action) [10].
MDI-QKD has the remarkable advantage of being inherently immune to all attacks against detectors, thus foiling all such existing and potential attacks. In fact, with MDI-QKD, one could even outsource the manufacturing of photon detection systems—the most challenging component of QKD—to an untrusted party without compromising security. This means, for instance, that a top-secret communication between two countries could be securely held even with a completely untrusted network node manufactured by a third, hostile party—a dream scenario in communication security.
The papers by Rubenok et al. and Liu et al. are important because they bring us closer to the practical application of MDI-QKD, showing that it can work on actual fiber links of a certain length, and, unlike the original demonstration paper [8], even if Bob’s and Alice’s signals have different intensities.
Rubenok et al.’s [1] experiment is the first field test of MDI-QKD with installed fibers: the author provided both a lab demonstration of the MDI approach over more than of spooled fiber as well as over of installed fibers connecting different locations within the city of Calgary (Canada). The authors prove that a number of essential elements of MDI-QKD can be made to work. First, they show that the scheme can work with attenuated pulses of varying photon numbers, without relying on single photons. Second, as in Ref. [8] but now over much longer transmission distances, they manage to fulfil an important requirement: Bell-state measurements can work only if Bob and Alice send out photons that are not distinguishable (in terms of spectrum, polarization, and timing). This is achieved through the implementation of active stabilization systems. However, the article falls short of being a full demonstration of QKD: the authors transmit the same key a number of times but do not perform a random generation of key bits and signal intensities.
Liu et al.’s lab experiment [2] goes one step further and performs a real QKD experiment. Similarly to the experiment of Rubenok et al., the authors use attenuated pulses and are able to control the pulses’ spectrum, timing, and polarization to make them undistinguishable. Furthermore, they use trusted sources that generate truly random keys: randomized coherent states with intensity modulation. The authors evaluate the scheme’s performance realistically, taking into account the finite length of the key. However, one should notice that their experiment requires expensive components for both state preparation and photon detection. For instance, expensive and complicated custom-made detectors are used, which may be available to only a few research groups in the world.
What lies in the future? Both experiments used a specific encoding scheme called time-bin encoding (in which information is encoded in photons arriving at different times). One needs to demonstrate the versatility of MDI-QKD with other popular encoding schemes such as polarization encoding [11]. Practical applications also demand higher speed: it is reasonable to think that the -megahertz scheme of Liu et al. may be extended to the delivery of secure keys at gigahertz rates. Finally a more systematic parameter optimization will need to be carried out and an MDI-QKD demonstration with standard commercial off-the-shelf single-photon detectors will be needed to pave the way for widespread deployment. In the longer term, demonstrating the feasibility of MDI-QKD in a network setting (as opposed to a one-to-one conversation) will be an important milestone. Conceptually, MDI-QKD allows many individual users to use, at their end, only simple signal preparation devices and outsource expensive and complex measurement devices to even untrusted network providers, which is very appealing for network security [12].
Should the above-mentioned outstanding issues be resolved, MDI-QKD will change the landscape of security research in QKD by making quantum hacking against detection systems obsolete, thus forcing quantum code breakers to attack QKD at the signal preparation stage in future.
Acknowledgments
H.-K. Lo acknowledges enlightening discussions with colleagues including Bing Qi, Li Qian, and Feihu Xu.
References
- A. Rubenok, J. A. Slater, P. Chan, I. Lucio-Martinez, and W. Tittel, “Real-World Two-Photon Interference and Proof-of-Principle Quantum Key Distribution Immune to Detector Attacks,” Phys. Rev. Lett. 111, 130501 (2013)
- Y. Liu et al., “Experimental Measurement-Device-Independent Quantum Key Distribution,” Phys. Rev. Lett. 111, 130502 (2013)
- Y. Zhao et al., “Quantum Hacking: Experimental Demonstration of Time-Shift Attack Against Practical Quantum Key Distribution Systems,” Phys. Rev. A 78, 042333 (2008); F. Xu, B. Qi, and H.-K. Lo, “Experimental Demonstration of Phase-Remapping Attack in a Practical Quantum Key Distribution System,” New J. Phys. 12, 113026 (2010); L. Lydersen et al., “Hacking Commercial Quantum Cryptography Systems by Tailored Bright Illumination,” Nature Photon. 4, 686 (2010); I. Gerhardt et al., “Full-Field Implementation of a Perfect Eavesdropper on a Quantum Cryptography System,” Nat. Commun. 2, 349 (2011); N. Jain et al., “Device Calibration Impacts Security of Quantum Key Distribution,” Phys. Rev. Lett. 107, 110501 (2011); Q. Liu et al., “A Universal Setup for Active Control of a Single-Photon Detector,”arXiv:1307.5951 (2013)
- C. H. Bennett, “Let Eve do the heavy lifting, while John and Won-Young keep her honest,” http://dabacon.org/pontiff/?p=5340
- Z. L. Yuan, J. F. Dynes, and A. J. Shields, “Reply to “Comment on ‘Resilience of gated avalanche photodiodes against bright illumination attacks in quantum cryptography’”,” Appl. Phys. Lett. 99, 196102 (2011)
- See H.-K. Lo and H. F. Chau, “Unconditional Security of Quantum Key Distribution over Arbitrarily Long Distances,” Science 283, 2050 (1999), footnote 21
- D. Mayers and A. C.-C. Yao, “Quantum Cryptography with Imperfect Apparatus,” in Proceedings of the 39th Annual Symposium on Foundations of Computer Science (FOCS98), (IEEE Computer Society, Washington, DC, 1998)[Amazon][WorldCat]; A. Acin et al., “Device-Independent Security of Quantum Cryptography Against Collective Attacks,” Phys. Rev. Lett. 98, 230501 (2007); N. Gisin, S. Pironio, and N. Sangouard, “Proposal for Implementing Device-Independent Key Distribution Based on a Heralded Qubit Amplifier,” 105, 070501 (2010); M. Curty and T. Moroder, “Heralded Qubit Amplifiers for Practical Device-Independent Quantum Key Distribution,” Phys. Rev. A 84, 010304 (2011)
- H.-K. Lo, M. Curty, and B. Qi, “Measurement-Device-Independent Quantum Key Distribution,” Phys. Rev. Lett. 108, 130503 (2012)
- E. Biham, B. Huttner, and T. Mor, “Quantum Cryptographic Network Based on Quantum Memories,” Phys. Rev. A 54, 2651 (1996); H. Inamori, “Security of Practical Time-Reversed EPR Quantum Key Distribution,” Algorithmica 34, 340 (2002); See also S. L. Braunstein and S. Pirandola, “Side-Channel-Free Quantum Key Distribution,” Phys. Rev. Lett. 108, 130502 (2012)
- W.-Y. Hwang, “Quantum Key Distribution with High Loss: Toward Global Secure Communication,” Phys. Rev. Lett. 91, 057901 (2003); H.-K. Lo, X. Ma, and K. Chen, “Decoy State Quantum Key Distribution,” 94, 230504 (2005); X.-B. Wang, “Beating the Photon-Number-Splitting Attack in Practical Quantum Cryptography,” 94, 230503 (2005)
- T. Ferreira da Silva, D. Vitoreti, G. B. Xavier, G. P. Temporao, and J. P. von der Weid, “Proof-of-Principle Demonstration of Measurement Device Independent QKD Using Polarization Qubits,”arXiv:1207.6345 (2012)
- R. J. Hughes, “Network-Centric Quantum Communications with Application to Critical Infrastructure Protection,”arXiv:1305.0305 (2013); B. Fröhlich et al., “A Quantum Access Network,” Nature 501, 69 (2013)