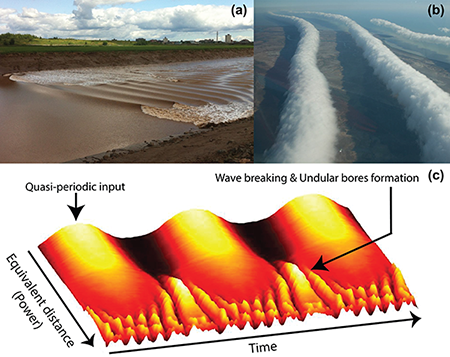
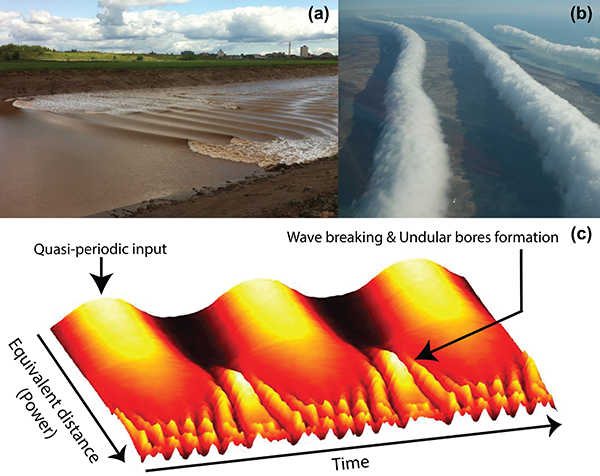
Water Waves in Optical Fibers
Undular bores are among the most interesting phenomena in nature. A familiar case is the tidal bore: A stable, moving water wave front caused by a large rise in sea level into a narrow channel during a flood tide. In essence, the front is a traveling wave that is continuously breaking, like waves hitting a shoreline. Undular bores can occur under specific conditions that cause the moving front to leave behind a nonstationary oscillating wave train [1]. Numerous examples illustrating this phenomenon can be found in nature (Fig. 1), ranging from the formation of large-scale atmospheric gravity waves (seen in the so-called “Morning Glory” phenomenon in Australia, for example) to impressive tidal bores appearing in several river estuaries all over the world (such as the Peticodiac River tidal bore in Canada). Now, reporting in Physical Review X, Julien Fatome at the University of Bourgogne, France, and colleagues demonstrate how faithfully these hydrodynamic phenomena can be recreated and studied in optical analog experiments [2]. In these experiments, they see waves reminiscent of undular bores, generated as light waves propagate in an optical fiber.
Although undular bores have been widely observed in nature, their experimental investigation in the original environment still represents a challenge, mainly due to the difficulty of engineering the complex initial conditions in a well-controlled and repeatable way. As a consequence, and in spite of long-standing research in related areas of physics such as hydrodynamics [3], atmospheric physics, or even plasma physics, new insights have been mainly obtained by either analytical or numerical approaches.
Optical systems, and specifically those featured by pulse propagation in optical fibers, can be excellent models for testing similar nonlinear dynamics. In 1995, Kodama and Wabnitz derived a set of equations for the evolution of pulses in a nonlinear fiber that closely resemble those governing the one-dimensional spatial evolution of shallow water waves [4]. This finding, among others, showed a strong similarity between hydrodynamics and nonlinear fiber optics.
An optical approach can therefore provide a more convenient and controlled environment to experimentally study specific nonlinear hydrodynamic problems. Recent analogies between optical supercontinuum generation (formation of broadband light from quasimonochromatic laser pulses) and the occurrence of oceanic rogue waves [5] have stimulated numerous studies in the photonics community. Among other results, these have led to the first experimental characterizations of some peculiar hydrodynamic solutions analytically predicted decades ago [6], later followed by the experimental observations of their counterparts in deep water hydrodynamics [7].
Within this context, the results obtained by Fatome et al. [2] provide the first experimental characterization of optical undular bores and their subsequent propagation dynamics in optical fibers. Like their water counterparts in river estuaries, optical bores emerge from a shock in the system and propagate like fast oscillating wave trains. In order to mimic this spectacular phenomenon, the authors designed an experimental setup where the spontaneous optical wave breaking is initiated by four-wave mixing (FWM)—a common nonlinear frequency mixing process used in numerous fundamental and applied fields of photonics (see, e.g., Ref. [8] and references within). As a succinct reminder, FWM arises from the interaction of two frequency components ( and ) propagating together in a third-order nonlinear medium and leading to the generation of two new frequency components complying with energy conservation, i.e., .
In their experiment, Fatome et al. designed a fiber configuration in a regime of weak normal dispersion, in which (i) the low-frequency components travel faster than the high-frequency ones, and (ii) the dynamics is mainly dominated by the Kerr nonlinearity (due to this effect, the index of refraction of the medium varies temporally according to the instantaneous intensity of the optical wave). As quasiperiodic signals (that is, bunches of modulated continuous wave signals) were launched to propagate along the fiber, FWM leads to the generation of a frequency cascade corresponding to the higher harmonics of the initial modulation. Taking advantage of these particular initial conditions, Fatome et al. were able to observe the spontaneous breaking of the propagating wave train arising from the interplay of the nonlinearity and the weak normal dispersion associated with the fiber under investigation.
In such a case—when nonlinearity overcomes dispersion—the Kodama-Wabnitz approach remains valid and light propagation follows the wave equation for one-dimensional shallow water waves. The wave-breaking mechanism seen by the authors can thus be understood with the help of classical hydrodynamics. Like a water wave that steepens while approaching the shore (as sections of its envelope with the highest elevation also present the highest velocity), an optical wave propagating in a Kerr medium will undergo a nonlinear phase shift, proportional to its instantaneous power. This results in a “chirp,” i.e., a temporally varying instantaneous frequency along the optical wave envelope. The trailing (leading) edge of the wave, within a given modulation period, therefore has a slightly higher (lower) frequency than the wave central part. In the normal dispersion regime of Ref. [2], the frequency shift induced by the nonlinearity will progressively result in the steepening of the initially smooth wave envelope edges, ultimately leading to wave breaking.
An important point to be underlined is that the propagation equations can, in fact, be integrated until the stage where the wave envelope singularity appears (that is, the formation of vertical edges on the wave envelope leading to its breakup). As reported in Ref. [2], the point at which this singularity appears can be predicted analytically in the case of a modulated carrier, and it is found to depend exclusively on the input signal-to-pump relative intensity and frequency detuning. By properly setting these parameters and using an appropriate optical fiber (for instance, a - -long nonzero-dispersion-shifted fiber), one can essentially replace the variation in propagation distance with adjustments to the input wave power: when nonlinear dynamics dominates, increasing the pulse intensity leads to additional nonlinear effects, which build up the nonlinear phase shift in the same way as further propagation would do. The authors take advantage of this specific property by directly mapping the propagation dynamics at different “equivalent” fiber lengths: by simply varying the experimental input power, they provide a highly spatially resolved longitudinal evolution of the initial wave form while avoiding the intrinsic drawbacks commonly experienced in fiber optics when a standard cutback technique is used.
As reported in Ref. [2], the authors carefully studied the dynamics arising from three of the most commonly encountered FWM schemes in nonlinear optics (for instance, symmetric and asymmetric dual-wave as well as three-wave pumped FWM). In all these cases, the experimental results revealed FWM-induced wave breaking and the subsequent formation of dispersive shock waves [Fig. 1(c)], showing excellent agreement with the numerical predictions. Depending on the initial type of FWM scheme considered, the authors pointed out several wave-breaking evolution scenarios, including the formation of so-called black and gray solitons (gray solitons are solitary waves that propagate as amplitude depressions, as opposed to regular solitons that are positive wave packets—note that black solitons are a limiting case of gray solitons in which the depression amplitude goes to zero) [9]. In any case, because of the quasiperiodic nature of the input signal, the authors managed to show that the optical undular bores generated within the initial modulation period would propagate outward and thus collide with adjacently generated bores, finally leading to more complex dynamics.
It is probable that the richness of these experimental results (also supported by detailed numerical studies) will encourage future investigations. Indeed, the fundamental aspects of the hydrodynamic-optic analogy have received increasing attention over the last several years and are still expected to provide both exciting results and stimulating discussions. Moreover, given the widespread applications relying on nonlinear processes in numerous platforms (such as fiber, bulk, or integrated devices), one can reasonably expect that these results will provide further insight into the understanding of FWM and the associated practical implementations, including numerous parametric amplifications architectures, ultrafast pulse trains generation, frequency comb generation, as well as optical signal regeneration.
References
- G. B. Whitham, Linear and Nonlinear Waves (Wiley, New York, 1974)[Amazon][WorldCat]
- J. Fatome, C. Finot, G. Millot, A. Armaroli, and S. Trillo, “Observation of Optical Undular Bores in Multiple Four-Wave Mixing,” Phys. Rev. X 4, 021022 (2014)
- T. B. Benjamin and M. J. Lighthill, “On Cnoidal Waves and Bores,” Proc. R. Soc. London A 224, 448 (1954)
- Y. Kodama and S. Wabnitz, “Analytical Theory of Guiding Center Nonreturn-to-Zero and Return-to-Zero Signal Transmission in Normally Dispersive Nonlinear Optical Fibers,” Opt. Lett. 20, 2291 (1995)
- “Discussion & Debate: Rogue Waves - Towards a Unifying Concept?,” edited by N. Akhmediev and E. Pelinovsky, Eur. Phys. J. Spec. Top. 185 (2010)
- B. Kibler, J. Fatome, C. Finot, G. Millot, F. Dias, G. Genty, N. Akhmediev, and J. M. Dudley, “The Peregrine Soliton in Nonlinear Fibre Optics,” Nature Phys. 6, 790 (2010); B. Kibler, J. Fatome, C. Finot, G. Millot, G. Genty, B. Wetzel, N. Akhmediev, F. Dias, and J. M. Dudley, “Observation of Kuznetsov-Ma Soliton Dynamics in Optical Fibre,” Sci. Rep. 2, 463 (2012)
- A. Chabchoub, N. P. Hoffmann, and N. Akhmediev, “Rogue Wave Observation in a Water Wave Tank,” Phys. Rev. Lett. 106, 204502 (2011); A. Chabchoub, N. Hoffmann, M. Onorato, G. Genty, J. M. Dudley, and N. Akhmediev, “Hydrodynamic Supercontinuum,” 111, 054104 (2013)
- D. J. Moss, R. Morandotti, A. L. Gaeta, and M. Lipson, “New CMOS-Compatible Platforms Based on Silicon Nitride and Hydex for Nonlinear Optics,” Nature Photon. 7, 597 (2013)
- Y. S. Kivshar and B. Luther-Davies, “Dark Optical Solitons: Physics and Applications,” Phys. Rep. 298, 81 (1998)