Finding some sense in disorder
It is sometimes remarked that quantum mechanics underlies much of the important technology that we use in our day to day lives. This is certainly true of semiconductors, which are the basis of modern computers and whose properties depend on the quantum behavior of electrons in crystal lattices. Yet the implications of quantum behavior in “real” systems are not always obvious, as Philip Anderson found when he discovered that electrons, which should exist as spatially extended states in perfect crystals, eventually localize as the amount of disorder in the crystal increases [1]. Because it is fundamental to our understanding of electrical conductivity, this phenomenon, known as the Anderson transition, or alternatively, the metal-insulator transition, has remained a central topic of research. Experimentally, however, it has proven very difficult to create the right conditions to observe the transition [2]. Now Julien Chabé and colleagues at the Université des Sciences et Technologies de Lille and the Université Pierre et Marie Curie in Paris have demonstrated an equivalent of the Anderson transition for electrons by trapping cold atoms in a periodic, optical potential [3]. Their experiment, reported in Physical Review Letters overcomes previous difficulties associated with observing the transition in cold atom gasses and also holds the promise of extending the study of the Anderson transition to higher dimensions.
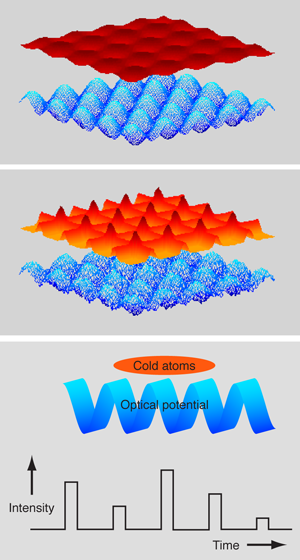
Metals and semiconductors are idealized as perfect crystal lattices: three-dimensional arrays of atoms that create a periodic potential for electrons. In such an array, the electrons take the form of Bloch waves that have the same periodicity as the lattice and are extended throughout the volume of the solid (see Fig. 1, top). However, this image of perfect periodicity turns out to be inadequate to describe real crystals, which contain imperfections. These defects can be lattice sites where an atom is missing or impurities that break the lattice symmetry. An important example of such a system is that of a doped semiconductor [4]. Naively, one would expect disorder in the lattice to give rise to dephasing of the Bloch waves and the break down of the electrons’ quantum wave properties at very high levels of disorder. However, one of the intriguing aspects of Anderson localization is that it is due to coherent quantum behavior in which scattering of extended electron wave functions gives destructive interference outside a certain range of the wave-packet center, leading to a characteristic exponential line shape of the particle wave function (see Fig. 1, middle).
According to this picture, Anderson localization is an interference phenomenon that can, in principle, occur in any wave-mechanical system [5]. A temporal equivalent of Anderson localization known as dynamical localization was observed in cold atoms some time ago [6] but spatial Anderson localization was found for the first time only very recently, and then only within one-dimensional systems [7]. Returning to the temporal domain, Chabé et al. have now observed the Anderson transition itself using a matter wave technique, and they have done it for an effective three-dimensional system—the case most relevant to our understanding of real materials.
In order to probe the Anderson transition, Chabé et al. made use of a well known mapping between the Anderson model and a system known as the kicked rotor. The kicked rotor is literally a free rotating mass on a pivot that receives periodic momentum “kicks” and has long been a workhorse in theoretical studies of dynamical chaos. Mark Raizen at the University of Texas first realized the kicked rotor in a quantum system in 1995 by placing laser-cooled atoms in an optical standing wave [6]. The standing wave was tuned to near the resonant frequency of the atoms and pulsed on and off, corresponding to the kicks in the original rotor theory. This experiment proved to be a very successful new way to investigate quantum chaos—the study of quantum analogs of classically chaotic systems.
In 1984, Grempel, Prange, and Fishman showed that the quantum kicked rotor problem can be mapped onto Anderson’s model for particles in a disordered potential [8]. Casati, Guarneri, and Shepelyansky (CGS) went further by demonstrating that adding extra modulation frequencies to the potential of the quantum kicked rotor produced a system that was effectively three dimensional (3D). In fact, they showed that the quantum kicked rotor with three incommensurate frequencies modulating the potential could be mapped onto the 3D Anderson model for particles in a disordered lattice. They also demonstrated numerically that a transition from extended to localized states occurred in this system with increasing modulation strength [9]. From here the stage was set for a demonstration of the 3D Anderson transition with cold atoms. Although the experiment considers temporal or dynamical localization, rather than the spatial localization originally envisaged by Anderson, the distinction is formally unimportant.
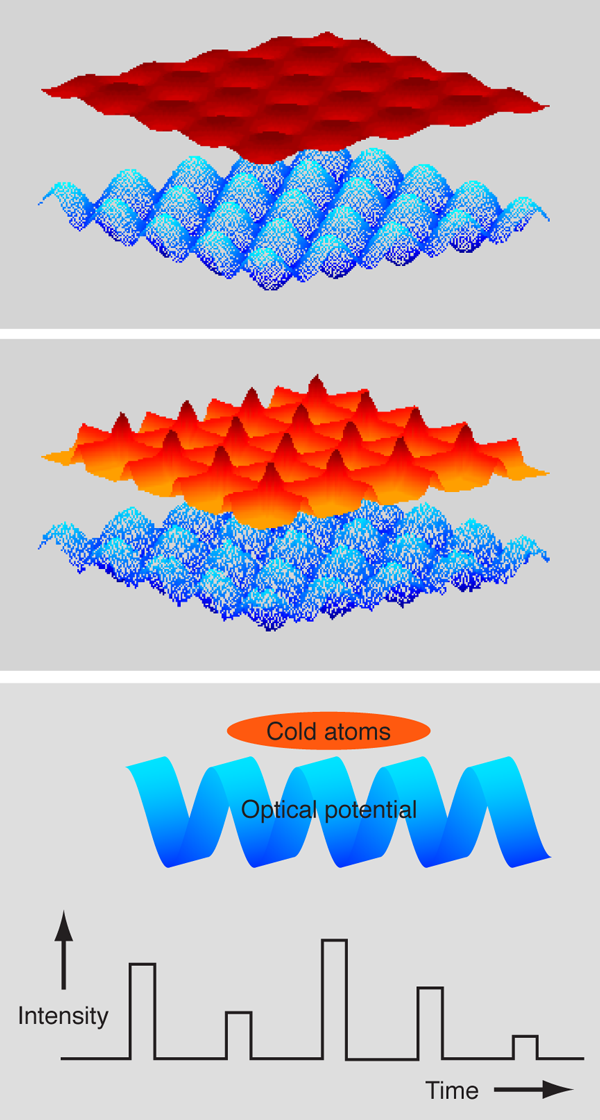
The experimental setup used by Chabé et al. to probe the Anderson transition consists of a magneto-optical trap, in which laser-cooling reduces the temperature of cesium atoms to a temperature of about 3 K. The atoms are then exposed to pulses from a one-dimensional periodic potential, or lattice, created by two overlapping laser beams that form a standing wave. The strength of the pulses is modulated according to a periodic function with three incommensurate frequencies, thus realizing the CGS model (Fig. 1, bottom). In the experiment, both the depth of modulation and the average potential seen by the atoms (proportional to the laser strength) control the effective disorder of the system. The experimenters had to carefully vary both quantities in order to observe the transition.
The physically observed quantity in the experiment is the population of atoms that have zero momentum after the optical pulses have been applied. This quantity is inversely proportional to how far the atoms have spread out along the lattice and thus provides information about the extent of the atomic wave functions, allowing the transition between extended and localized states to be measured.
The fact that it has taken almost two decades from the creation of the CGS model to its realization in an experiment is due to some thorny technical difficulties. Numerical studies indicate that there should be a sharp transition from the extended Bloch waves to localized states at a critical level of disorder. According to the CGS model, to observe this transition the localized wave functions must be detected on a time scale that may be long compared with the typical experimental running time. This is because a long spatial localization length maps to a long localization time in the CGS model.
Chabé et al.’s insight was to analyze their experimental results with the same techniques developed to overcome these problems in numerical simulations. In this way, they were able to identify the sharp crossover between the localized and delocalized states of a cold atom gas. In particular, Chabé et al. applied techniques from a field known as scaling theory [10,11], which describes how the conductivity of a system with disorder changes as the size of the system is scaled. Specifically, a technique called finite size scaling [12] was used to infer the long time behavior of the atoms’ wave functions from the short time data collected in the experiment.
One of the most exciting aspects of this experiment is the immediate possibility of extending it to higher dimensions. The localization phenomenon is sensitive to the dimensionality of the disordered system. In fact, all states can be proven to be localized in a one-dimensional system and, for two dimensions, given a large enough system, states also seem to assume a localized form. The true Anderson transition is, therefore, first found in 3D disordered systems. In higher dimensional systems, numerical studies become difficult and the expected nature of the dynamics is still controversial. Anderson localization is expected to appear in higher dimensions but there are some dissenting voices regarding what the transition would look like. It is tantalizing to note that the relatively simple addition of an extra modulating frequency to Chabé et al.’s existing setup could begin to probe such unknown regimes.
Acknowledgments
The author would like to thank Tomi Ohtsuki for discussions about the Anderson transition.
References
- P. W. Anderson, Phys. Rev. 109, 1492 (1958)
- P. A. Lee and T. V. Ramakrishnan, Rev. Mod. Phys. 57, 287 (1985)
- J. Chabé, G. Lemarié, B. Grémaud, D. Delande, P. Szriftgiser, and J. C. Garreau, Phys. Rev. Lett. 101, 255702 (2008)
- N. Mott, M. Pepper, S. Pollitt, R.H. Wallis, C. J. Adkins, Proc. R. Soc. Lond. A 345 169 (1975)
- T. Schwartz, G. Bartal, S. Fishman, and M. Segev, Nature 446, 52 (2007)
- F. L. Moore et al., Phys. Rev. Lett. 75, 4598 (1995)
- J. Billy et al., Nature 453, 891 (2008); G. Roati et al., 453, 895 (2008)
- D. R. Grempel, R. E. Prange, and S. Fishman, Phys. Rev. A 29, 1639 (1984)
- G. Casati, I. Guarneri, and D. L. Shepelyansky, Phys. Rev. Lett. 62, 345 (1989)
- J. T. Edwards and D. J. Thouless, J. Phys. C 5 807 (1972)
- K. Slevin and T. Ohtsuki, Phys. Rev. Lett. 82, 382 (1999)
- M. E. Fisher and M. N. Barber, Phys. Rev. Lett. 28, 1516 (1972)