Topological Insulators Turn a Corner
Identifying new phases of matter that have unusual properties is a key goal of condensed-matter physics. A famous recent example is the theoretical prediction of crystalline materials known as topological insulators (TIs), several of which have now been identified in the laboratory [1]. TIs are electronic insulators in their -dimensional interior (bulk) but allow metallic conduction on their ( )-dimensional boundaries. This is because in their bulk these materials have an energy gap between the ground and first excited states of electrons, but at their boundaries electrons can move, and hence conduct charge, without paying an energy penalty. Such “gapless” boundary states are unusually robust to the detrimental effects of impurities, and they are responsible for exotic properties that emerge when TIs are coupled to magnets or superconductors. For instance, they endow superconducting vortices with “non-Abelian” quantum statistics that could make the vortices a robust platform for quantum computing.
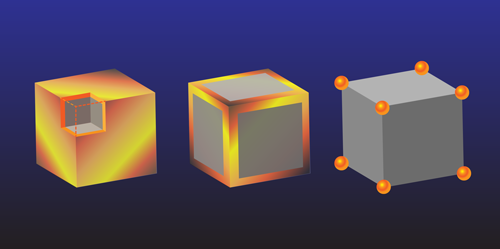
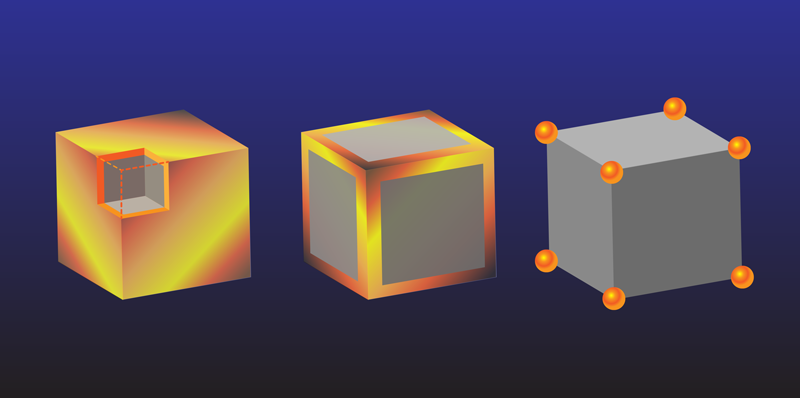
Now, four teams of researchers [2–6] have identified a new class of TIs in dimensions . These “higher-order” [6] TIs have ( )-dimensional boundaries that, unlike those of conventional TIs, do not conduct via gapless states but instead are themselves TIs. An th order TI instead has gapless states that live on ( )-dimensional subsystems. For instance, in three dimensions, a second-order TI has gapless states located on 1D "hinges" between distinct surfaces, whereas a third-order TI has gapless states on its 0D corners (Fig. 1). Similarly, a second-order TI in two dimensions also has gapless corner states. Such higher-order systems constitute a distinctive new family of topological phases of matter.
There are several features that one would desire in a theoretical construction of a new topological phase. First, the phase should be realized in simple models. Second, the symmetries necessary to protect conduction via the phase’s boundary states, and thereby its topological properties, should be identified. Third, the phase should admit an effective description that is insensitive to microscopic details—for TIs, such a description is usually a field theory that captures a quantized response of the material to external fields. Finally, its gapless boundary states shouldn’t come from run-of-the-mill surface physics, but rather from a topologically nontrivial bulk. Naively, however, it seems as though one could simply circumvent all of these challenges and build, for example, second-order TIs by “decorating” edges or surfaces of conventional (topologically trivial) 2D or 3D insulators with 1D or 2D TIs. However, this does not work in general; for example, if each edge of a finite 2D system is decorated with a 1D TI, each of the system’s four corners will then host a pair of gapless states, one from each edge. Yet in many cases, a single state that is gapless in isolation is destroyed when it encounters another. So in our example, the corners will generically have a gap to electronic excitations.
This failed attempt carries two lessons that succinctly capture the challenge of constructing higher-order TIs: first, not all lower-dimensional TIs are automatically good building blocks with which to construct higher-order TIs, and second, additional symmetries may be necessary to stabilize gapless boundary states. The four research groups take distinct approaches to this problem; when combined, the approaches have all the desirable features outlined above.
Wladimir Benalcazar at the University of Illinois at Urbana-Champaign and colleagues [2, 3] build on the observation that several distinct known topological quantization phenomena can be unified in terms of a generalized bulk dipole moment in dimensions that is quantized. Examples of such phenomena are the charge polarization in crystals [7], the Hall conductance in two-dimensional Chern insulators with broken time-reversal symmetry [8], and the magnetoelectric polarizability in 3D TIs [9]. These phenomena are also linked to the existence of ( )-dimensional gapless boundary states. Benalcazar and colleagues [2, 3] ask the natural question of whether this picture of a quantized dipole generalizes to quantized quadrupole and octupole moments, and they answer it in the affirmative using an effective-field theory. This allows the researchers to identify phases whose hinges and corners have gapless degrees of freedom. Crucially, they show that these moments are robustly quantized only in the presence of various crystalline symmetries and that the gapless states at hinges and corners cannot emerge in isolation—their existence is inextricably tied to that of a bulk topological phase. The quantized moments are, in turn, related, like charge polarization [7], to topological properties of an electron’s wave function, the most familiar of which is known as Berry’s phase. This quantity and its relatives are topological invariants—meaning that they are insensitive to “smooth” changes in material properties—and describe how the electronic wave functions behave as their momenta are varied over the “Brillouin zone,” the periodic set of momentum values allowed inside a crystal.
Both Benalcazar and colleagues [2, 3] and Zhida Song from the Chinese Academy of Sciences in Beijing and collaborators [4] leverage an alternative description of TIs that allows the researchers to deduce the material’s properties from the structure of their “Wannier orbitals.” These real-space objects resemble the orbitals in atoms and molecules, and like the momentum-space wave functions, they are also sensitive to Berry phases. Song and co-workers, in particular, use this approach to propose a simple example of a 2D “corner TI”—a 2D TI with gapless modes on its corners but not its edges—whose Wannier orbitals are centered at high-symmetry points that are distinct from the material’s atomic lattice sites. Although Wannier orbitals are normally defined using periodic boundary conditions, their topological properties may be reflected in the behavior of samples with physical boundaries. In the corner TI example, when a finite sheet of the material preserves the symmetry of 90° rotations about such high-symmetry points (for example, if the sample has a square shape), then, intuitively, each of the four corners linked by such rotations has a quarter of an electronic Wannier orbital of the periodic system, giving rise to gapless states. This intuitive picture can be made rigorous by analyzing properties of the Wannier orbitals [2, 3].
Josias Langbehn and others at the Free University of Berlin [5] adopt a slightly different route, similar in spirit to the thought experiment sketched earlier in this article. They build second-order TIs by developing an algorithm for “gluing” ( )-dimensional TIs to ( )-dimensional boundaries of -dimensional conventional insulators in a manner that preserves gapless corner or hinge states. Building on a recent classification [10] of reflection-symmetric TIs, they elucidate how second-order TIs fit into the existing topological classification of insulators and show that various crystalline-symmetry requirements could be relaxed while preserving the surface conduction. This is an important step in widening the experimental relevance of higher-order TIs because such symmetries can be very difficult to realize as exact symmetries of a material.
Finally, Frank Schindler at the University of Zurich and colleagues [6] use a mix of these techniques to study two different types of second-order TIs in 3D: ones with “chiral” hinge states, in which electrons flow in only one direction, and “helical” ones, in which the electrons propagate in both directions but with their spin locked to their direction of motion. These behaviors parallel those of electrons in the 1D edge states of the 2D integer quantum Hall phase and in 2D TIs, respectively. Excitingly, Schindler and colleagues suggest candidate solid-state materials that could host these new phases. These candidate materials belong to classes of materials that are known to be fertile sources of TI physics, and they can be readily studied with a wealth of existing experimental techniques.
We have not discussed various other elegant results from these new studies, such as the classification of higher-order TIs [5, 6] and the derivation of simple formulae for determining the topological invariants that distinguish them from conventional insulators [4]; we direct the reader to the original articles for details. The work suggests several exciting new directions; for instance, it will be interesting to generalize these ideas from the current setting of noninteracting fermionic systems to interacting systems of fermions or bosons (Song and colleagues have already taken first steps towards this [4]). On the experimental side, there are now both candidate solid-state materials [6], as mentioned earlier, and specific proposals to engineer higher-order TIs in cold atomic gases and photonic systems [2, 3], along with realistic protocols for detecting corner and hinge states [2, 3, 5, 6]. While, just as in ordinary TIs, the protected conduction and other unusual properties of these new TIs might find applications, these new discoveries are really of a fundamental, rather than applied, nature. All in all, these works remind us that there may yet be surprises in store as we delve deeper into the study of topological matter.
This research is published in Science, Physical Review Letters, and Physical Review B and posted on the arXiv.
References
- Excellent reviews of the prediction and discovery of TIs may be found in M. Z. Hasan and C. L. Kane, “Colloquium: Topological Insulators,” Rev. Mod. Phys. 82, 3045 (2010); J. E. Moore, “The Birth of Topological Insulators,” Nature 464, 194 (2010).
- W. A. Benalcazar, B. A. Bernevig, and T. L. Hughes, “Quantized Electric Multipole Insulators,” Science 357, 61 (2017).
- W. A. Benalcazar, B. A. Bernevig, and T. L. Hughes, “Electric Multipole Moments, Topological Multipole Moment Pumping, and Chiral Hinge States in Crystalline Insulators,” Phys. Rev. B. 96, 245115 (2017).
- Z. Song, Z. Fang, and C. Fang, “()-dimensional edge states of rotation symmetry protected topological states,” Phys. Rev. Lett. 119, 246402 (2017).
- J. Langbehn, Y. Peng, L. Trifunovic, F. von Oppen, and P. W. Brouwer, “Reflection-symmetric second-order topological insulators and superconductors,” Phys. Rev. Lett. 119, 246401 (2017).
- F. Schindler, A. M. Cook, M. G. Vergniory, Z. Wang, S. S. P. Parkin, B. A. Bernevig, and T. Neupert, “Higher-Order Topological Insulators,” arXiv:1708.03636.
- For a review, see R. Resta and D. Vanderbilt, “Theory of Polarization: A Modern Approach,” in Physics of Ferroelectrics: A Modern Perspective, edited by K. Rabe, Ch. H. Ahn, and J.-M. Triscone (Springer, Berlin, 2007), p. 3-68[Amazon][WorldCat].
- D. J. Thouless, M. Kohmoto, M. P. Nightingale, and M. den Nijs, “Quantized Hall Conductance in a Two-Dimensional Periodic Potential,” Phys. Rev. Lett. 49, 405 (1982); F. D. M. Haldane, “Model for a Quantum Hall Effect without Landau Levels: Condensed-Matter Realization of the ‘Parity Anomaly’,” 61, 2015 (1988).
- X.-L. Qi, T. L. Hughes, and S.-C. Zhang, “Topological Field Theory of Time-Reversal Invariant Insulators,” Phys. Rev. B 78, 195424 (2008); A. M. Essin, J. E. Moore, and D. Vanderbilt, “Magnetoelectric Polarizability and Axion Electrodynamics in Crystalline Insulators,” Phys. Rev. Lett. 102, 146805 (2009); T. L. Hughes, E. Prodan, and B. A. Bernevig, “Inversion-Symmetric Topological Insulators,” Phys. Rev. B 83, 245132 (2011); A. M. Turner, Y. Zhang, R. S. K. Mong, and A. Vishwanath, “Quantized Response and Topology of Magnetic Insulators with Inversion Symmetry,” 85, 165120 (2012).
- C.-K. Chiu, H. Yao, and S. Ryu, “Classification of Topological Insulators and Superconductors in the Presence of Reflection Symmetry,” Phys. Rev. B 88, 075142 (2013); T. Morimoto and A. Furusaki, “Topological Classification with Additional Symmetries from Clifford Algebras,” 88, 125129 (2013); K. Shiozaki and M. Sato, “Topology of Crystalline Insulators and Superconductors,” 90, 165114 (2014); L. Trifunovic and P. W. Brouwer, “Bott Periodicity for the Topological Classification of Gapped States of Matter with Reflection Symmetry,” arXiv:1707.06306.