Acoustic Crystals with a Möbius Twist
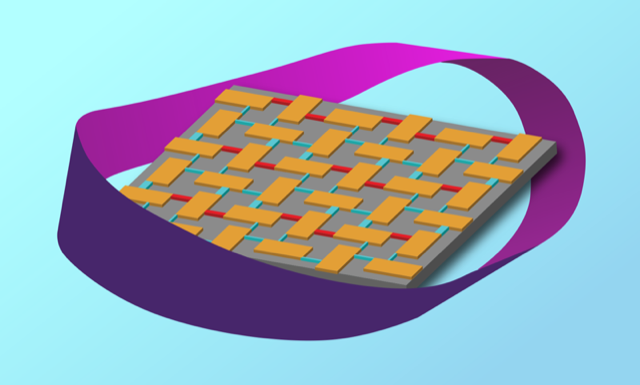
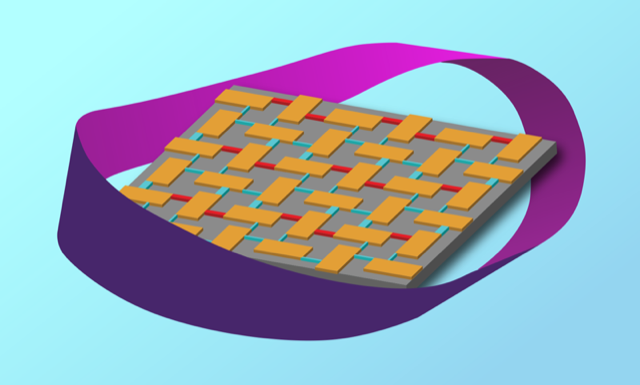
The Möbius loop is an iconic geometry that shows the oddity of a topological transformation. One can easily construct this one-sided surface by twisting a strip of paper by 180° and sticking the ends of the strip together (Fig. 1). As topology has turned into a central topic in condensed-matter physics, an interesting question arises: Can a Möbius-like geometry interact with topological states and enrich them? Seeking an answer to that question, two groups of researchers from Nanyang Technological University, Singapore, and Wuhan University, China, respectively, have coupled a Möbius loop with the topological edge states of acoustic lattices—periodic arrays of acoustic resonators [1, 2]. The experimental observations indicating that this system hosts a new class of “Möbius-twisted” topological bands will inspire new fundamental research directions in topology and may lead to new devices for controlling acoustic or electromagnetic waves.
The topological phases in a topological insulator are closely tied to the system’s spatial symmetries. Studies of topological crystalline insulators have focused chiefly on point-group symmetries, in which the set of operations that leave the crystal structure unchanged has a fixed point. Other types of symmetries, however, remain largely unexplored, including those that involve translational symmetry under a displacement that is nonprimitive; that is, it doesn’t correspond to a multiple of a lattice vector. In 2015, a theoretical model for a nonsymmorphic symmetry—a symmetry about a glide plane or screw axis, which cannot be described as a combination of translations and rotations—revealed a new route toward a Z2 topological phase that does not involve antiunitary symmetries [3]. It was predicted that this new topological phase supports stable and gapless topological edge states with a Möbius twist in the band structures, leading to systems with such a topological phase being dubbed “Möbius insulators.”
Möbius insulators have bands with a 4𝜋 periodicity in the projective translational symmetry direction—meaning that, like a finger tracing the surface of a Möbius strip, a particle must complete two circuits of the system before it returns to its initial location and phase. While researchers have been looking for Möbius insulators in various quantum systems [4–6], topological acoustic lattices offer an alternative experimental path for finding these exotic systems as the Nanyang and Wuhan groups show.
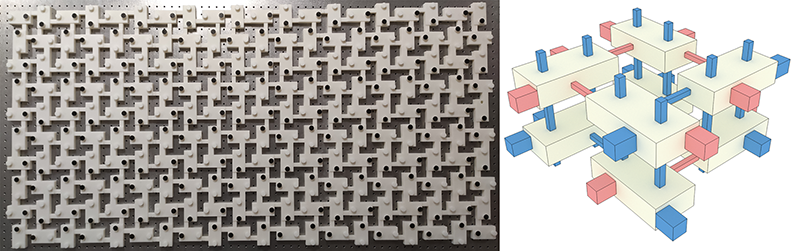
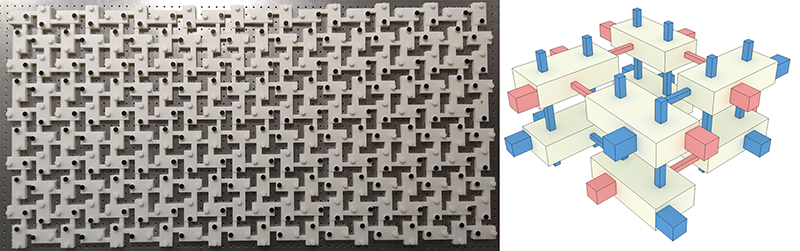
Acoustic lattices have become fertile ground for investigating topological physics owing to the flexibility with which the hopping of acoustic waves between lattice sites can be manipulated. In acoustic lattices, this hopping, which corresponds to the hopping of electrons between lattice sites in electronic topological materials, is engineered using coupling tubes. Resonating cavities, meanwhile, provide the counterparts of the on-site orbitals in electronic topological materials. Using these components, researchers can not only engineer geometrical symmetries but also control the sign of the lattice hopping.
These degrees of freedom have recently been used to demonstrate acoustic quadrupole topological insulators [7, 8]. Acoustic quadrupole insulators provide some essential features of Möbius insulators—such as the 𝜋 gauge flux, which describes how a particle hopping around a group of four neighboring lattice sites has its phase changed after one circuit. However, the challenging realization of a lattice with the nonsymmorphic symmetries that produce the Möbius topology has not yet been achieved. The Nanyang and Wuhan groups have found an alternative way to create this topology by replacing nonsymmorphic symmetries with a more easily realizable projective symmetry (so called because it is created by a projection of the primitive translation symmetry of the lattice via the gauge transformation process).
The researchers from Nanyang Technological University constructed a 2D acoustic lattice by combining cuboid acoustic resonators with coupling tubes, allowing the control of the sign of the hopping terms (Fig. 2) [1]. The 2D translational symmetry in such a lattice creates a fourfold degenerate Dirac point in the band structure. Interestingly, the behavior of the band structure varies depending on how the symmetry is broken. The acoustic lattice shows topological edge-state bands featuring a Möbius twist when the translational symmetry is broken in the x direction, while the one in the y direction is preserved. The researchers observed these twisted gapless edge states, which are detached from the bulk bands, by measuring the acoustic pressure distributions on the x and y edges and determining the edge-state dispersion. If the translation symmetries in both the x and y directions are broken, the acoustic lattice shows a graphene-like semimetal phase. In this case, a flat edge band exists on the edge parallel to the x direction, and this band connects the two Fermi points. The matching of the measured edge dispersion with theoretical predictions demonstrates the direct connection between the new topological phase and projective symmetries.
The researchers from Wuhan University instead introduced a 3D version of an acoustic lattice consisting of multiple layers of acoustic resonators and coupling tubes (Fig. 2) [2]. They observed not only Möbius twisted edge states but also high-order topological states with energy confined at the hinges, which demonstrate that the projective symmetry can endow 3D systems with unique features. Given the extra dimension, 3D acoustic Möbius insulators show the capability of engineering the topological order of acoustic lattices, as well as the ability to route acoustic energy in the z direction.
The construction of acoustic Möbius insulators shows the potential of the acoustic lattice as a robust platform for pushing the frontiers of topology exploration in condensed-matter systems. It would be exciting to see whether the use of projective symmetry can be expanded to bring new physics to a large number of topological systems; projective inversion symmetry, for example, can be harnessed to achieve, in a spinless system, topological phases that would otherwise be unique to spinful systems and vice versa [9]. The generalization of these works will also benefit research in other systems carrying classical waves, such as electromagnetic and elastic waves, in which gauge fields can be readily realized. Finally, the observation of these new topological acoustic states may inspire new devices that feature extraordinary acoustic wave behaviors, such as the waveguiding of acoustic energy and the control of acoustic noise.
References
- H. Xue et al., “Projectively enriched symmetry and topology in acoustic crystals,” Phys. Rev. Lett. 128, 116802 (2022).
- T. Li et al., “Acoustic Möbius insulators from projective symmetry,” Phys. Rev. Lett. 128, 116803 (2022).
- K. Shiozaki et al., “Z2 topology in nonsymmorphic crystalline insulators: Möbius twist in surface states,” Phys. Rev. B 91, 155120 (2015).
- Y. X. Zhao and A. P. Schnyder, “Nonsymmorphic symmetry-required band crossings in topological semimetals,” Phys. Rev. B 94, 195109 (2016).
- P. Y. Chang et al., “Möbius Kondo insulators,” Nat. Phys. 13, 794 (2017).
- R. X. Zhang et al., “Möbius insulator and higher-order topology in MnBi2nTe3n+1,” Phys. Rev. Lett. 124, 136407 (2020).
- M. Serra-Garcia et al., “Observation of a phononic quadrupole topological insulator,” Nature 555, 342 (2018).
- Y. Qi et al., “Acoustic realization of quadrupole topological insulators,” Phys. Rev. Lett. 124, 206601 (2020).
- Y. X. Zhao et al., “Switching spinless and spinful topological phases with projective PT symmetry,” Phys. Rev. Lett. 126, 196402 (2021).