Looking for Hofstadter’s Butterfly in Cold Atoms

Cheng Chin; Erich J. Mueller

Viewpoint

Looking for Hofstadter’s Butterfly in Cold Atoms

Cheng Chin

James Franck Institute, Enrico Fermi Institute, and Department of Physics, University of Chicago, Chicago, IL 60637, USA

Erich J. Mueller

Laboratory of Atomic and Solid State Physics, Cornell University, Ithaca, NY 14853, USA

October 28, 2013• Physics 6, 118

Two research groups have engineered an optical lattice of atoms that mimic the behavior of electrons moving in a crystal and a strong magnetic field.

Figure caption — **Figure 1:** Schematic of the experiments by Aidelsburger *et al.* [1] and by Miyake *et al.* [2]. Rubidium atoms hop between sites in a two-dimensional square lattice in a regime where tunneling requires absorbing and emitting photons produced by lasers. Interaction with these lasers produces path-dependent phases for the atoms, mimicking the effect of a magnetic field on electrons. The phase accumulated when moving around a single unit cell, $ϕ$ , is of order $π / 2$ for these experiments.

In a sufficiently strong magnetic field, charged particles in a periodic potential (such as a crystal lattice) display novel physics: as a function of magnetic field, the electron excitations are predicted to form a stunning fractal structure, called the Hofstadter butterfly [1]. This effect has never been seen in a natural crystal, because of the sizable magnetic fields required, but has been observed in various artificial superlattices [2–6]. Now, two independent groups, one led by researchers at the University of Munich and the Max Planck Institute of Quantum Optics in Garching, Germany, and the other at the Massachusetts Institute of Technology (MIT), Cambridge, have used the interaction between cold atoms and lasers to create a system that should also exhibit Hofstadter excitations. As reported in Physical Review Letters [7,8], both groups placed rubidium atoms in an optical lattice and used external lasers to coax the atoms into circular motion, analogous to the motion of electrons in a magnetic field. Colder temperatures will be needed to see the fractal structure of the atom excitations, but because atomic systems are essentially free of defects, they may ultimately provide the cleanest view of Hofstadter’s butterfly. This system is also a platform for exploring more exotic physics involving the interplay of lattices, magnetic fields, and strong interactions.

A classical electron moving perpendicular to a uniform magnetic field $B$ undergoes cyclotron motion. The frequency of the electron’s circular orbit is $ω_{C} = e B / m$ ( $e$ is the electron’s charge and $m$ its mass) and doesn’t depend on the radius of the orbit or its location. In quantum mechanics, this symmetry gives rise to a large number of states with the same energy: in a two-dimensional sample of area $A$ the electron energies can only take quantized values, called Landau levels, and each level contains $N_{Landau} = m ω_{C} A / h$ states.

The Landau quantization can be compared to the physics of electrons in the periodic electric field of a crystal lattice (with no magnetic field). In this case, the electron energies divide up into distinct bands, with gaps between them. The Bloch bands can each accommodate $N_{Bloch}$ states, where $N_{Bloch}$ is typically the number of sites in the lattice.

An important milestone in condensed matter physics was answering two questions: what happens to the Bloch bands in the presence of a magnetic field, and what happens to the Landau levels in the presence of a lattice? In 1933, Rudolf Peierls began developing the basic formalism to answer these questions [9]; in 1955, Philip Harper reframed the questions in a simpler mathematical form [10]. But it wasn’t until 1976 that Douglas Hofstadter showed that, as a function of magnetic field, the Landau and Bloch bands fragment into a large number of smaller bands [1]. The number of states in each of these bands is given by the greatest common divisor of $N_{Landau}$ and $N_{Bloch}$ . Even a small change in the magnetic field can lead to a drastic change in the number of bands, and a plot of the allowed single particle energies versus magnetic field yields an intricate self-similar fractal with a shape reminiscent of a butterfly.

The fractal structure of the Hofstadter bands is an interesting mathematical object in its own right. But another property of these energy bands is that they cannot be continuously deformed into Bloch bands without closing the energy gaps between them [11]. Materials with this property will generically have quantum mechanical states that exist only on the edge of the sample. These edge states are particularly robust in this system: at particular filling factors (and sufficiently low temperature), they lead to quantized Hall conductance that is unaffected by disorder. Related physics is seen in topological insulators (see 23 July 2008 Viewpoint).

Physicists have searched for Hofstadter bands in two-dimensional electronic materials, but the magnetic fields available to typical solid-state experiments ( $100$ tesla or less) are sufficiently small that $N_{Landau} > > N_{Bloch}$ . In this limit, each Bloch band is simply divided into Landau levels, whose internal structure is obscured by even the smallest amount of disorder. Signatures of the Hofstadter bands can instead be seen in artificial superlattices [2–6].

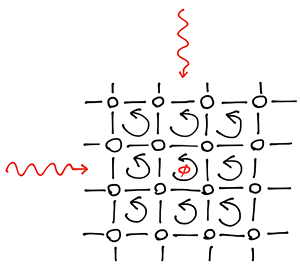
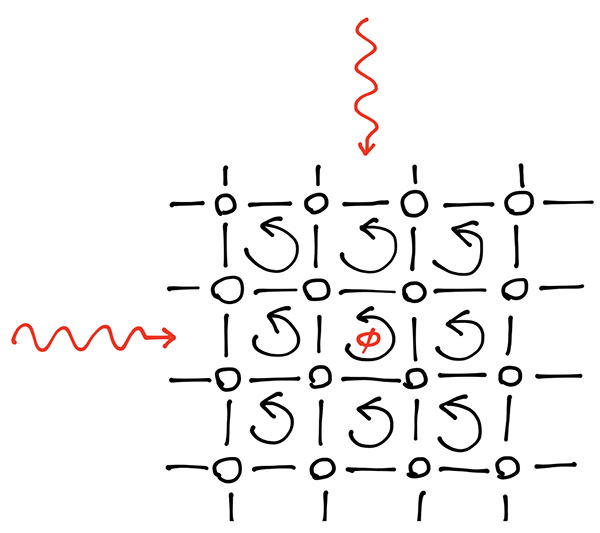
Monica Aidelsburger and colleagues at the University of Munich and the MPI [7] and Hirokazu Miyake and colleagues at MIT [8] have both designed lattices of cold atoms that act as analogs to these electronic systems, but with potentially fewer defects and the possibility to probe the Hofstadter-Harper model in new regimes. Both groups built upon existing techniques to produce the same effect on neutral atoms that a magnetic field has on electrons (See 12 December 2011 Viewpoint). When an electron completes a loop in a plane perpendicular to a magnetic field, it acquires a quantum-mechanical phase (the Aharanov-Bohm phase.) Similarly, Aidelsburger et al. and Miyake et al. prepared cold-atom systems in which the atoms (bosonic rubidium atoms) acquired a phase as they underwent circular motion. To do this, the two groups engineered a lattice in which the neutral atoms could only hop to the neighboring sites by absorbing and emitting photons. These photon-induced tunneling events introduced phase shifts (Fig. 1): when an atom moved in a closed loop, the phase shift was proportional to the area of the loop, and independent of where the atom was located. Atoms acquired a phase of order $π / 2$ when they circled a single unit cell; a similar phase shift in an electronic system would require a magnetic field of more than $1000$ tesla.

Neither group can directly measure the fractal excitations associated with the Hofstadter-Harper model, but they do provide compelling evidence that they have engineered this Hamiltonian. Miyake et al. studied the diffusion of the atoms in the lattice as they changed various system parameters to demonstrate that they had good control and understanding of the rates at which atoms tunneled from site to site. Aidelsburger et al. instead studied the motion of their bosons in isolated plaquettes (sets of four lattice sites that form a square). They tracked the circular motion of the atoms, analogous to the cyclotron motion of an electron in the presence of a uniform magnetic field. They were able to observe opposite cyclotron motion for atoms with different spin states. This counter-rotation shows that their experiment has time inversion symmetry, and can therefore be used as a platform for studying physics that relies on this symmetry, such as the spin Hall effect and topological insulators.

One way to look for the Hofstadter fractal energy spectrum in the future would be to load a degenerate Fermi gas (as opposed to the gas of bosons the groups have studied so far) in the same optical lattice: the fractal spectrum would be evident in the density profile of the atom cloud. Even with bosons, it would be possible to directly probe the band structure using techniques such as “band-mapping” (See 23 September 2011 Focus). Moreover, studying interacting bosons, as opposed to fermions, in Hofstadter bands could reveal unexpected effects. In either case, observing the Hofstadter butterfly directly, or its associated topological state, will require temperatures that are small compared to the gaps between Hofstadter bands. Reaching these temperatures may prove challenging given how much heat the lasers used in the experiments generate. We should emphasize, however, that reaching record low temperatures has been the routine work of cold atom experimentalists.

Acknowledgment

C.C. acknowledges support from NSF PHY-1206095 and ARO-MURI 63834. E.M. acknowledges support by the National Science Foundation under Grant No. PHY-1068165.

References

D. R. Hofstadter, “Energy Levels and Wave Functions of Bloch Electrons in Rational and Irrational Magnetic Fields,” Phys. Rev. B 14, 2239 (1976)
M. C. Geisler, J. H. Smet, V. Umansky, K. von Klitzing, B. Naundorf, R. Ketzmerick, and H. Schweizer, “Detection of a Landau Band-Coupling-Induced Rearrangement of the Hofstadter Butterfly,” Phys. Rev. Lett. 92, 256801 (2004)
S. Melinte et al., ”Laterally Modulated 2D Electron System in the Extreme Quantum Limit,” Phys. Rev. Lett. 92, 036802 (2004)
T. Feil, K. Výborný, L. Smrčka, C. Gerl, and W. Wegscheider, “Vanishing Cyclotron Gaps in a Two-Dimensional Electron System with a Strong Short-Period Modulation,” Phys. Rev. B 75, 075303 (2007)
C. R. Dean et al. "Hofstadter’s Butterfly and the Fractal Quantum Hall Effect in Moire Superlattices,” Nature 497, 598 (2013)
L. A. Ponomarenko et al., “Cloning of Dirac Fermions in Graphene Superlattices,” Nature 497, 594 (2013)
M. Aidelsburger, M. Atala, M. Lohse, J. T. Barreiro, B. Paredes, and I. Bloch, “Realization of the Hofstadter Hamiltonian with Ultracold Atoms in Optical Lattices,” Phys. Rev. Lett. 111, 185301 (2013)
H. Miyake, G. A. Siviloglou, C. J. Kennedy, W. C. Burton, and W. Ketterle, “Realizing the Harper Hamiltonian with Laser-Assisted Tunneling in Optical Lattices,” Phys. Rev. Lett. 111, 185302 (2013)
R. E. Peierls, “On the Theory of Diamagnetism of Conduction Electrons,” Z. Phys. 80, 763 (1933)
P. G. Harper, “Single Band Motion of Conduction Electrons in a Uniform Magnetic Field,” Proc. Phys. Soc. A 68, 874 (1955)
D. J. Thouless, M. Kohmoto, M. P. Nightingale, and M. den Nijs, “Quantized Hall Conductance in a Two-Dimensional Periodic Potential,” Phys. Rev. Lett. 49, 405 (1982)

About the Authors

Cheng Chin is a professor at the James Franck Institute, Enrico Fermi Institute, and the Department of Physics at the University of Chicago http://ultracold.uchicago.edu/. He received his Ph.D. in 2001 from Stanford University and completed his postdoc research at Innsbruck University. He conducts experimental research on ultracold gases. He has received several awards, including the 2011 I. I. Rabi prize from the American Physical Society.

Erich Mueller is an associate professor in the Laboratory of Atomic and Solid State Physics at Cornell University http://people.ccmr.cornell.edu/~emueller/. He received his Ph.D. in 2001 from the University of Illinois at Urbana-Champaign, and completed a postdoctoral fellowship at the Ohio State University. He conducts theoretical research on ultracold gases. In 2009 he was recognized as an Outstanding Referee by the American Physical Society.